Application of CL multi-wavelet transform and DCT in Information Hiding Algorithm

Published Online February 2011 in MECS

How to make the Information Hiding scheme have a better performance in terms of invisibility, robustness, sensitivity and anti-steganalysis becomes a research hotspot. Currently, the algorithm has not achieved four performances at the same time. The scheme based on space domain are good at invisibility, but can not meet the requirements of robustness and anti-steganalysis at the same time [1][2]. The scheme based on transform domain are good at robustness. Zhang et al [3] and Zhu et al [4] proposed Information Hiding scheme and digital watermarking scheme respectively based on discrete cosine transform (DCT) and discrete wavelet transform (DWT). They are robust enough against compression, but they are costly and low robust against cutting. and However, most algorithm are not strong in anti-steganalysis, and does not increase significantly [5][6].

Combination of different technologies can bring new ideas into Information Hiding. Taking advantage of the

CL multi-wavelet transforms and Discrete Cosine Transform in application of information processing, propose an Information Hiding scheme based on CL multi-wavelet transform and Discrete Cosine Transform. In particular, CL multi-wavelet transform can process multi-transformation at the same time, and satisfy compact sup-port and symmetry of the image processing. This new scheme is named as CL-DCT. Experiment results illustrate that CL-DCT is better than traditional algorithms as DWT-DCT and DCT-LSB in invisibility and robustness against image attacks such as JPEG2000, cutting, filtering and noise. Has certain ability against steganalysis such as Higher Order Statistics based on wavelet coefficients. Moreover, the CL-DCT has excellent sensitivity and against cutting to image attacks.

• II.    Information Hiding Algorithm design

A. Embedding region

In digital image processing based on the multi-wavelet transform, the energy distribution due to the order of decomposition and the direction of components. The energy distribution of CL multi-wavelet transform [7] provides a flexible information hiding strategy. Fig. 1 shows CL multi-wavelet first-order transformation to Lena image.

(a) Lena Normal

Figure 1. First-order CL multi-wavelet transform

(b) Lena CL first-order

Energy ratio of four First-order CL Multi-wavelet Transform sub-images is approximately as TABAL I [8].

TABLE I.

E nergy distribution of first - order CL multi - wavelet

CL transform LL 1 sub-image energy percentage of total image energy	Each component energy distribution of LL 1 sub-image (%)
	LL 2	LH 2	HL 2	HH 2
97.36	96.53	2.51	0.62	0.34

After CL multi-wavelet transform, the most energy not only concentrated in the first-order sub-image ( LL 1 ), but also concentrated in the first component of first-order sub-image ( LL 2 ).Based on the energy distribution of CL, Generating CL-DCT embedding region have four steps:

Step1. Transform the cover image with first-order CL multi-wavelet to obtain four sub-images. Embed robust parameters in LL 2 . Embed hiding Information in LH 2 and HL 2 . Embed fragile sign in HH 2 . See Fig. 2;

Figure 3. Bit Plane decomposition of 256 gray-scale Image

Step4. Decomposed HH ₂ into lαβ color component and transform the β component into gray image [9]. Bit plane decompose to the gray image. The Bit Plane 2 is the embedding region in HH 2 . Fig. 3 shows a bit plane decomposition of normal Lena as a 256 gray-scale image.

B. Embedding rules

The embedded rules of CL-DCT are as follows:

Rule1. Odd and Even of DCT coefficient separately stand for 0 and 1;

Rule2. Embed information into LH 2 and HL 2 with RAID1, as shown in Fig. 4;

Robust Information LL 2	Embed Information LH 2
Embed Information HL 2	Fragile Sign HH 2

Figure 2. Embedded region strategy

Figure 4. Knight-tour route

Step2. Transform LL ₂ component ( N × N ) with DCT. Choose the interval distribution [( N ² /4) - 1, N ² - 1] of DCT coefficient as embedding region in LL 2 ;

Step3. Transform LH 2 and HL 2 ( N × N ) with DCT. Choose the interval distribution [( N 2/ 4) - 1,( N 2/ 2) - 1] of DCT coefficient as embedding region in LH ₂ and HL ₂;

Rule3. Order by [( N ²/4) - 1, N ² - 1] to embed information into LL ₂, and order by [( N ² /4) - 1, ( N ² /2) - 1] in LH ₂ and HL ₂;

Rule4. Order by Knight-tour route traversal to embed information into HH 2 . The Matrix of 9×9 Knight-tour is T . In the matrix T , “1” stands for the initial point of Knighttour rout [10].

1 34 3 16

4 173243

33 2 3564

18 5 6873

(a) Lena Normal

(b) Bit Plane 7

(c) Bit Plane 6

T = 81 74 63 70

6 198075

79 76 61 22

20 7 78 59

77 60 21 8

31 42 37 14 29 3615304138 6954392813 4465465340

67  72  55  1227

71  56  51  2611

50  9  24  5748

23  58  49  1025

(d) Bit Plane 5

(e) Bit Plane 4

(f) Bit Plane 3

According to the matrix tour route of 9×9 image.

T , Fig. 5 shows the Knight-

(g) Bit Plane 2

(h) Bit Plane 1

(i) Bit Plane 0

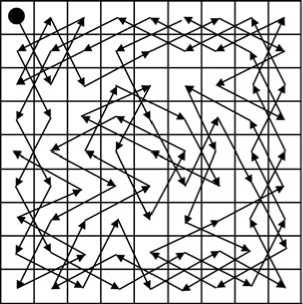
Figure 5. Knight-tour route

• C.    Embedding process

Information hiding scheme based on CL Multi-wavelet and CL-DCT divided into seven steps. Fig. 6 shows general process:

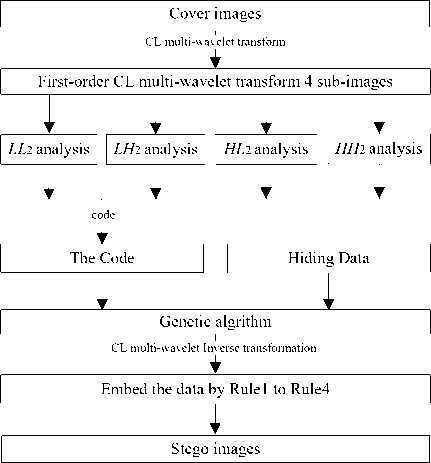
Figure 6. Embedding process for CL-DCT

Step1. Transform the cover image with first-order CL multi-wavelet to obtain four LL 1 sub-images;

Step2. Transform the LH 2 and HL 2 component ( N × N ) with DCT. Draw the data from DCT coefficient of LH ₂ and HL 2 order by [0,( N ²/2) - 1] according to Rule2. The data separately denoted as CLL ₁⁽²⁾ and CLL ₁⁽³⁾ . CLL ^ = x^,x 2 ²⁾, l , x<²⁾ and 0 <  m <  N2/2    .

CLL ( ³⁾ = x (3) , x (3) , l , x ( ³⁾ and 0 <  n <  N ²/2 . The final 11 , 2 ,, n               .

analysis result denoted as C ;

C = ( x , , x ₂ , l , x ) = ( x ⁽²⁾, x 2²⁾, l , x^,x <³⁾ , x 2³⁾, l , x n , ³⁾ ) (1)

Step3. Use Chebyshev mapping [11] of chaotic map algorithm to optimize information, as defined in (2) and (3). Suppose the parameter is μ, η and xk . The chaotic sequence after the Chebyshev mapping is Ch. The Pre- hiding bit series is Cpre. Scrambling formula is defined in (4), CIN =(b1,b2,l,b,) = (bx,bx,Kbm+„_pbm+„);

^-1 <  ^xk + i <  П П <  xk + i < ¹

xk _{+ 1} = cos ( ^ arccos( xk ) )                 (3)

x

INpreh

Step4. In order to optimize the sequence of embedded bits with genetics algorithm [12], suppose F as the amount of the same bit value in matched positions between C_I^x_N and C . The optimization model based on

CL-DCT is (5). Get the optimal solution x_n' >  П and ц' by genetic algorithms optimization;

F ( x„ , П , Ц ) = Max ^ ( x ® bi)      (5)

Step5. Bring x _n' >  П and Ц into (2) (3) and (4) to get optimization embedded bits C_I^y_N , CIN = by , by , K bn - 1 , bn . Embed CIN into DCT coefficients of LH ₂ and HL ₂ between ( N ²/4) - 1 and ( n ²/2) - 1;

Step6. Transform the LL 2 component ( N × N ) with DCT. LL 2 is the most robust region in four LL 1 sub-images. In order to judge and recover the imperfect information, The CL-DCT embed the check code of RAID1, optimized code of Chebyshev scrambling ( xn' >  П and Ц ) and Hash value of embedding information (recorded as R^L ) into DCT coefficient between ( N ² /4) - 1 and N ² -1;

Step7. HH 2 is the most vulnerable region in four subimages. Embed the Hash of embedding information (recorded as R^H ). Receiver can judge whether the stego image is attacked by comparing R^H and R^L .

• D.    Extracting information

Extracting information is divided into five steps. Fig. 7 shows general process:

Stego images

CL multi-wavelet transform

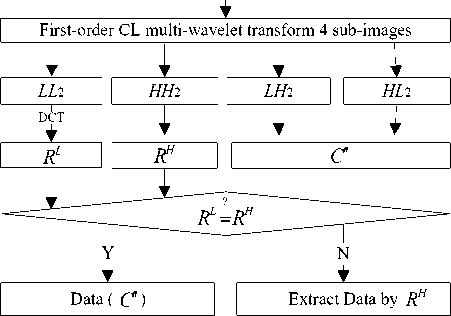
• Figure 7. Process for Extracting Information

Step1. Transform the cover image with first-order CL multi-wavelet and get four LL 1 sub-images;

Step2. Transform LL 2 , LH 2 and HL 2 component with DCT. Draw the R^L from LL 2 component. Draw the data from LH 2 and HL 2 component with the order in the Knight-tour route, and denoted as C ′′ . Draw the R^H from HH 2 ;

Step3. if R^L = R^H .The C ′′ is the secret information. if R^L ≠ R^H .The process continues;

Step4. if R^L = R^H .The C ′′ is the secret information. if R^L ≠ R^H .The process continues;

Step5. Draw the check code of RAID1 from LL 2 component and use them to process C ′′ and get final secret information.

• III.    Information Hiding Algorithm design

• A.    Invisibility and Robustness Analysis

Use high energy component LL 1 as information hidden area, the energy percentage of which is about 97.36% in the total image energy. This feature satisfies robust basic requirement of hiding area.

Main hidden area of information are LH 2 and HL 2 components, the energy of which is much lower than the energy of LL ₂ (energy distributions of LL ₁ are 2.51% and 0.62%, energy percentages of the total energy are 2.51% and 0.60%).

This characteristic meets the invisible basic condition of hiding area. Choose high frequency of DCT coefficients in high energy component LL 2 , meeting invisible hiding area rule of DCT. Choose low frequency of DCT coefficients in medium energy components LH 2 and HL ₂, which qualifies robust hiding area rule of DCT. The general design idea of information hiding algorithm based on CL multi-wavelet and DCT is hiding information in low energy area of high energy component. This design can satisfy robustness and invisibility and solve the opposition between them well.

• B.    Anti-Steganalysis Analysis

The information hiding analysts generally use “all black” characteristic of LH 2 and HL 2 in CL component to analyze hidden information. Without obvious texture and pattern feature, information hidden in LH 2 and HL 2 is easily to expose.

Considering about the above, choose high frequency coefficient interval of DCT as hiding area to avoid information hiding analysis effectively. With consistent statistical properties of Chebyshev traversal and Knighttour route, zero mean white noise [13, 14], hidden information is of good distribution and hidden characteristics, thus improve the anti-steganalysis of algorithm. β components of HH 2 in lαβ color space are grayed to satisfy sensitive perceptivity and anti-steganalysis based on general LSB analysis.

C. Sensitivity Analysis

Hide Hash value in LL 2 robust unit with highest energy and LL 2 fragile sign unit with lowest energy. By contrast judgment on Hash value, the system can have sensitive perception against distortion.

IV. Simulation Experiment

Simulation environment of the algorithm is Matlab7.0.0.19920. Cover image is Lena (256×256) as shown in Fig. 8(a). Stego image is binary image Baboon (64×64) as shown in Fig. 8(b).

A. Invisibility Experiment

Fig. 8(c) shows stego image based on CL-DCT. PSNR

value equals 41.3295. It shows that this method is of

better invisibility.

(a) Cover image

(b) Hiding information

(c) Stego image

Figure 8. Hiding and result of CL-DCT

Invisibility is determined by Information content. Embed information in 200 images randomly. 2 ^k is used to denote bit quantity (0 ≤ 2 ^k ≤ 4096). Fig. 9 shows the PSNR of different embedding quantity. The experimental results indicated that when k ≤ 12, it is of better

invisibility (PSNR ≥ 41.3295).

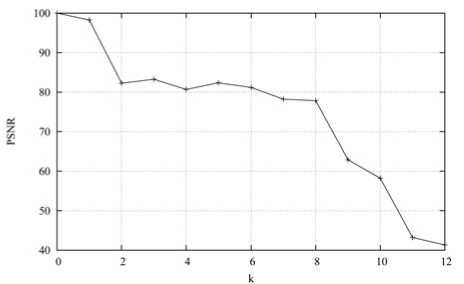
Figure 9. Experiment of embedding dense and corresponding Invisibility

B. Robustness Experiment

Define texture evaluation and modification rate of binary image ( n × n pixels) separately in (6) and (7).

n -1 n -1

∑∑ f ( i , j ) ⊕ f ( i + µ , j ± η )

w =

i =0 j =0

2 n ²

p =

∑∑ f ( i , j ) ⊕ f ′( i , j )

n ²

Where n = N /2 d , d e {1,2, L ,log₂( N - 1)} . f ( i , j ) and f '( i , j ) are separately for the pixel at ( i , j ) of normal and extraction image with n × n pixels.

Robustness test algorithm is defined in (8). Q is robustness test value and Q e [0,1] . In the following experiments, μ = η =1. Expand Q 100 times to accommodate judgment habit.

Q = w ( 1 ^- p ) ⁽8)

Fig. 10 shows the result of different attacks such as JPEG2000 compression, cutting, filtering and noise.

(c) mean filter_[3,3] Q =73.01

(d) wiener2 filter_[3,3] Q =61.36

(e) Gaussi _ μ =0, σ ²=0.003 Q =75.79

(f)' salt & pepper '_ d =0.15 Q =45.30

Figure 10. Results of robustness experiment

Images are vulnerable to compression and cutting attacks, Fig. 11 shows the Q ’s value corresponding to ratio of these attacks.

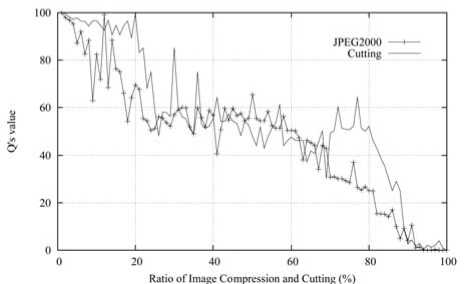
Figure 11. JPEG2000 and cutting experiment compression below 75%, cutting below 83%, common filtering and adding noise.

• C.    Experiment of ability against steganalysis

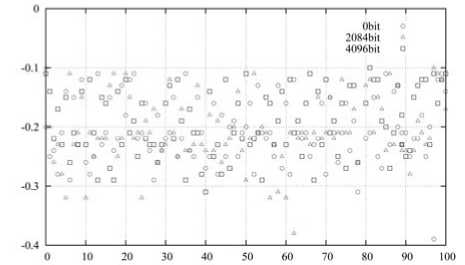
Higher order statistics detection algorithm based on wavelet coefficients (HOSWC) is a general detection algorithm [15]. Use the algorithms above-mentioned to analyze the performance of CB2-CFS. Experiment results are shown in Fig. 12 and Fig. 13.

Objective Images (ps)

Figure 12. Steganalysis experiment result of HOSWC to CL-DCT

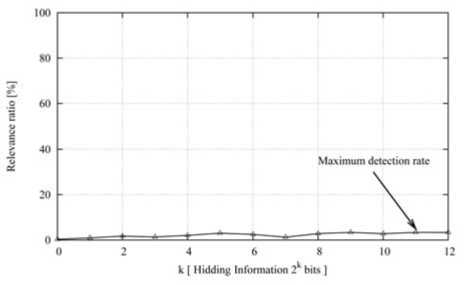
Figure 13. Steganalysis detection rate of HOSWC to CL-DCT

Fig. 12 shows there is no one or more threshold value found to recognize which pictures embedded information in 100 images. Use 1000 images to test, as shown in Fig. 13, largest detection rate below 3.3%. Experiments show that CL-DCT is of better anti-steganalysis.

• D.    Experiment of sensitivity to image attacks

  Sensitivity to image attacks is the peculiar characteristic in CL-DCT. Comparing R^L with R^H indicates the algorithm has excellent sensitivity of image processing. TABLE II lists the detectable rate when JPEG2000 compression ratio is 5%, random cutting ratio is 5%, [3, 3] median filter, Gaussian ( μ =0, σ ²=0.003) and ' salt & pepper '( d =0.15). The average of detectable rate is 99.72%.

According to experiment, embedded information can be identified when Q reach about 30. Fig. 10 and Fig. 11 show that CL-DCT is robust against JPEG2000

TABLE II.

D etectable rate of attacks

ATTACKS	JPEG2000	Cutting	Filtering	Gaussian	' salt pepper '
Detectable rate	99.98%	99.94%	99.98%	98.79%	99.92%

• E.    Experiments of invisibility comparison

According to PSNR, CL-DCT has advantages in invisibility compared with DWT-DCT and DCT-LSB. TABLE III shows that invisibility increases by 6.00% averagely when embedding rate is 25%.

TABLE III.

I nvisibility comparison based on PSNR

Algorithm	CL-DCT	DWT-DCT	DWT-LSB
PSNR	41.3295	38.3270	39.9501

• F.    Experiments of robustness comparison

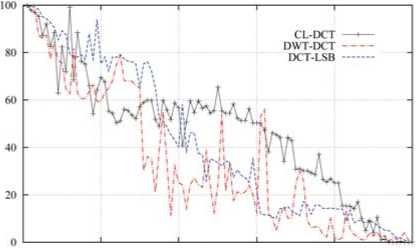
Fig. 14, Fig. 15 and TABLE IV show robustness comparison results when embedding rate is 25% based on the Q ’s value.

О            20           40           60           80           IOO

Ratio of Image JPEG2000 Compression (%)

Figure 14. Compression comparison

The Q ’s value of CL-DCT in JPEG2000 compression is 44.7315. DWT-DCT is 32.9980, and DCT-LSB is 42.6989. Indicate CL-DCT is better robustness at JPEG2000.

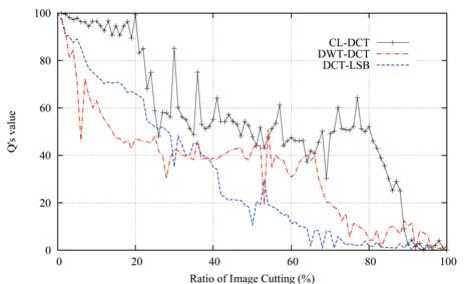
Figure 15. Cutting comparison

The Q ’s value of CL-DCT in cutting is 50.4678. DWT-DCT is 33.7810, and DCT-LSB is 31.0311. Indicate CL-DCT is better robustness at cutting.

TABLE IV.

RTV comparison of filtering and noise

Attacks	Information Hiding Algorithm
	CL-DCT	DWT-DCT	DCT-LSB
[3,3] median filter	72.11	45.33	60.11
[3,3] wiener2 filter	60.54	58.74	49.60
Gaussi ( μ =0, σ 2=0.003)	72.17	46.90	60.14
' saltpepper '( d =0.15)	43.54	53.48	42.15

TABLE IV show that CL-DCT have better performance in robustness under filter and noise except compared with ' salt & pepper ' noise of DWT-DCT.

• V. Conclusions

Propose an Information hiding scheme based on CL and DCT. Take advantage of the energy distribution ratios in the four sub-images after first-order CL multiwavelet transformation, and use DCT to generate the DCT coefficients. Change the DCT coefficient to achieve information hiding. Chose LL 1 , the energy of which accounts for 97.36% of the total image energy, as the algorithm hiding area. The feature of LL 1 can meet the basic requirement of robustness hiding area. The main information hiding areas are LH 2 and HL 2 , the energy of which is much lower than the energy of LL 2. The energy of LH ₂ and HL ₂ separately account for 2.51% and 0.62% of LL 1 , and approximately account for 2.44% and 0.60% of the image total energy. The energy feature of LH 2 and HL 2 can meet the basic requirement of invisibility hiding are. Choose high frequency DCT coefficients of LL 2 component according to invisibility rule of DCT hiding area. Choose low frequency DCT coefficients of LH 2 and HL ₂ according to robustness rule of DCT hiding are. The statistical properties of Chebyshev traverse and zero mean white noise are consistent [16], which is of good distribution and hiding characteristics to improve anti-steganalysis. Decompose HH 2 according to lαβ color space. Hide information with LSB method in β components after gray-scaling which can meet the requirement of sensitivity and anti-steganalysis against common analysis based on LSB.

The future work is focus on alternating selection of bit plane, choosing embedded module and robustness parameters in LL 2 in order to improve invisibility, robustness and tampering perception. Especially research on applications in information hiding based on the theory of Image Multiscale Geometric Analysis [17].

Acknowledgment

This work was supported by The Graduate Starting Seed Fund of Northwestern Polytechnical University (NPUZ2010). This work also has been supported by the National Defense Fund of China (C2720061361) and the

National Science and Technology Major Special Project (2008ZX03006)

The authors wish to thank all the reviewers for their valuable comments and suggestions that improved the quality of this paper.