Класcы преобразований нестационарного сигнала в информационно-измерительных системах. II. Время-частотные преобразования
Автор: Меркушева А.В.
Журнал: Научное приборостроение @nauchnoe-priborostroenie
Рубрика: Оригинальные статьи
Статья в выпуске: 2 т.12, 2002 года.
Бесплатный доступ
Представлены аналитическая форма и характеристики время-частотных преобразований, являющихся средством анализа нестационарных сигналов, в том числе большинства сигналов в информационно-измерительных и информационно- управляющих системах. В примерах двумерное представление модельных сигналов при преобразованиях Зака и Вигнера сопоставлено с традиционным методом кратковременного преобразования Фурье. Дана систематизация и взаимосвязи преобразований время-частотного класса.
Короткий адрес: https://sciup.org/14264237
IDR: 14264237 | УДК: 621.391.266.037.372
Текст научной статьи Класcы преобразований нестационарного сигнала в информационно-измерительных системах. II. Время-частотные преобразования
Представлены аналитическая форма и характеристики время-частотных преобразований, являющихся средством анализа нестационарных сигналов, в том числе большинства сигналов в информационноизмерительных и информационно-управляющих системах. В примерах двумерное представление модельных сигналов при преобразованиях Зака и Вигнера сопоставлено с традиционным методом кратковременного преобразования Фурье. Дана систематизация и взаимосвязи преобразований время-частотного класса.
ОБЩИЕ ПОЛОЖЕНИЯ
Совершенствование вычислительных средств, развитие существующих и разработка новых математических методов позволяют применять в информационно-измерительных системах (ИИС) для анализа нестационарных сигналов время-частотные преобразования. Такие преобразования локализуют по времени каждую частотную компоненту сигнала и таким образом дают полное представление о динамике его частотного спектра.
Существует несколько разновидностей время-частотных преобразований (ВЧП), которые представлены во многих статьях, посвященных интенсивно развивающемуся научному направлению — Signal processing. Анализ и систематизация время-частотных преобразований направлены на возможность их более широкого применения в прикладных исследованиях, включающих обработку нестационарных процессов в ИИС, а также имеют целью способствовать более полной (а возможно, и более корректной) интерпретации исследуемых явлений, порождающих эти процессы.
Вместе с тем к ИИС, реализующим контроль, обработку, анализ и интерпретацию нестационарных сигналов (а также управление — в качестве реакции системы) относится подавляющее большинство информационных и управляющих систем (ИУС). Существует тенденция постепенной интеллектуализации всех фаз функционирования системы и всех этапов формирования информационной базы для выработки решения, а также практика передачи системе самой процедуры принятия решений. В связи с этим становится особенно актуальным расширение методической базы для новых усложненных форм преобразования измерительной информации в ИИС—ИУС и, в частности, двумерной формы представления сигнала, которую предоставляют методы время-частотных преобразований.
В предшествующей статье [1] представлены элементы теории, которые лежат в основе всех преобразований нестационарных сигналов в ИИС, показана взаимосвязь преобразований, общность их выражения с помощью ядра и требования на соотношения, ограничивающие аналитический вид ядра, которые могут обеспечить те или иные полезные свойства время-частотного преобразования. Показаны методы построения некоторых видов преобразований. Представляется, что это может служить базой для расширенного анализа видов и модификаций семейства время-частотных преобразований.
Принятая форма изложения позволяет использовать статью даже без знакомства с предшествующим материалом.
В приложении дана сводка формул преобразований, в ссылках на них в тексте перед номером стоит буква П.
ЛИНЕЙНЫЕ ВРЕМЯ-ЧАСТОТНЫЕ ПРЕОБРАЗОВАНИЯ
Развитие методов время-частотных преобразований (ВЧП) и их модификаций, относящихся к классу время-масштабных преобразований, связано с работами Габора, Вигнера, Вилле, Пейджа, Моэла, Коэна, Мейера, Маллата, Добеши [2–11]. Для результата преобразования применяется также термин "время-частотное распределение", который ассоциируется с двумерным вероятностным распределением энергии сигнала по времени и частоте [4, 12]. Распределение по времени и частоте, как результат преобразования, не всегда имеет свойства распределения в вероятностном смысле. Не все преобразования дают положительные распределения с правильными маргиналами, т. е. с правильными частными распределениями энергии сигнала отдельно по времени и по частоте (при проинтег- рированной второй переменной распределения). Наличие правильных маргинальных распределений желательно, т. к. расширяет возможности интерпретации результатов применения ВЧП.
К ранним формам ВЧП относится преобразование Габора (Gabor) [13–16]. Это ВЧП основано на локализации сегмента сигнала с помощью окна h( т - t ) = ( a4П ) ехр{ - ( т - t ) 2 / a 2} и на последующем преобразовании Фурье этого сегмента. Окно эквивалентно гауссиану, в котором g = a /V2 . Обратное преобразование Габора реализуется по аналогичной схеме в соответствии со вторым соотношением (П1) из сводки формул.
Развитие преобразования Габора состояло в использовании различных функций для окна и привело к кратковременному преобразованию Фурье (КПФ) [17, 18] — (выражение (П2) в сводке). Квадрат модуля КПФ получил название спектрограммы. Подобная по концепции спектрограмма может основываться и на других ВЧП. Подход, использованный в преобразованиях Габора и КПФ, позволяет улучшить частотное разрешение за счет сужения окна в частотной области и, следовательно, расширения его во временнóй области, а это ведет к ухудшению временнóго разрешения. Имеется и обратная картина, т. е. сужение временнóго окна ведет к расширению его частотного образа и к ухудшению разрешения по частоте. Если изменения состава частотных компонент сигнала происходят достаточно быстро (имеет место кратковременная нестационарность), то спектрограмма неадекватна задаче обработки такого сигнала.
К линейным преобразованиям относится ВЧП Зака (Zak) [19, 20], вид которого представлен выражениями (П3) в сводке. Преобразование имеет свойство квазипериодичности
Z ( t + 1, to ) = Z ( t , to ),
Z (t, to + 1) = e 2njtZ (t, to), в связи с чем в плоскости время—частота его достаточно вычислять на квадрате [0, 1]×[0, 1].
Преобразование Зака используется при построении ВЧП Вигнера (Wigner) методом теории фреймов и связано с алгоритмом преобразования Вильсона (Wilson). Последнее основано на базисе с двухмодальной формой кривой, имеющем хорошую локализацию одновременно в частотной и временнóй областях [21].
Спектральные исследования на основе ВЧП Зака редки. Поэтому проведено моделирование с использованием комплексного сигнала, синтезированного на ЭВМ и содержащего две комплексные гармонические компоненты с возрастающими частотами. Временнáя локализация сигнала опреде-
(n - N/2)2 — лена гауссианом e N , где N = 4000:
-
- ( n - N /2) 2
5 (n) = e N x x { 2nj[0.012 n+0.0005 n (n-N/2)] +
+ e 2 n j [0.003 n + 0.0005 n ( n - N /2)] } (1)
Для этого сигнала составлена программа, с помощью которой выполнено кратковременное преобразование Фурье и преобразование Зака. Результаты преобразований представлены в логарифмическом масштабе на рис. 1.
Как следует из графиков, КПФ не имеет удовлетворительного разрешения по частоте и не позволяет разделить отдельные компоненты сигнала. Преобразование Зака позволяет достаточно четко разделить две компоненты возрастающего по частоте сигнала, но достигается это значительным уменьшением частотного диапазона преобразования. Такое свойство преобразования Зака показывает его преимущество и позволяет использовать совместно с другими преобразованиями для увеличения разрешения по частоте.
БИЛИНЕЙНЫЕ ВРЕМЯ-ЧАСТОТНЫЕ ПРЕОБРАЗОВАНИЯ
К билинейному типу принадлежит ряд ВЧП [22]. Для анализа изменяющегося во времени спектра сигнала используют преобразование Вигнера W ( t , to ) [2, 11, 16] и двойственную функцию А ( t , to ) [4], представленные выражениями (П4) и (П5) в сводке. ВЧП Вигнера, подобно вероятностному распределению, имеет маргинальные распределения — частные распределения по времени и по частоте. Маргинальные распределения по частоте и по времени связаны с сигналом s ( t ) и его преобразованием Фурье S5 ( to ) соотношениями:
те
J Ws (t, to) • dt =|S (to )|2, -те те
J W5 ( t , to ) • d to =| 5 ( t )| 2 . -те
Преобразование Вигнера вещественно и инвариантно к сдвигу по времени и частоте. Однако оно билинейно относительно сигнала, поэтому преобразование суммы компонент сигнала вида s ( t ) = s 1 ( t ) + s 2 ( t ) приводит к появлению так называемых кросс-членов W s 1 s 2 ( t , ω ), W s 2 s 1 ( t , ω ), вызывающих явление интерференции:
W s ( t . to ) = W s , ( t , to ) + W s 2 ( t , to ) +
+ W s , s 2 ( t , to ) + W s 2 s 1 ( t , to ).
где
∞
W s , s 2 J s , ( t + т I2) . s^ t - т I2) . e d T .
-∞
Предложен ряд модификаций преобразования Вигнера, направленных на снижение вклада кроссчленов. Во-первых, это применение аналитической формы s a ( t ) сигнала (с добавлением мнимой части), которая получается на основе применения к сигналу преобразования Гильберта по соотношению:
s a ( t ) = s ( t ) + i J^I Z l d y .
t - y
-∞
Аналитическая форма сигнала не имеет отрицательных частот в спектре Фурье, поэтому отсутствует интерференция положительных и отрицательных частот. Получение аналитической формы s a ( t ) реализуется несложным алгоритмом на основе преобразования Фурье сигнала.
Во-вторых, снижению кросс-членов способствует введение функции окна h ( t ) при вычислении ВЧП Вигнера [23, 24]. Такая процедура приводит к преобразованию псевдо-Вигнера — W p ( t , to ) в форме (П6), (П7), приведенной в Приложении. Для вещественной четной функции окна h ( т / 2) выполняется условие h ( т / 2) h* ( - т / 2) = h 2 ( т / 2), поэтому ВЧП псевдо-Вигнера подавляет влияние кросс-членов. В то же время избыточное сужение окна ведет к размытию распределения в плоскости время—частота, поэтому ширину окна необходимо соотносить с требованиями к контрастности результирующего время-частотного распределения. ВЧП псевдо-Вигнера нестационарного сигнала не всегда положительно [25]. В отличие от преобразования Вигнера оно вычисляется через сегмент данных (вырезанных окном), и при этом не происходит размазывания во временной области, как у кратковременного преобразования Фурье или как у спектрограммы.
В-третьих, уменьшению влияния кросс-членов способствует введение дополнительной частотной весовой функции. Преобразование такого типа называется сглаженным ВЧП псевдо-Вигнера (П8). По современным представлениям [4, 22, 23, 26, 27],
а
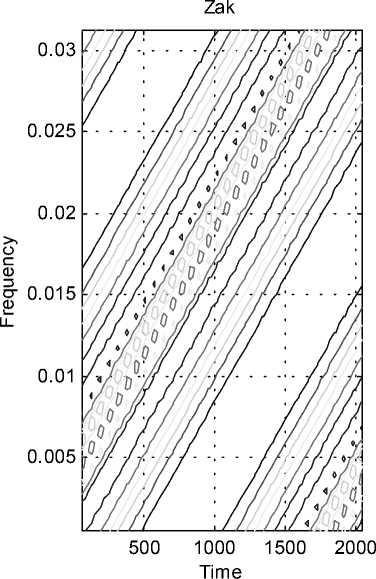
Рис. 1 . Время-частотное представление тестового сигнала (1). а — Преобразование Зака.
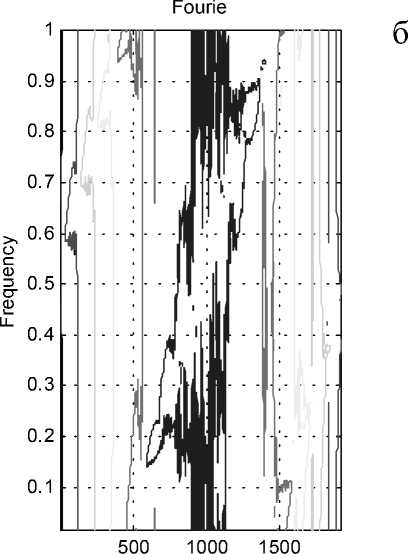
б — Кратковременное преобразование Фурье
Time
а
W igner
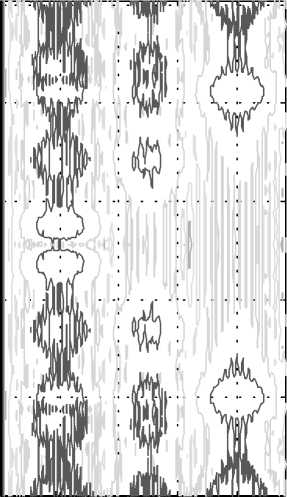
10 11 12 13 14
Tim e

Рис. 2. Время-частотное представление сигнала (2) на основе преобразований Вигнера (а) и кратковременного преобразования Фурье (б)
б
билинейные время-частотные преобразования могут интерпретироваться как сглаженные или комбинированные варианты преобразований Вигнера с соответственным типом сглаживания, степенью подавления кросс-членов и некоторой потерей способности к концентрации распределения энергии сигнала в время-частотной области (свойственной ВЧП Вигнера). В частности, КПФ может быть выражено двойной сверткой преобразований Вигнера от сигнала и от окна:
| КПФ s ( t , ω ) | =
∞
∞
∞∞
= 2 1 π ∫ ∫ Ws ( τ , Ω ) Wh ( t - τ , ω -Ω )d τ d Ω .
-∞
-∞
Для тестового сигнала, состоящего из трех локализованных гармонических компонент вида (2):
s ( t ) = e -3.7( t -11) ⋅ cos[2 π f 1( t - 11)] +
+ e-3.7(t-11) ⋅ cos[2πf2(t - 11)] +
+ e -3.7( t -14) ⋅ cos[2 π f 2 ( t - 14)], (2)
составлена программа и получены преобразования Вигнера и КПФ, результаты которых представлены в логарифмическом масштабе на рис. 2. Преобразование Вигнера позволяет правильно локализо- вать две гармонические компоненты с частотами f1 = 12 Гц и f2 = 5 Гц, появившиеся в среднем во время t = 11 c, и одну частотную компоненту f2 = 5 Гц со временем t = 14 c. Явления интерференции как вдоль частотной, так и вдоль вре-меннóй осей значительно ухудшают качество преобразования. КПФ, выполненное при тех же условиях, не позволяет локализовать гармонические составляющие сигнала, но не имеет свойства интерференции.
Более обширным классом преобразований, к которому относится и преобразование Вигнера, является класс билинейных преобразований Коэна (L. Cohen) [3, 4] (называемый также обобщенным классом), — соотношение (П9) в Приложении. Особенность этого класса состоит во введении ядра общего вида Ф( τ , ξ ), свойства которого отражаются на характеристиках ВЧП. Если ядро Ф( τ , ξ ) = 1, то преобразование класса Коэна дает преобразование Вигнера. Ядро, построенное по схеме двойственной функции от некоторой весовой функции — окна h ( t ), имеет вид ∞
Φ h ( τ , ξ ) = ∫ h ( u + τ /2) ⋅ h *( u - τ /2) e - jξu d u .
-∞
Преобразование Коэна Cs(t,ω,Фh) с этим ядром превращается в спектрограмму, т. е. в квадрат модуля кратковременного преобразования Фурье (с той же весовой функцией h) от сигнала s(τ) [4, 22]:
Cs (t,to, Фh) =|КПФ(t,to) |2 = to 2
= J s (t ) h (t - t ) e -j toT d T .
-TO
О взаимосвязи ряда билинейных ВЧП свидетельствует также соотношение (3), которое связывает преобразование Коэна C s ( t , to , Ф) от сигнала с двойственной функцией A s ( t , Z ), определенной для этого же сигнала:
частоте. Преобразование выражается соотношениями (П14), (П15). Сглаженное ВЧП псевдоБертрана PB(t , to ) связано с основным (оригинальным) преобразованием Бертрана B s операцией обобщенного аффинного сглаживания по соотношениям
~
PB s ( t , to ) =
TOTO z,,
= f p s ( t , Z ) • П I to ( T - 1 ), Z
J J l
0 -to\
П ( t , Z ) =
,
Cs ( t , to , Ф) =
= тЧЛФ (t , 5 ) A s (t , 5 ) e
2 П
= Z JJ G (u ) ^ ( V ) u
• V1Z l
A ( v ) ^ Л ( u ) J
X
- j ( b + toT )d s d T .
XV *[? l
.y - v ) ^ Л ( - u ) J
• exp[2 n / 5 ( v ) tZ ]d u d v .
Таким образом, согласно выражению (3) ВЧП Коэна — это двумерное преобразование Фурье от произведения ядра Ф( т , 5 ) с двойственной функцией A s ( t , Z ) и от сигнала s ( t ) .
Для снижения влияния кросс-членов на распределение энергии сигнала при ВЧП Коэна предложено преобразование со специальной весовой
функцией вида
2n
T
U 1 J
• h I — I . Такое преобразова- l т J
ние называется время-частотным преобразованием со сниженной интерференцией [28].
К группе билинейных ВЧП принадлежат преобразование Бертрана (Bertrand), преобразование псевдо-Бертрана и сглаженное преобразование псевдо-Бертрана. По сути дела они относятся к время-масштабным преобразованиям [29], хотя параметр масштаба обозначают символом частоты ω и называют частотой. Преобразование Бертрана определяется выражением (П10) в сводке и имеет маргинальное распределение по частоте. ВЧП Бертрана имеет два недостатка: наличие интерфе-
ВЧП Бесселя (Bessel) [32] принадлежит обобщенному классу Коэна, который порождает также ряд других преобразований (Вигнера, Чои—Виль-ямса). ВЧП Бесселя построено на основе ядра:
J\(2 rnaZT ) _ _ , , „ r
Ф(5,т) =---------, где J1 — функция Бесселя яа^т
1-го рода, и имеет выражение (П16 в Приложении). Преобразование Бесселя вещественное, обладает свойствами ковариантности для сдвига по времени или по частоте и наличия маргинальных распределений — после интегрирования преобразования по одной из переменных (по t или ω ).
К билинейным преобразованиям класса Коэна относится преобразование Чои—Вильямса (Choi, Williams) [31, 32]. Оно построено на основе использования экспоненциального ядра в обобщенной форме преобразований класса Коэна и имеет форму
ChW s ( t , to ) =
ренции и невозможность реализации в реальном времени, связанную с тем, что в преобразование входит весь сигнал при вычислении его в любой точке ( t , ω ).
ВЧП псевдо-Бертрана является более совершенным преобразованием, имеющим асимптотически те же свойства, что и преобразование Бертрана. Это ВЧП подавляет кросс-компоненты интерференции вдоль частотной оси и допускает реализацию в реальном времени. Вид преобразования дают соотношения (П11), (П12) в сводке.
Для одновременного уменьшения интерференции вдоль временнóй и частотной осей разработано сглаженное ВЧП псевдо-Бертрана, в котором
JJJ ехР{’(-6t - tv + tou)}• s(u + т /2) X
X s * ( u - т /2) • exp -
0 2 t 2
о
- d u d T d 0 .
введено дополнительное сглаживающее окно по
Опыт использования преобразования Чои— Вильямса связан с медицинскими исследованиями и обнаруживает существенное снижение интерференции в результатах, связанных с время-частотным представлением биомедицинских сигналов [32].
Существуют также не нашедшие распространения формы ВЧП, введенные с целью получения улучшенных характеристик преобразования. К ним относится полиномиальная L -модификация
преобразования Вигнера [33, 34]. В этом преобразовании с помощью параметра L масштабируется фаза и временнáя шкала интегрирования в выражении преобразования Вигнера, в связи с чем преобразование названо масштабированным L -преобразованием Вигнера:
LW s ( t , ω ) =
∞
= ∫s[L](t+τ/2)s[L]*(t-τ/2)ejωτdτ.
-∞
В этом выражении s [ L ] — модификация сигнала, которая состоит в том, что фаза умножается на L , а амплитуда не изменяется, т. е. s [ L ] ( t ) = | s ( t ) | e jLϕ ( t ) , если сигнал имеет вид s ( t ) = | s ( t ) | e jϕ ( t ) .
L -преобразование Вигнера улучшает разрешающую способность для отслеживания времен-нóго изменения мгновенной частоты сигнала при сложном законе ее изменения, например при законе в форме полинома выше второго порядка (черп-сигнал выше 2-го порядка). За счет такого усложнения алгоритма преобразования достигается уменьшение помех, связанных с интерференцией при время-частотном анализе сложных сигналов
Аналогичные по структуре L -модификации построены для преобразований псевдо-Вигнера и сглаженного преобразования псевдо-Вигнера. В [33] показан также способ L -модификации кратковременного преобразования Фурье. Однако возможности и преимущества этой формы билинейных время-частотных преобразований пока не исследованы.
КЛАССИФИКАЦИЯ ВРЕМЯ-ЧАСТОТНЫХ ПРЕОБРАЗОВАНИЙ
Приведенная выше систематизация время-частотных преобразований и их отдельных характеристик позволяет ввести классификацию ВЧП на группы на основе признаков общности структуры и свойств. Классификация преобразований представлена на рис. 3. Для отражения логики развития ВЧП включен этап классических форм анализа сигнала (т. к. на их базе возник новый вид время-частотного спектрального анализа) и этап, отражающий первые реализации ВЧП в области физики (в квантовой механике) и связанный с работами Моэла, Вилле, Вигнера, Мейера, Коэна [3, 9, 11, 35]. Этап дал операторный метод представления физической величины в пространстве координата—импульс или координата—частота (т. е. двумерное представление, эквивалентное время-частотному анализу), ввел понятия ковариантности при сдвиге одной из переменных (в случае
ВЧП — при сдвиге времени или частоты), трактовку результатов преобразования как вероятностное время-частотное распределение энергии сигнала с желательными маргинальными (частными) распределениями при интегрировании по одной из переменных. При этом представление вре-мя-частотного распределения реализовалось по методу характеристической функции.
Второй основой развития время-частотного анализа явилось построение ВЧП Габора. Расширение типов окна такого преобразования привело к группе кратковременных преобразований Фурье. КПФ — наиболее простое и доступное ВЧП, однако для него форма ячейки разрешения ( ∆ t , ∆ ω ) постоянна на всей плоскости время—частота [10, 12]. Это существенный недостаток, т. к. выбор хорошего разрешения на малых частотах ведет к избыточному представлению сигнала на всем частотном диапазоне, и аналогично для быстро протекающего сигнала, например переходного процесса, выбор хорошего разрешения по времени (необходимого для анализа ранней стадии процесса) соответствует избыточности ВЧП-представления сигнала на всем временнóм интервале.
ВЧП Габора, КПФ и преобразование Зака составляют группу линейных ВЧП. При этом в преобразовании Зака заложено временнóе масштабирование сигнала, однако масштабный параметр (постоянная λ ) лишь деформирует шкалу времени в целом. ВЧП Зака является вариантом преобразования Фурье с масштабированной шкалой времени и симметризованное относительно произвольной точки τ . Ячейка разрешения ВЧП Зака также постоянна на всей плоскости время—частота. Это делает преобразоване Зака в большей части процедурным преобразованием, которое используется в схемах расчета других ВЧП, особенно по методу теории фреймов [36, 37].
Обобщенную форму билинейных ВЧП представляет класс Коэна. Класс обобщил предшествующие разрозненные попытки двухпараметрического анализа сигнала в формате время—частота и операторный метод, определил структуру ВЧП через ядро преобразования и установил условия наличия маргинальных распределений в форме требований на свойства ядра.
Класс билинейных преобразований включает три модификации преобразования Вигнера (Вигнера, псевдо-Вигнера и сглаженное псевдо-Вигнера), преобразование с ядром Бесселя, преобразование Чои—Вильямса с экспоненциальным ядром и три модификации преобразования Бертрана. Модификации ВЧП Вигнера и Бертрана построены по общей логике введения сглаживающих фильтров (окон), но существенно отличаются по структуре самого преобразования (см. Приложение).
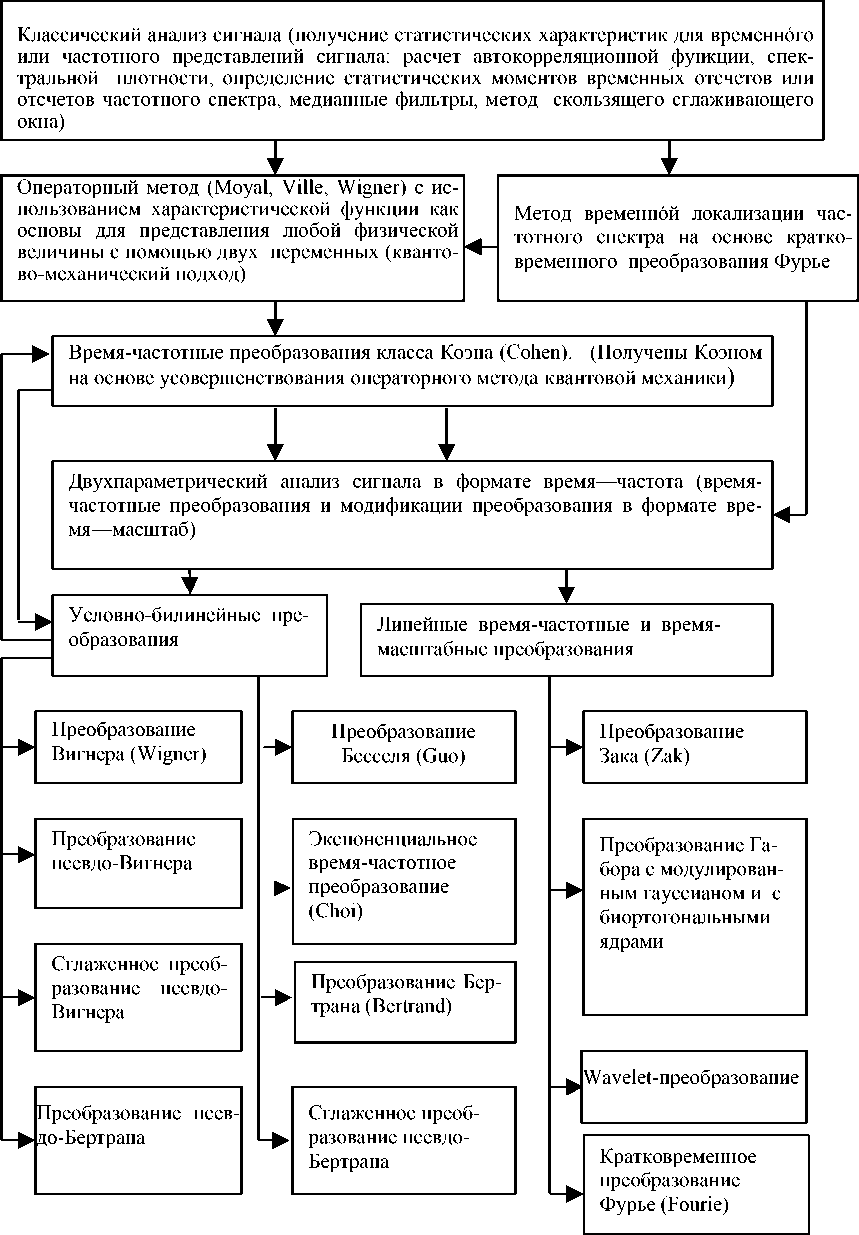
Рис. 3. Классификация время-частотных преобразований
Кроме того, группа билинейных преобразований — преобразования Бертрана и его модификаций (псевдо- и сглаженного псевдо-Бертрана) включает масштабирование времени. Это преобразование — и билинейное, и время-масштабное.
К классу линейных время-масштабных преобразований относятся все виды вейвлет-преобразований, включая их разновидность — преобразование на основе сплайн-базиса. В классификацию ВЧП они внесены на том основании, что параметр масштаба a вейвлет-преобразования (обычно а = 2 – j для j -уровня вейвлет-разложения сигнала) связан с частотой соотношением а = с/ω . Так что распределение сигнала в плоскости время—масштаб является одновременно распределением в плоскости время—частота при гиперболической деформации оси частот (т. е. для частоты в шкале ω = c/a ) .
ЗАКЛЮЧЕНИЕ
Таким образом, на основе проведенного анализа время-частотных преобразований можно сделать ряд выводов.
-
1) Линейные время-частотные преобразования типа Габора, КПФ и Зака не обладают хорошими свойствами локализации по частоте и по времени одновременно. Как правило, хорошее разрешение по частоте сопровождается ухудшением временнóй локализации, а повышение времен-нóго разрешения снижает частотное разрешение.
-
2) Билинейные преобразования имеют высокие характеристики локализации во время-частотной плоскости, но обладают свойством интерференции.
-
3) Устранение интерференции, осуществленное в модифицированных билинейных преобразованиях Вигнера и Бертрана путем введения сглаживания во временнóй и в частотной областях, ухудшает разрешение по времени и по частоте. Это в определенной степени снижает их преимущества и перспективу использования в исследовании.
-
4) Вычислительная сложность и отсутствие быстрых алгоритмов, основанных на методах билинейных преобразований, не позволяют использовать их при обработке сигналов в реальном времени. Фактор сложности в наибольшей степени относится к преобразованиям группы Бертрана.
ПРИЛОЖЕНИЕ . Общая сводка время-частотных преобразований и их аналитических выражений
G s ( t , f ) = J s T ) h ( т - t ) • exp — jj
dт, h (т -t) = 1 exp aVn
-
2 т - t A
a
,
s ( т ) = ;1 J J G s ( t , f ) h ( т - t ) • exP jf f T - t 2nJJ LI 2
d t d f ,
(П1)
a — параметр.
Кратковременное преобразование Фурье ( h ( t ) — любое нормированное окно)
S s ( тот ) =
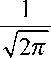
J s ( t ) h(t - т ) • e jmt d t,
s (t ) = JJ S s ( to , T ) h (t - т ) • ejmT d T d ^ .
(П2)
TO
S' s ( т , ю ) = У s ( ^ ( T + k )) • exp( jk to ), k =-to
1. ( t A s ( t ) = Xх ' f Ss | — , to | d to .
0 I X )
(П3)
Преобразование Вигнера и преобразование на основе двойственной функции
Ws (t, to) = J s(t + т /2) • s(t - т /2) • e- jtoT dт,(П4)
^
A(t,to) = Js(t + т/2) • s *(t -т/2) • e-jMdt.(П5)
-^
Преобразование псевдо-Вигнера
PW(t,to) = Js(t + т /2) • s(t - т /2) • h(т) • e-j'rdr(П6)
или, если S s ( t , to ) — преобразование Фурье сигнала с окном g ( т )=[ h (2 т )] 1/2 , то преобразование дает
PW (t,to) = 2J Ss (t,to + v) • Ss (t,to - v)dto.
Сглаженное преобразование псевдо-Вигнера
PWs (t, to) = 2J Gv) • Ss (t, to + v) • S* (t, to - v)dv, где G(v) — функция окна по частоте.
Обобщенное время-частотное преобразование класса Коэна
G ( t to ,®)=± jjj
ej i -тto-f t )
• Ф( ^ , т ) • s ( ц + т /2) • s ( ц - т / 2)d ц d т d f .
(П9)
Преобразование Бертрана
B s ( t , to ) = J [< Л ( u ) • to ) 1/2 J s ( т ) • e - jX ( u ) to ( т - t ) d т ] • |я( - u ) to ) 1/2 J s ( т ') e - jX ( - u ) to ( т" t ) d т 'f d u .
Более общая форма преобразования Бертрана
B s ( t , to ) = to J ц ( u ) • s ( Я ( u ) • to ) • s * ( Я ( - u ) • to ) • exp jf ( u ) tto d u ,
Я ( u ) = — e
- u
- u - 1
,
i ( u ) = Я ( u ) - Я ( - u ), ц ( u ) = [ Я ( u ) • Я ( - u )] 1/2 f d ^ u )
( d u J
(П10)
.
Преобразование псевдо-Бертрана
PB s ( t , to ) =
= J - [ ^ ( u ) to ] 1/2 [ Я ( - u ) to ]
1/2 • [J s ( т ) • ^ ( Я ( u ); to ( т - t wL [j s ( т ') • i/r( Я ( - u ); to ( т '- 1 ))d т ']* j • d u ,
(П11)
у(X(и);to(T -t)) : = h [Л(и) • to(T -t)] • ejX(u)m(T t), h — окно сглаживания по частоте(П12)
(читается: ψ (…) по определению равно h (…)).
Преобразование псевдо-Бертрана, выраженное через вейвлет-преобразование сигнала
PBs (t, to) = J Ds (t, X( и )to) • D* ( t, X( - и )to )d и,(П13)
D s — вейвлет-преобразование от сигнала s с функцией ^ ( T ) = h( T )-exp(2 n j T ).
Сглаженное преобразование псевдо-Бертрана
PBs (t, to) = J G (и). Ds (t, toX (и)) • Ds (t, toX (- и ))d и,(П14)
Ds (t,to) = to1/2 J s(t)y *(to(T -1))dT.(П15)
BD s ( t , to ) = J e - jtoT
T
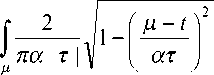
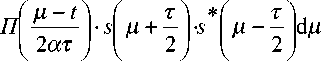
• d T ,
(П16)
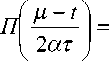
1,
0,
если | ц - t |< ат, иначе,
(П17)
а — параметр масштаба, входит в ядро Коэна с Бесселевой функцией Ф ( ^ , т ) =
J 1 (2 n a ^T ) л а ^т
Список литературы Класcы преобразований нестационарного сигнала в информационно-измерительных системах. II. Время-частотные преобразования
- Меркушева А.В. Классы преобразований нестационарного сигнала в информационно-измерительных системах. I. Элементы теории//В этом номере.
- Claasen T.A.C., Mecklenbrauker W.F.G. Wigner distribution//Philips journal on research. 1980. V. 35, parts 1, 2, 3. P. 217-250, 276-300, 372-389.
- Cohen L. Quantization Problem and Variational Principle in Quantum Mechanics//Journal of Mathematical Physics. 1976. V. 17. P. 1863-1866.
- Cohen L. Time-frequency Distribution//Proceedings of IEEE. 1989. V. 77, N 7. P. 941-981.
- Daubechies I. Orthogonal Bases of Compactly Supported Wavelets//Communications in Pure and Applied Mathematics. 1988. V. 41, N 7. P. 909-996.
- Daubechies I. Ten Lectures on Wavelets//Conference Board of Mathematical Sciences. Society of Industrial and Applied Mathematics (SIAM), 1991. P. 258.
- Grossman A., Morlet J. Decompositions of Hardy Functions into Square Integrand Wavelets of Constant Shape//SIAM Journal of Mathematical Analysis. 1984. V. 15. P. 723.
- Heil G.E., Walnut D.F. Continuous and Discrete Wavelet Transforms//SIAM Review. 1989. V. 31, N 12. P. 628.
- Lamarie P.G. Ondelettes a Localization Exponentiale//Journal Mathematics Pure Applied. 1988. V. 67. P. 227-336.
- Mallat S.G. Multifrequency Chanal Decompositions of Image and Wavelet Models//IEEE Transactions on Acoustic, Speech and Signal Processing. 1989. V. 37, N 12. P. 2091.
- Wigner E.P. On Quantum Correction for Thermodynamic Equilibrium//Physical Review. 1932. V. 40. P. 749-759.
- Janssen A.J.E.M. On the Locus and Spread of Pseudo Density Functions in the Time-Frequency Plane//Phillips Journal of Researchs. 1982 V. 37, N 3. P. 79.
- Bastiaans M.J. Decomposition of Signals in Gabor Series of Elementary Gaussian Signals//Proceedings of IEEE. 1980. V. 68, N 4. P. 123.
- Gabor D. Theory of Communication//Journal of Institute for Electrical Engineering. 1946. V. 93, N 11. P. 429.
- Qian S., Chen D. Discrete Gabor Transform//IEEE Transactions on Signal Processing. 1993. V. 41, N 7. P. 2429.
- Qian S., Chen D. Signal Representation Using the Gaussian Functions//Signal Processing. 1994. V. 36. P. 1-11.
- Allen J.B., Rabiner L.R. A Unified Approach to Short-Time Fourier Analyses and Synthesis//Proceedings of IEEE. 1977. V. 65, N 11. P. 1558.
- Portnoff M.R. Time-Frequency Representation of Digital Signals and Systems Based on Short-Time Fourier Analyses//IEEE Transactions on Signal Processing. 1980. V. 28, N 2. P. 55.
- Auslander I., Gertner I.C., Tolimieri R. The Discrete Zak Transform Application to Time-Frequensy Analysis and Synthesis of Nonstationary Signals//IEEE Transactions on Signal Processing. 1991. V. 39, N 4. P. 825.
- Janssen A.J.E.M. The Zak Transformations. A Signal Transformations for Sampled Time-Continuous Systems//Phillips Journal of Research. 1988. V. 43. P. 23.
- Daubechies I., Jaffard S., Journe J.L. A Simple Wilson Orthonormal Basis with Exponential Decay//SIAM Journal of Mathematical Analysis. 1991. V. 22, N 2. P. 554.
- Loughlin P.J., Pitton J.W., Atlas L.E. Bilinear Time-Frequency Transformation. New Insight and Properties//IEEE Transactions on Signal Processing. 1993. V. 41, N 5. P. 750.
- Gaunaurd G.C., Strifors H.C. Signal Analysis by Means of Time-Frequency Transformation оf Wigner Type//Proceedings of IEEE. 1996. V. 84, N 9. P. 1231-1247.
- Hlawatsch F., Boudreaux-Bartels G.F. Linear and Quadratic Time-Frequency Signal Representations//IEEE Signal Processing Magazine. 1992. V. 9, N 4. P. 21-67.
- Consalves P., Baraniuk R.G. Bertran's Bilinear Time-Frequency Transformation and its Modifications//Signal Processing Letters. 1996. V. 3, N 3. P. 82.
- Auger F., Flandrin P. Improvement of Readability of Time-Frequency Representation by Reassignment Method//IEEE Transactions on Signal Processing. 1993. V. 43, N 5. P. 1068.
- Loughlin P. Non Negative Time-Frequency Representation//IEEE Transactions on Signal Processing. 1994. V. 42, N 10. P. 2697.
- Choi H.I., Williams W.J. Exponential Kernel with Reduced Interference on the Basis of Exponential Kernel//Proceedings of IEEE. 1996. V. 84, N 9. P. 1264.
- Bertrand J., Bertrand P. A Class of Wigner Functions with Extended Covariance Properties//Journal of Mathematical Physics. 1992. V. 33, N 7. P. 2515.
- Guo Z., Durand L. Time-Frequency Transformation with Bessel function as a Kernel//IEEE Transactions on Signal Processing. 1994. V. 42, N 7. P. 1700.
- Choi H., Williams W.J. Improved Time-Frequency Representation with Exponential Kernels//IEEE Transactions on Signal Processing. 1989. V. 37, N 6. P. 862-871.
- Choi H.I., Williams W.J., Zaveri H. Analysis of Event Related to Potentials: Time-Frequency Energy Distribution//Biomedical Science Instrument. 1987. V. 23. P. 251.
- Stankovic L.J. Time-Frequency Distribution Concentrated Along the Instantaneous Frequency//IEEE Signal Processing Letters. 1996. V. 3, N 3. P. 89.
- Stankovic L.J. Improved Concentration in Time-Frequency Transformation for Using L-Wigner-Distribution//IEEE Transactions on Signal Processing. 1995. V. 43, N 5. P. 1263.
- Ville J. Theory et Applications de la Notion de Signal Analitique//Cables et Transmission. 1948. V. 24. P. 61-74.
- Morris J.M., Lu Y. Discrete Gabor Expansion of Discrete-Time Signal via Frame Theory//Signal Processing. 1994. V. 40, N 2. P. 155.
- Duffin R.J., Schaeffer A.C. A Class of Nonparametric Fourier Series//Transactions of American Mathematical Society. 1952. V. 72. P. 341.


