Конечно-элементная оценка деформированного состояния по данным компьютерной томографии
Автор: Воробьв О.В., Семнова Е.В., Мухин Д.А., Стаценко Е.О., Балтина Т.В., Герасимов О.В.
Статья в выпуске: 2, 2021 года.
Бесплатный доступ
В статье представлен один из возможных подходов к моделированию объектов с анизотропными свойствами на основе изображений исследуемой области. Данные с таких изображений учитываются при построении численной модели. В этом случае неоднородность материала может быть включена за счет интегрирования локальной матрицы жесткости каждого конечного элемента с некоторой весовой функцией. Цель исследования заключается в разработке конечного элемента для формирования расчетного ансамбля и моделирования механического поведения с учетом данных двумерных медицинских изображений. Для реализации предложенного подхода использовалось предположение о наличии связи между значениями в пикселях изображений и упругими свойствами материала. Построение сетки базировалось на применении четырехузлового плоского конечного элемента. Подобный подход позволяет использовать количественные фазовые или сканирующие электронные изображения, а также данные компьютерной томографии. Произведен расчет ряда тестовых задач на сжатие образцов элементарной геометрии. В качестве модельной задачи была рассмотрена дистальная часть бедренной кости крысы. Срез компьютерной томографии образца использовался при построении численной модели с учетом неоднородности распределения материала внутри органа. Представлено поле распределения узловых перемещений, основанное на данных, полученных с изображений исследуемой области. В рамках модельной задачи был рассмотрен вопрос влияния разрешающей способности компьютерного томографа на качество получаемых результатов. С этой целью были проведены расчеты на основе сжатых входных медицинских изображений.
Конечно-элементное моделирование, цифровой прототип, компьютерная томография, негомогенные структуры, костная ткань
Короткий адрес: https://sciup.org/146282052
IDR: 146282052 | УДК: 539.37 | DOI: 10.15593/perm.mech/2021.2.05
Текст научной статьи Конечно-элементная оценка деформированного состояния по данным компьютерной томографии
ВЕСТНИК ПНИПУ. МЕХАНИКА № 2, 2021PNRPU MECHANICS BULLETIN
В настоящее время одной из актуальных проблем клинической практики выступает оценка напряженно-деформированного состояния органов при проведении хирургического вмешательства. Первоначально на основе параметрической цифровой модели органа производится локальная оценка прочности кости после установки имплантата, в том числе при различных возможных патологиях [1–3]. Необходимость такой оценки обеспечивается в том числе физиологическими процессами, возникающими в костной ткани под действием силовых факторов. Происходит так называемая перестройка костной ткани, что влечет за собой изменения структурных свойств исследуемых органов. Этот эффект неоднократно был замечен в рамках экспериментов in vivo на животных [4–6]. Существуют подходы к моделированию таких процессов. Разрабатываемые модели позволяют определить архитектуру костной ткани после перестройки на основе численных расчетов [7–9]. Необходимость учета структурных свойств органов продиктована особенностями локальной прочности. Один из подходов оценки механических свойств костной ткани основан на гомогенизации исследуемой области с применением различных методов. Одним из распространенных подходов является метод представительного объемного элемента [10, 11]. Такой способ позволяет учитывать в том числе и нелинейность материала [12]. Применение данных компьютерной томографии объекта для построения представительного объемного элемента расширило возможности ее использования [12, 13]. При этом стоит отметить трудозатратность такого метода.
К другому подходу гомогенизации можно отнести тензор структуры, определяющий основные направления распределения свойств материала [14, 15]. Существующие физические соотношения позволяют использовать тензор структуры в расчетах [16]. В первую очередь получили большое распространение вопросы гомогенизации области расчета путем введения эффективных свойств [17–19]. В данном случае некоторой нетривиальной геометрии ставится в соответствие упрощенная область с усредненными параметрами, позволяющая производить расчет с приближенными характеристиками объекта. К другому способу моделирования можно отнести построение негомогенной модели на основе вычисления локальных свойств для каждого представительного объема в отдельности [20–22]. Данный подход обладает существенным недостатком в виде ресурсоем-кости в силу необходимости проведения колоссального количества расчетов. Можно отметить обособленный подход к моделированию гетерогенных сред, в рамках которого формирование конечно-элементного ансамбля осуществляется на основе данных компьютерной томографии. Учет неоднородного распределения свойств при таком подходе осуществляется при формировании расчетных матриц для метода конечных объемов [23]. Отдельно были рассмотрены задачи построения расчетной сетки по данным компьютерной томографии [24– 25]. Обобщение вышеуказанных методик было произведено в работе [26] для персонализированных расчетов по данным медицинских изображений. Предлагаемый подход учета данных компьютерной томографии в трехмерной постановке был реализован на основе метода конечных элементов [27]. В этой же работе приведена оценка влияния плотности заполнения вокселями конечноэлементного ансамбля на результаты расчетов.
Цель данного исследования – разработка конечного элемента для формирования расчетного ансамбля и моделирования механического поведения с учетом данных двумерных медицинских изображений.
1. Материалы и методы
Исследование вводит в рассмотрение методологию моделирования механического поведения системы, принадлежащей некоторому пространству сплошного материала Ω и соответствующему ему дискретному пространству Ω'. Исследуемый объект площади S лежит в Ω из R 2. Данные компьютерной томографии, соответствующие объекту, определяются пространством Ω' и состоят из микрообластей со значениями рентгеновской плотности, которые могут быть визуализированы с использованием цветового спектра. Данные объекта дискретной области S' из Ω' могут быть получены путем разделения площади S на большое число микроподобластей (пикселей), размер которых зависит от разрешающей способности измерительного устройства. Подразумевается, что объект области S состоит из материала и пор. Первым предположением выступает наличие связи между значениями в пикселях изображения (цифрового прототипа) и упругими свойствами материала. Вторым предположением полагается, что материал сплошной и изотропный, а анизотропию на макроуровне определяет наличие и распределение пор. На практике к таким изображениям могут быть отнесены изображения, получаемые на основе рентгеновской томографии. Так, данные компьютерной томографии могут быть использованы при определении локальных механических параметров. В этом случае необходимо понимать взаимосвязь между механическими параметрами и значениями, полученными из шкалы Хаунсфил-да. На рис. 1 представлено пространство сплошного материала Ω и связанное с ним дискретное пространство Ω'.
from images
Определим основные возможности предложенного подхода в случае плоских деформаций. Рассмотрим принцип реализации конечного элемента неоднородной среды. За основу был взят известный метод построения четырехузлового плоского изопараметрического конечного элемента с линейной аппроксимацией геометрии и поля перемещений. Использовались следующие функции для аппроксимации радиус-вектора r и вектора перемещений ϑ :
x4
r = {r} = i r=Ei n rNn C^,n),(1)
L y n= 1 I y n
u
9 = H = ] Г = E nrNn (4,n),(2)
Lv«=1 L Vn где x и y – вектор координат в глобальной декартовой системе координат; u и v – вектор перемещений в глобальной декартовой системе координат; n – локальный номер узла конечного элемента; Nn(ξ, η) = (1 + ξnξ)∙(1 + + ηnη)/4 – функции формы, ξn и ηn – локальные координаты узлов элемента, ξ и η – координаты в локальной системе координат.
Данные соотношения могут быть переписаны в матричной форме:
{ 9 } = [ N ] - { 9 e } , (3)
где [ N ] – матрица аппроксимирующих функций; ϑ e – вектор узловых перемещений.
Деформация среды может быть описана с помощью компонент линейных и сдвиговых деформаций, представленных в виде приведенного вектора ε и выраженных через перемещения с помощью известных соотношений вида
ε xx
d u
—, £ dx yy
dv du
—, £„, = — +— dy dy
ε = ε = ε = 0 zz yz zx ,
которые также могут быть представлены в матричном виде:

а
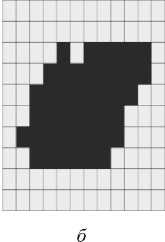
Рис. 1. Схематическое представление исходного объекта: а – пространство сплошного материала Ω; б – дискретное пространство соответствующих данных с изображений Ω'
Fig. 1. A schematic representation of the original object: ( a ) solid material space Ω; ( b ) discrete space Ω' of the corresponding data
{ £ } = [ B ] - {9} . (5)
Напряженное состояние определяется тензором напряжений через компоненты главных напряжений σ xx , σ yy , σ zz , т.е. нормальных для случая, когда тангенциальные напряжения σ xy = σ xz = σ zy равны нулю. Здесь под осями x – y понимаются главные оси тензора напряжений. Тензор напряжений, подобно тензору упругих деформаций, может быть записан в виде приведенного вектора σ . Закон Гука, связывающий приведенные векторы напряжений и деформаций, может быть представлен в следующем виде:
{ O } = [ D ( Г ) ]■ { £ } . (6)
Для вычисления локальной матрицы жесткости использовались известные соотношения вида [28]
[ K ] = J [ B ( r ) ] T •[ D ( r ) ]•[ B ( r ) ] d , (7)
Ve где Ve – объем конечного элемента в пространстве конечно-элементной сетки Ωe; [D(r)] – тензор упругости; [B(r)] – матрица, связывающая приведенный вектор упругих деформаций и вектор узловых перемещений:
{ 8 } - [ B ( Г ) ]• { & e } . (8)
С применением гипотезы об анизотропии, возникающей вследствие наличия пор, матрица жесткости конечного элемента относительно дискретного пространства Ω' может быть вычислена с помощью следующего соотношения:
[ K e ] = J [ B ( r ) ] T . [ D ] •[ B ( r ) ]. Ю ( r ) dV e , (9)
Ve где ω(r) – функция Ω→Ω' и [D] – тензор упругости изотропного материала, который может быть выражен через модуль упругости Юнга и коэффициент Пуассона.
В следующих уравнениях будет опущено интегрирование в направлении оси z . В случае перехода к локальным координатам уравнение (9) может быть переписано в виде
[ K e ] = h - J J [ B ( S,n ) ] T • [ D ] •[ B ( S,n ) ]' J ( S,n ) ' ® ( S,n ) d S d П, (10) - 1 - 1
где | J (ξ, η)| – определитель якобиана преобразования координат; he – размер элемента в направлении оси z .
Численное интегрирование локальной матрицы жесткости проводилось с использованием метода средних прямоугольников. В качестве конечного элемента неоднородной среды был выбран выпуклый четырехугольник с линейными ребрами. Точки интегрирования для такого элемента представляют собой локальные координаты геометрических центров пикселей входного изображения, таким образом:
[ Ke ] = h-2Z[ B ( Si,n)] T •[ D ]•[ B (Si ,n)]x i e I j e J xJ (S i ,n j )• ^(S i ,n j )ASAn, (11)
где ξ i и η i – локальные координаты точек интегрирования; ∆ξ и ∆η – шаг интегрирования в направлении двух локальных координатных осей; ω(ξ i , η i ) – веса квадратурной формулы, определяемые значениями с изображений в текущей точке интегрирования; I и J – количество квадратурных точек в направлении двух локальных координатных осей в элементе.
Для определения принадлежности пикселя области конечного элемента было использовано следующее неравенство:
где r A – радиус-вектор точки A (центр пикселя); r I – радиус-вектор точки I ; n i – вектор нормали к грани i ( i от 1 до 4); δ – параметр точности.
На рис. 2 представлены численные данные ( а ) и геометрия конечного элемента ( б ), наложенная на дискретное пространство компьютерной томографии ( I , J , K , L – узлы конечного элемента). Если пиксель полностью принадлежит области конечного элемента, параметр точности δ определяется нулевым значением. В процессе интегрирования возникают некоторые трудности вследствие внутренней дискретности пространства данных со входных изображений.

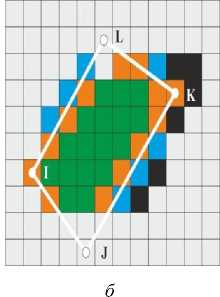
Рис. 2. Схематический вид исследуемой области: а – бинаризованная область S' дискретного пространства компьютерной томографии Ω'; б – пространство конечно-элементной сетки Ωe, наложенное на дискретное пространство Ω' (зеленым цветом отмечены пиксели, полностью принадлежащие внутренней области конечного элемента, оранжевым цветом обозначены пиксели, принадлежащие области относительно их геометрического центра, синим цветом определены пиксели, касающиеся границы конечного элемента некоторой окрестностью геометрического центра, черным цветом – пиксели, лежащие вне интегрируемой области)
Fig. 2. A schematic view of the investigated area: ( a ) binarized area S' of the discrete space Ω' of the computed tomography; ( b ) the space of the finite element mesh Ωe superimposed on the discrete space Ω' (the pixels that completely belong to the inner region of the finite element are marked in green, the pixels belonging to the area relative to their geometric center are marked in orange, the pixels touching the boundary of the finite element by some neighborhood of the geometric center are defined in blue;
in black one can see pixels lying outside the integrated area)
Существует несколько способов определения вхождения пикселя в область конечного элемента – в этом случае рассматривается некоторая окрестность точки интегрирования. На рис. 2, б представлены варианты со следующими условиями принадлежности области элемента: 1) геометрический центр пикселя лежит внутри области (зеленый и оранжевый цвета); 2) некоторая окрестность пикселя имеет ненулевое пересечение с областью элемента (оранжевый и синий цвета).
В силу предположения о разрывности значений напряжений в узлах элементов необходимо провести анализ напряжений, основанный на локальном усредне- нии напряженного состоянии по объему каждого конечного элемента. Вектор усредненных напряжений может быть найден с помощью выражения [28, 29]
о - 1 Jo dV , (13)
VV где V – объем конечного элемента в пространстве сплошного материала Ω; V' – объем в дискретном пространстве компьютерной томографии Ω'.
Для сегментации исследуемого объекта на изображении использовались методы, основанные на оптической плотности [30, 31] с модификациями, описанными в [32, 33]. Для автоматизации построения расчетной сетки использовался подход фильтрации сетки, описанный в [34]. Оценка влияния плотности заполнения конечного элемента пикселями на точность результата подробнее представлена в работе [27]. Весовая функция может быть определена в зависимости от типа входных двумерных изображений. Так, для данных томографии существуют формулы для пересчета оптической плотности и механических характеристик (модуль Юнга, предел прочности) [17, 20].
3. Результаты и обсуждение 3.1. Тестовые задачи
Для оценки сходимости предложенного подхода были рассмотрены тестовые задачи на основе образцов прямоугольной геометрии. Алгоритм был реализован в системе компьютерной алгебры Wolfram Mathematica . Сравнение результатов было проведено в соответствии с данными, полученными в универсальном программном комплексе конечно-элементного анализа ANSYS.
Таблица 1
Геометрические и физические параметры модели
В первой тестовой задаче рассматривался образец с квадратной геометрией, состоящий из сплошного изотропного материала. Размер массива данных компьютерной томографии составлял 100×100 пикселей. Прикладываемая к верхней грани равномерно распределенная нагрузка равнялась 3000 Н. Нижняя грань фиксировалась в перемещениях в направлении двух координатных осей. Для сравнения результатов было произведено моделирование в системе ANSYS образцов с двумя типами сеток, состоящих из 1 и 10 000 конечных элементов соответственно. Результаты сравнивались с данными модели, построенной на основе предложенного подхода с использованием 1, 4 и 9 конечных элементов.
Таблица 2
Результаты численного расчета тестовой задачи: средние перемещения в нагруженных узлах вдоль вертикальной оси
Table 2
Results of numerical calculations of the test problem: average displacements in loaded nodes along the vertical axis
|
ANSYS, 1 КЭ |
ANSYS, 10 000 КЭ |
1 КЭ |
4 КЭ |
|
|
∆ y , мм |
–1,4553 |
–1,48171 |
–1,4553 |
–1,4714 |
Исходя из предположения о непрерывности материала, весовые функции принимали значения, равные единице. Количество пикселей, принадлежащих области одного конечного элемента, варьировалось в зависимости от его размера. Численное интегрирование локальной матрицы жесткости осуществлялось на основе стандартного метода интегрирования средними прямоугольниками. В связи с этим ошибка результатов была связана с ошибкой метода интегрирования и способа усреднения значений по верхнему краю (табл. 2).
Table 1
Geometric and physical parameters of the model
|
a , мм |
b , мм |
h , мм |
E , ГПа |
ν |
|
10,0 |
10,0 |
0,1 |
20 |
0,3 |
В табл. 1 приведены геометрические и физические параметры тестовых задач: a и b – размеры геометрии тестового образца вдоль горизонтальной ( Ox ) и вертикальной ( Oy ) осей соответственно, h – толщина в направлении нормали к плоскости образца ( Oz ), E – модуль упругости Юнга, ν – коэффициент Пуассона. Для сравнения полученных результатов были рассмотрены перемещения в направлении оси Oy в узлах нагруженной линии.
3.2. Сплошной образец

б
Рис. 3. Деформированное состояние сплошного образца: а – геометрия модели с граничными условиями;
б , в , г – 1, 4 и 9 КЭ соответственно
г
Fig. 3. The deformed state of a solid specimen: ( a ) a model geometry with boundary conditions; ( b ), ( c ) and ( d ) are 1, 4 and 9 FEs, respectively
На рис. 3 представлено деформированное состояние образца в зависимости от числа конечных элементов.

Рис. 4. Поле узловых перемещений вдоль вертикальной оси (10 000 КЭ, ANSYS )
Fig. 4. The field of nodal displacements along the vertical axis (10 000 FEs, ANSYS )
Рис. 4 отображает деформированное состояние вдоль вертикальной оси, вычисленное в системе ANSYS. Свойства материала определяют симметрию в распределении полученных значений поля деформаций.
-
3.3. Образец с треугольным вырезом
В следующем эксперименте рассматривался образец 100×100 пикселей с треугольным вырезом, определяющим остаточную рамку шириной 0,1 мм. В этом случае весовая функция принимала значения двух видов (0 и 1), в зависимости от локальных координат текущей точки интегрирования. Параметры модели аналогичны постановке первой тестовой задачи, прикладываемая нагрузка соответствовала 300 Н.
Рис. 5. Образец с треугольным вырезом: а – геометрия выреза; б , в , г – деформированное состояние модели из 1, 4 и 9 КЭ соответственно
Fig. 5. A sample with a triangular cut: ( a ) a cut geometry; ( b ), ( c ) and ( d ) is the deformed state of the model with 1, 4 and 9 FEs, respectively
Полость, образованная надрезом, влияет на податливость образца к внешней нагрузке, что определяется повышенной деформацией геометрии рассматриваемой области (рис. 5, б – г ). Следует отметить, что при небольшом количестве конечных элементов аппроксимация геометрии образца в деформированном состоянии является угловатой (рис. 6). В связи с этим было предложено рассматривать модели, построенные по данным компьютерной томографии с использованием 100 и 400 конечных элементов соответственно. В этом случае модуль Юнга был равен 200 ГПа, а приложенная нагрузка – 20 Н. Для автоматизации построения такой расчетной сетки использовался подход фильтрации сетки [34].
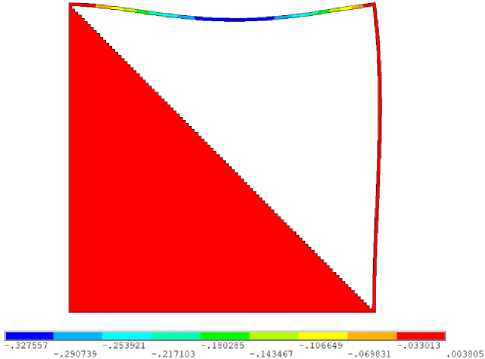
Рис. 6. Поле узловых перемещений вдоль вертикальной оси (10 000 КЭ, ANSYS )
Fig.6. The field of nodal displacements along the vertical axis (10 000 FEs, ANSYS )


в
При построении численных моделей учитывался тот факт, что конечные элементы, целиком принадлежащие областям, в которых нет материала (значения в пикселях равны нулю), определяются нулевой локальной матрицей жесткости. При отсутствии связи в узлах с элементами, соответствующими ненулевой матрице жесткости, это приводит к вырождению глобальной матрицы жесткости. Таким образом, подобные «пустые» элементы заранее исключались из сетки (рис. 7, а , б ).
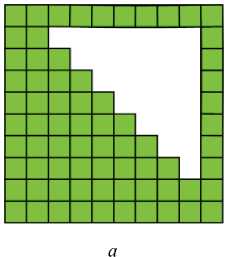
Рис. 7. Деформированное состояние модели: а – 100 КЭ, б – 400 КЭ

Fig. 7. The deformed state of the model: ( a ) 100 FE, ( b ) 400 FE
-
3.4. Образец с неравномерной плотностью
В следующей тестовой задаче рассматривалось моделирование области с неравномерным распределением плотности материала. Законы распределения неоднородности взяты из работы [26]. Граничные условия задачи и механические параметры аналогичны предыдущим постановкам тестовых задач. На рис. 8, а приведено изображение, определяемое функцией
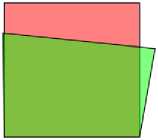
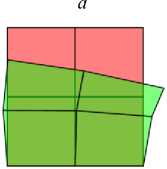
Ю( i, j) - (j +10)+(i +10)2, а на рис. 9, а – функцией to (i, j) = (1 + 0,7sin (2n i) sin (2nj'))(j +10) + (i +10)2, где i и j – индексы пикселя в матрице изображения.

в

Рис. 8. Первый образец с неравномерной плотностью: а – первый тип распределения плотности; б , в , г – деформированное состояние модели с 1, 4 и 9 КЭ соответственно
Fig. 8. The first sample with an uneven density: ( a ) the first type of density distribution; ( b ), ( c ) and ( d ) the deformed state of the model with 1, 4 and 9 FEs, respectively

Рис. 9. Второй образец с неравномерной плотностью: а – второй тип распределения плотности; б , в , г – деформированное состояние модели с 1, 4 и 9 КЭ соответственно
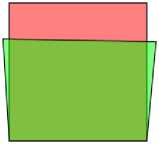
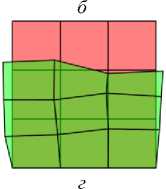
Fig. 9. The second sample with the uneven density: ( a ) the second type of density distribution; ( b ), ( c ) and ( d ) the deformed state of the model with 1, 4 and 9 FEs, respectively
Исходя из характера деформации, можно сделать вывод, что модель, построенная по данным со входных изображений, способна описывать поведение анизотропного материала с достаточной точностью путем учета неоднородности при интегрировании матрицы жесткости каждого конечного элемента. Характер деформации геометрии моделей с анизотропными свойствами (рис. 8, б–г , 9, б–г ) существенно отличается от результатов, полученных с использованием моделей, основанных на предположении об изотропии материала (см. рис. 4, б–г ). При этом снижается требование к количеству элементов, а анизотропность среды учитывается за счет интегрирования локальных матриц жесткости.
3.5. Модельная задача
В постановке модельной задачи использовалось изображение среза дистального отдела бедренной кости крысы, полученное с использованием данных компьютерной томографии. Разрешение исходного изображения составляло 996×795 пикселей (рис. 10, а ). Для оценки влияния разрешающей способности сканирующего устройства на качество получаемых результатов исходное изображение было сжато с потерей качества. Таким образом, проводились расчеты также на аналогичных изображениях, но с уменьшенным разрешением – 332×265 и 166×132 пикселей соответственно.
Таблица 3
Геометрические и механические параметры образца модельной задачи
Geometric and mechanical parameters of the model problem sample
Table 3
|
a , мм |
b , мм |
h , мм |
E , ГПа |
ν |
|
8,79 |
11,01 |
0,1 |
12 |
0,3 |
Механические и геометрические параметры испытания представлены в табл. 3: a и b – размеры геометрии образца модельной задачи вдоль горизонтальной и вертикальной осей Ox и Oy соответственно, h – толщина в направлении ортогональной к плоскости образца оси Oz , E – модуль упругости Юнга, ν – коэффициент Пуассона. Сравнение полученных результатов осуществлялось на основе значений перемещений в направлении вертикальной оси Oy в узлах верхней линии (рис. 11 красным цветом обозначена точка, для которой производилось сравнение полученных величин).
Геометрия образца была аппроксимирована 70 конечными элементами (7 элементов по горизонтальной оси и 10 – по вертикальной, рис. 10, б ). Рассматриваемый образец подвергался воздействию одноосной продольной сжимающей вертикальной нагрузки, равномерно распределённой по верхним узлам и равной 100 Н. Величина нагрузки была выбрана на основе проводимых экспериментальных исследований. Узлы, принадлежащие нижней линии, фиксировались в перемещениях вдоль направления двух координатных осей (Ox и Oy). Для предотвращения вырождения глобальной матрицы жёсткости из сетки заранее исключались конечные элементы, соответствующие нулевой плотности, определяющей отсутствие материала (рис. 10, в ).
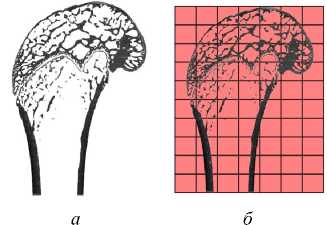
Рис. 10. Дистальная часть бедренной кости крысы: а – компьютерная томография среза; б – конечно-элементная сетка, наложенная на изображение; в – сетка с граничными условиями после удаления элементов с нулевой плотностью материала

Fig. 10. The distal part of the rat femur: ( a ) the computed tomography of the section; ( b ) the finite element mesh superimposed on the image; ( c ) the mesh with boundary conditions after removing elements with zero material density
Полученная картина перемещений отражает асимметрию в геометрии испытуемого образца. Характер наблюдаемой деформации также объясняется неравномерной плотностью материала. Следует отметить, что разница в результатах, полученных по изображениям с различным разрешением, незначительна (рис. 11, а – в ).
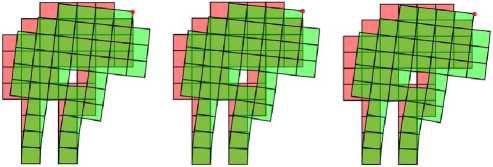
а б в
Рис. 11. Деформированное состояние модели: а – 996×795;
б – 332×265; в – 166×132 пикселей
Fig. 11. The deformed state of the model: (a) 996×795, (b) 332×265, (c) 166×132 pixels
Незначительное различие результатов свидетельствует о возможности уменьшения количества точек интегрирования вследствие ослабления разрешения компьютерной томографии (табл. 4).
Подобная процедура может уменьшить объем вычислений без значительного увеличения погрешности результатов.
Таблица 4
Перемещения красной точки вдоль горизонтальной и вертикальной осей
Table 4
Displacements of the red point along the horizontal and vertical axes
|
996×795 |
332×265 |
166×132 |
|
|
∆ x , мм |
0,126686 |
0,126512 |
0,136352 |
|
∆ y , мм |
–0,060812 |
–0,060795 |
–0,066001 |
Заключение
В статье представлен метод построения плоского конечного элемента, способного учитывать неравномерное распределение свойств материала на основе изображений исследуемой области. Такой подход позволяет производить построение сетки конечных элементов на основе связи между значениями в пикселях и упругими свойствами материала. В статье представлено решение показательных тестовых задач, позволяющих оценить обоснованность предложенной методики. Алгоритмы численных расчетов реализованы в собственном программном обеспечении. Представленные результаты отображают данные численного эксперимента в модельной постановке: данные компьютерной томографии среза бедренной кости крысы использовались в качестве входного изображения исходной области. Полученные значения сравнивались с результатами решения соответствующих задач в системе ANSYS. Представленные деформации рассматриваемых областей показали адекватность получаемых результатов, а также указали на возможность значительного сокращения времени расчета путем разумного уменьшения разрешения исходного изображения. В дальнейшем предполагается развитие данной методики с использованием других типов конечных элементов и улучшенных методов интегрирования конечных элементов.
Исследование выполнено за счет гранта Российского научного фонда проект № 18-75-10027.
Acknowledgements
The study was supported by a grant from the Russian Science Foundation, Project No. 18-75-10027.
Список литературы Конечно-элементная оценка деформированного состояния по данным компьютерной томографии
- Natali A.N., Pavan P.G., Ruggero A.L. Evaluation of stress induced in peri-implant bone tissue by misfit in multi-implant prosthesis // Dent. Mater. Off. Publ. Acad. Dent. Mater. - 2006. -Vol. 22, no. 4. - P. 388-395. DOI: 10.1016/j.dental.2005.08.001
- Numerical study of stress-strain state of pelvis at the proximal femur rotation osteotomy / O.A. Sachenkov, R.F. Hasanov, P.S. An-dreev, Yu.G. Konoplev // Russian Journal of Biomechanics. - 2016. -Vol. 20, no. 3. - P. 220-232. DOI: 10.15593/RJBiomech/2016.3.06
- Determination of muscle effort at the proximal femur rotation osteotomy / O. Sachenkov, R. Hasanov, P. Andreev, Y. Konoplev // IOP Conf. Ser.: Mater. Sci. Eng. - 2016. -Vol. 158, no. 1, 012079.
- In vivo trabecular bone morphologic and mechanical relationship using high-resolution 3-T MRI / A. Alberich-Bayarri, L. Marti-Bonmati, R. Sanz-Requena, E. Belloch, D. Moratal // Am. J. Roentgenol. - 2008. - Vol. 191, no. 3. - P. 721-726. DOI: 10.2214/AJR.07.3528
- The influence of hindlimb unloading on bone and muscle tissues in rat model / T. Baltina, N. Ahmetov, O. Sachenkov, A. Fedyanin, I. Lavrov // BioNanoScience. - 2017. - Vol. 7, no. 1. - P. 67-69. DOI: 10.1007/s12668-016-0288-8
- Bone remodeling in the resurfaced femoral head: effect of cement mantle thickness and interface characteristic / M. Prez, P.-A. Vendittoli, M. Lavigne, N. Nuo // Med. Eng. Phys. - 2014. -Vol. 36, no. 2. - P. 185-195. DOI: 10.1016/j.medengphy.2013.10.013
- The evolution of the bone in the half-plane under the influence of external pressure / O. Gerasimov, F. Shigapova, Y. Konoplev, O. Sachenkov // IOP Conf. Ser. Mater. Sci. Eng. -2016. - Vol. 158, no. 1, 012037.
- Formation and elaboration of the classical theory of bone tissue structure description / A.A. Kichenko, V.M. Tverier, Y.I. Nyashin, E.Y. Simanovskaya, A.N. Elovikova // Russian Journal of Biomechanics. - 2008. - Vol. 12, no. 1. - P. 66-85.
- Biomechanical modelling of trabecular bone tissue in remodelling equilibrium / T.N. Chikova, A.A. Kichenko, V.M. Tverier, Y.I. Nyashin // Russian Journal of Biomechanics. - 2018. - Vol. 22, no. 3. - P. 245-253. DOI: 10.15593/RJBiomeh/2018.3.01
- Parameter determination of hereditary models of deformation of composite materials based on identification method / R.A. Kayumov, I.Z. Muhamedova, B.F. Tazyukov, F.R. Shakirzjanov // J. Phys. Conf. Ser. - 2018. - Vol. 973, no. 1, 012006. DOI: 10.1088/1742-6596/973/1/012006
- Carniel T.A., Klahr B., Fancello E.A. On multiscale boundary conditions in the computational homogenization of an RVE of tendon fascicles // J. Mech. Behav. Biomed. - 2019. -Vol. 91. - P. 131-138. DOI: 10.1016/j.jmbbm.2018.12.003
- Kayumov R.A. Structure of nonlinear elastic relationships for the highly anisotropic layer of a nonthin shell // Mech. Compos. Mater. - 1999. - Vol. 35, no. 5. - P. 409-418. DOI: 10.1007/BF02329327
- Construction of a representative model based on computed tomography / N.V. Kharin, O.V. Vorobyev, D.V. Berezhnoi, O.A. Sachenkov // PNRPU Mechanics Bulletin. - 2018. - Vol. 3. - P. 95-102. DOI: 10.15593/perm.mech/2018.3.10
- Experimental determination of the fabric tensor for cancellous bone tissue / A.A. Kichenko, V.M. Tverier, Y.I. Nyashin, A.A. Zaborskikh // Russian Journal of Biomechanics. - 2011. - Vol. 15, no. 4. - P. 66-81.
- Determination of the orthotropic parameters of a representative sample by computed tomography / N. Kharin, O. Vorob'yev, P. Bolshakov, O. Sachenkov // J. Phys. Conf. Ser. - 2019. - Vol. 1158, no. 3, 032012.
- Bone volume fraction and fabric anisotropy are better determinants of trabecular bone stiffness than other morphological variables / G. Maquer, S.N. Musy, J. Wandel, T. Gross, P.K. Zysset // J. Bone Miner. Res. - 2015. - Vol. 30, no. 6. -P. 1000-1008.DOI: 10.1002/jbmr.2437
- Micro finite element analysis of dental implants under different loading conditions / P. Martian, J. Wolff, L. Horackova, J. Kaiser, T. Zikmund, L. Borak // Comput. Biol. - 2018. -Vol. 96. - P. 157-165. DOI: 10.1016/j.compbiomed.2018.03.012
- Finite element analysis of 6 large PMMA skull reconstructions: A multi-criteria evaluation approach / A. Ridwan-Pramana, P. Marcian, L. Borak, N. Narra, T. Forouzanfar, J. Wolff // PLOS ONE. - 2017. - Vol. 12, e0179325. DOI: 10.1371/journal.pone.0179325
- A cell-based framework for numerical modeling of electrical conduction in cardiac tissue / A. Tveito, K.H. Jsger, M. Kuchta, K.-A. Mardal, M.E. Rognes // Front. Phys. - 2017. -Vol. 5, no. 48. DOI: 10.3389/fphy.2017.00048
- Building the inhomogeneous finite element model by the data of computed tomography / O.A. Sachenkov, O.V. Gerasimov, E.V. Koroleva, D.A. Mukhin, V.V. Yaikova, I.F. Akhtyamov, F.V. Shakirova, D.A. Korobeynikova, H. Chzhi // Russian Journal of Biomechanics. - 2018. - Vol. 22, no. 3. - P. 291-303. DOI: 10.15593/RJBiomeh/2018.3.05
- An x-fem and level set computational approach for image-based modelling: application to homogenization / G. Legrain, P. Cartraud, I. Perreard, N. Mos // Int. J. Numer. Methods Eng. -2011. - Vol. 86, no. 7. - P. 915-934. DOI: 10.1002/nme.3085
- Microstructural finite-element analysis of influence of bone density and histomorphometric parameters on mechanical behavior of mandibular cancellous bone structure / P. Martian, Z. Florian, L. Horackova, J. Kaiser, L. Borak // SSP. - 2017. - Vol. 258. -P. 362-365. DOI: 10.4028/www.scientific.net/SSP.258.362
- The finite cell method for bone simulations: verification and validation / M. Ruess, D. Tal, N. Trabelsi, Z. Yosibash, E. Rank // Biomech. Model. Mechanobiol. - 2012. - Vol. 11, no. 3-4. - P. 425-437. DOI: 10.1007/s10237-011-0322-2
- Efficient finite element methodology based on cartesian grids: application to structural shape optimization / E. Nadal, J.J. Rodenas, J. Albelda, M. Tur, J.E. Tarancon, F.J. Fuenmayor // Abstr. Appl. Anal. - 2013. - 953786. DOI: 10.1155/2013/953786
- Exact 3d boundary representation in finite element analysis based on Cartesian grids independent of the geometry / O. Marco, R. Sevilla, Y. Zhang, J.J. Rodenas, M. Tur // Int. J. Numer. Methods Eng. - 2015. - Vol. 103, no. 6. - P. 445-468. DOI: 10.1002/nme.4914
- Direct medical image-based Finite Element modelling for patient-specific simulation of future implants / L. Giovannelli, J.J. Rodenas, J.M. Navarro-Jimenez, M. Tur // Finite Elem. Anal. Des. - 2017. - Vol. 136. - P. 37-47. DOI: 10.1016/j.finel.2017.07.010
- Mechanical model of a heterogeneous continuum based on numerical-digital algorithm processing computer tomography data / O.V. Gerasimov, D.V. Berezhnoi, P.V. Bolshakov, E.O. Statsenko, O.A. Sachenkov // Russian Journal of Biomechanics. - 2019. - Vol. 23, no. 1. - P. 87-97.
- Zienkiewicz O.C., Zhu J.Z. A simple error estimator and adaptive procedure for practical engineering analysis // Int. J.
- Accuracy of finite element predictions in sideways load configurations for the proximal human femur / L. Grassi, E. Schileo, F. Taddei, L. Zani, M. Juszczyk, L. Cristofolini // J. Biomech. - 2012. -Vol. 45, no. 2. - P. 394-399. DOI: 10.1016/j.jbiomech.2011.10.019
- Improved USCT of paired bones sing wavelet-based image processing / F. Marwa, E. Y. Wajih, L. Philippe, M. Mohsen // IJIGSP. -2018. - Vol. 10, no. 9. - P. 1-9. DOI: 10.5815/ijigsp.2018.09.01
- Method for quantitative assessment of acetabular bone defects / G. Hettich, R.A. Schierjott, H. Ramm, H. Graichen, V. Jansson, M. Rudert, F. Traina, T.M. Grupp // J. Orthop. Res. -2018. - Vol. 37, no. 1. - P. 181-189. DOI: 10.1002/jor.24165
- Automatically gradient threshold estimation of anisotropic diffusion for Meyer's watershed algorithm based optimal segmentation / K.P.K. Mithun, A. Gauhar, M.R. Mohammad, A.S.M.H. Delowar // IJIGSP. - 2014. - Vol. 6, no. 12. -P. 26-31. DOI: 10.5815/ijigsp.2014.12.04
- Mithun K.P.K., Mohammad M.R. Metal artifact reduction from computed tomography (CT) images using directional restoration filter // IJITCS. - 2014. - Vol. 6, no. 6. -P. 47-54. DOI: 10.5815/ijitcs.2014.06.07
- Automation of bone tissue histology / V.V. Yaikova, O.V. Gerasimov, A.O. Fedyanin, M.A. Zaytsev, M.E. Baltin, T.V. Baltina, O.A. Sachenkov // Front. Phys. - 7(JUN). - 2019. -Vol. 91. DOI: 10.3389/fphy.2019.00091


