Многопорядковые оптические пространственные вихревые фильтры для одновременного выделения контуров различных частей объекта
Автор: Хонина С.Н., Порфирьев А.П., Хорин П.А., Дзюба А.П., Серафимович Д.П., Скиданов Р.В.
Журнал: Компьютерная оптика @computer-optics
Рубрика: Дифракционная оптика, оптические технологии
Статья в выпуске: 4 т.48, 2024 года.
Бесплатный доступ
Проведено исследование преобразования анализируемого объекта набором из нескольких пространственных фильтров, включая полосовые, дифференциальные, радиальные и вихревые различных типов, а также фильтры Гильберта. Численно продемонстрированы преимущества и недостатки различных фильтров по четкости, инвариантности направления выделения краев, а также энергетической эффективности. На основе полученных результатов разработаны многопорядковые оптические пространственные вихревые фильтры для одновременного выделения контуров различных частей объекта. При этом показано, что многопорядковый фильтр позволяет в одной плоскости формировать набор картин с чётко выделенным полным контуром, углами объекта и различными частями контура. Проведена численная и экспериментальная апробация 4- и 5-канальных пространственных вихревых фильтров различных типов на тестовых объектах. Показана возможность использования предложенных фильтров для одновременного выделения краёв всего изображения и различных частей изображения с целью извлечения большего числа особенностей из анализируемого изображения.
Пространственная фильтрация, выделение краёв, многопорядковые оптические фильтры
Короткий адрес: https://sciup.org/140308601
IDR: 140308601 | DOI: 10.18287/2412-6179-co-1497
Текст научной статьи Многопорядковые оптические пространственные вихревые фильтры для одновременного выделения контуров различных частей объекта
Оптическая обработка даёт возможность с большой скоростью проводить параллельную обработку больших массивов информации в режиме реального времени [1, 2], что остаётся привлекательной характеристикой оптических вычислений, несмотря на определённые сложности преобразования оптикоцифровой информации [3–5].
Оптическая реализация таких математических операций, как свёртка и корреляция, легко осуществляется с использованием обычных линз, действие которых описывается преобразованием Фурье [6– 10].
Оптическое преобразование Фурье (ОПФ) – мощный инструмент, широко используемый для преобразования оптических данных при условии когерентности излучения [11 – 13].
Манипулирование пространственными частотами двумерных изображений с помощью ОПФ хорошо изучено для таких приложений, как контрастирование, выделение контуров, распознавание объектов, криптография [14 – 24].
В частности, в работе [19] было продемонстрировано усиление контуров за счёт блокирования областей с низкой пространственной частотой.
Однако простой метод амплитудной пространственной фильтрации имеет некоторые недостатки. При изменении объекта будет меняться и пространственно-частотный спектр в плоскости Фурье.
Таким образом, каждый раз размер пространственного фильтра должен изменяться соответствующим образом и должен быть точно размещён по центру на плоскости Фурье.
Чтобы преодолеть эти трудности, исследователи разработали несколько методов нелинейной фильтрации для самоадаптирующихся вычислений в реальном времени. В качестве нелинейной среды используются органические и биологические молекулы, фо-торефрактивные полимеры и жидкие кристаллы. Като и Гудман продемонстрировали логарифмическую фильтрацию, поместив полутоновый контактный экран (периодический массив виньетированных точек на гибкой опоре) во входной плоскости когерентной оптической системы [25]. Спектр Фурье обрабатывается соответствующим пространственным фильтром и подвергается обратному преобразованию Фурье. Обработанное изображение отображает желаемую нелинейную функцию исходной интенсивности. Бабкина и соавторы использовали акустооптический модулятор в плоскости Фурье для выполнения про- странственно-частотной фильтрации, когда акустическая волна в кристалле парателлурита образует решётку [26]. Когда падающий оптический луч взаимодействует с решёткой, свет дифрагирует в нулевой и первый порядок. При изменении частоты возбуждения модулятора в дифрагированном луче в реальном времени наблюдается эффект усиления краёв.
Гибридные методы обработки также предлагаются и демонстрируются в литературе, когда пространственные фильтры (созданные в цифровом виде на компьютере) отображаются на пространственном модуляторе света (ПМС), помещённом в фокусе плоскости для обработки в реальном времени [20, 21]. Такой гибридный метод обработки был применён для получения фазово-контрастных изображений живых биологических образцов [27].
Удобным подходом, не зависящим от размера пространственного спектра, является применение вихревых фазовых фильтров. Впервые вихревой пространственный фазовый фильтр использован для выполнения радиального преобразования Гильберта [28]. В отличие от обычно используемых в обработке изображений двумерных преобразований Гильберта [29], радиальное преобразование является изотропным [30, 31]. Показано существенное увеличение контраста при использовании вихревого фильтра, в том числе по сравнению с фильтром высоких частот.
В работе [32] предложено заменить центральную часть вихревого пространственного фильтра на постоянное значение. В этом случае формируется псев-дорельефное изображение, облегчающее визуальное наблюдение мелких деталей изображения. Также при небольшой модификации центральной части рассматриваемого фильтра возможно формирование псевдорельефных изображений для визуального выделения протяжённых объектов различных направлений. Аналогичный фильтр использован для построения спираллограмм [33] – интерференционных картин фазовых объектов, учитывающих направление изменения фазовой функции. Это позволяет визуально различать прозрачные объекты, имеющие включения с различной оптической плотностью. В работе [34] были применены вихревые фильтры с различными порядками сингулярности (в том числе дробными) для построения спираллограмм. В общем случае для выпуклых объектов по направлению закрутки можно определить, является ли оптическая плотность объекта больше оптической плотности окружающей среды либо меньше. При этом на численных примерах было показано, что количество витков спиралей на спирал-лограммах пропорционально кратности набега фазы.
Модифицированные вихревые фильтры, например, дробных порядков [35, 36], анизотропные [37, 38] или смещённые [39] могут использоваться для избирательного выделения краёв.
Вихревые фильтры высоких порядков были использованы для расщепления контуров световых кри- вых и внесения фазового градиента вдоль этих кривых [40]. Расщепление светового контура востребовано при оптическом захвате и манипуляциях с микрочастицами различного типа (прозрачными и непрозрачными). Генерация фазового градиента востребована в различных приложениях, так как в этом случае формируется поперечный поток энергии в заданном направлении.
В задачах выделения краёв и визуального контрастирования выбор параметров вихревого фильтра может быть не очевидным и требовать подбора. Подбор параметров можно осуществлять динамически за счёт использования ПМС. Однако это не только требует перестройки распределения, но и определения характера перестройки. В данной работе предлагается осуществить одновременное преобразование анализируемого объекта набором из нескольких пространственных фильтров, которые могут различаться как по типам, так и параметрически.
Такое преобразование возможно осуществить при использовании многопорядковых дифракционных оптических элементов [41], согласованных с заданным набором фильтров.
Удобство такого подхода состоит в том, что одновременно визуализируется преобразование различных типов или с различными параметрами. В этом случае возможна сравнительная оценка преобразованных распределений по некоторым критериям, в том числе по визуальному восприятию.
1. Теоретические основы
Основная концепция оптической обработки на основе пространственного фильтра с использованием ОПФ показана на рис. 1.
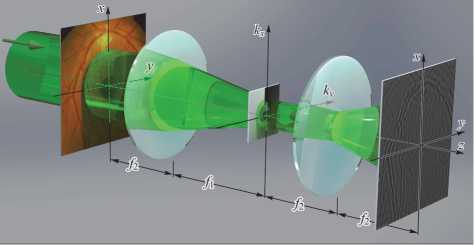
Рис. 1. Схема для реализации оптической пространственной фильтрации на основе двухлинзовой системы
Коллимированный лазерный пучок падает на обрабатываемый объект. Затем излучение, несущее информацию об объекте, преобразуется через ОПФ с помощью собирающей линзы, отображая разные пространственные частоты в разные области в фокальной плоскости.
В спектре Фурье низкие пространственные частоты находятся в центральной части с высокой интенсивностью, а высокие пространственные частоты – на периферии с низкой интенсивностью. Различные ме- тоды обработки изображения, такие как низкочастотный, полосовой, высокочастотный, а также фазовые фильтры, используются в плоскости Фурье для обработки различных пространственных частот для различных приложений. Обратное преобразование Фурье с другой линзой используется для отображения преобразованного изображения на ПЗС-камеру.
Математически действие оптической пространственной фильтрации на основе двухлинзовой системы описывается следующим образом. Действие первой линзы соответствует преобразованию Фурье от объекта:
G ( и , v ) = 3 { g ( x, y ) } =
Ю
= k If g ( x , У )exp - k ( xu + yv ) d x d У , f i -L L f i _
- L
где k = 2п / X - волновое число лазерного излучения с длиной волны X , f , - фокусное расстояние первой линзы.
Далее выполняется действие фильтрации при прохождении пространственного спектра (1) через фильтр H ( u , v ), расположенный в фокальной плоскости:
G H ( и , v ) = G ( и , v ) H ( и , v ). (2)
Затем действие второй линзы с фокусным расстоянием f 2 позволяет сформировать преобразованное изображение:
g H ( x ', y ') = 3 { G ( и , v) } =
L
= — IJ g ( и , v )exP - ~( x ' и + У ' v ) d x d У • f 2-V L f 2' J
-L
Хорошо известны амплитудные фильтры следу- ющего вида:
0, 0 < 4и 2 + v 2 < r s ,
H ( и , v ) =
1, rs < 4 и 2 + v 2 < r ,
0, re< V и 2 + v 2.
Выделение краёв при использовании полосового фильтра (4) достигается за счёт подавления низких пространственных частот и выделения высокочастотных компонент, которые как раз соответствуют резким перепадам яркости объекта.
Отметим, что при использовании полосовых фильтров (4) требуется подобрать оптимальные параметры (радиусы полос) для выполнения определённой операции.
Также для выделения краев применяют пространственные фильтры, осуществляющие оптическое дифференцирование вдоль направления под углом ф о :
H ( и , v ) = и cos ф 0 + v sin ф 0. (5)
Принцип действия основан на резком увеличении значения производной на краях, где происходит значи- тельное изменение яркости объекта. Из выражения (5) также видно, что такой фильтр усиливает вклад высокочастотных составляющих. В связи с этим выделять края независимо от направления можно с использованием радиальных фильтров следующего вида:
H ( и , v ) = ( V и 2 + v 2 ) . (6)
Преимущество фильтров (5) и (6) состоит в отсутствии зависимости от размера пространственного спектра. Однако фильтры (4)–(6) являются амплитудными, что приводит к потере энергии, так как часть пространственного спектра блокируется.
Энергетически эффективным является фазовый вариант дифференциального фильтра:
H ( и , v ) = exp [ i arg ( и cos ф 0 + v sin ф 0 ) _ , (7)
где arg() – операция вычисления фазы.
Аналогом фильтра (6) можно считать фильтр Гильберта [29]:
H ( и , v ) = i sgn ( и cos ф 0 + v sin ф 0 ) , (8)
где sgn() – функция взятия знака.
Удобным подходом, сохраняющим энергию, не зависящим от размера пространственного спектра, а также направления выделения краев, является применение вихревых фазовых фильтров следующего вида [28, 30, 31]:
H ( ф ) = exp ( im ф ).. (9)
Универсальность вихревого фильтра (9) состоит в том, что вариации одного параметра m , который может принимать дробные значения, позволяет выделять не только контур целиком, но и отдельные части [35, 36]. Кроме того, смещение вихревого фильтра также позволяет выполнять направленное выделение контуров [39].
2. Численное моделирование 2.1. Пространственная фильтрация на основе двухлинзовой системы
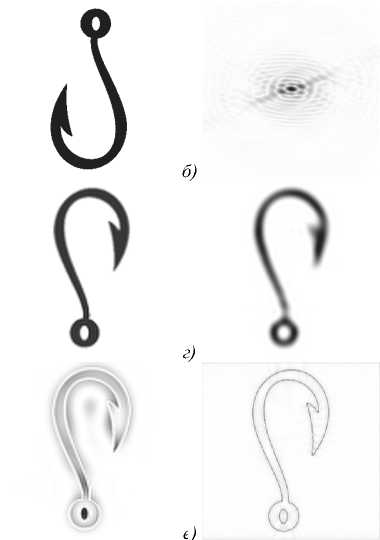
На рис. 2 показаны результаты применения фильтра (4) для подавления высоких (рис. 2 в , г ) и низких частот (рис. 2 д , е ) к пространственному спектру изображения «крюк» (рис. 2 а , б ). В качестве нормированного радиуса r 0 выбран размер центрального светового пятна пространственного спектра (рис. 2 б ).
Видно, что при подавлении низких частот в области радиусом около 5 r 0 можно выделить контур изображения.
-
2.2. Применение вихревого фильтра
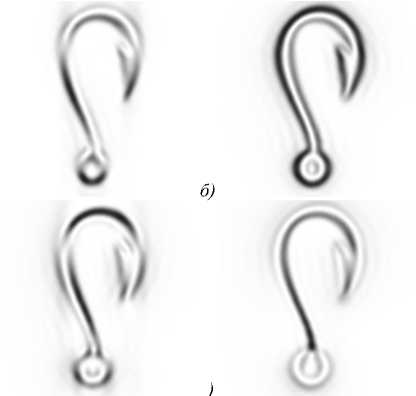
Хотя наиболее известным является вихревой фазовый фильтр первого порядка m = 1, возможно использование фильтров с более высокими целыми, а также дробными порядками.
На рис. 3 показаны результаты моделирования применения различных фильтров вида (5). Видно, что при m = 1 происходит практически равномерное выделение контура независимо от направления. При использовании других значений m чётче выделяются разные части контура, что может быть полезно для всестороннего анализа объекта.
рис. 4 а , чтобы можно было сравнить с результатами предыдущего параграфа.
д)
a)
в)
е)
Рис. 2. Демонстрация действия амплитудных фильтров (4) для объекта «крюк» (a) в плоскости его пространственного спектра (б):
r s = 0, r e = 15r 0 (в), r s = 0, r e = 7r 0 (г), r s = 2r 0 , r e = 15r 0 (д), r s = 5r 0 , r e = 30r 0 (е) (рисунки инвертированы)
2.3. Применение дифференцирующего и радиального фильтров
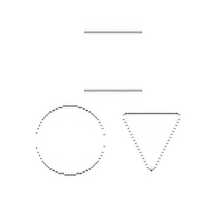
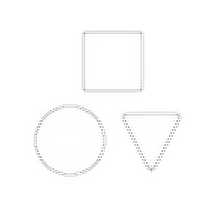
Чтобы продемонстрировать действие дифференцирующего фильтра (5) вдоль некоторого направления, а также радиального фильтра (6), мы рассмотрели объект, состоящий из нескольких фигур, имеющих различную геометрию контуров (рис. 4 а ).
Как видно из рис. 4, дифференцирующие фильтры очень четко выделяют соответствующие направления. Радиальные фильтры выделение краев осуществляют менее четко, однако оно происходит равномерно по всем направлениям.
2.4. Сравнение действия классического и радиального фильтра Гильберта
В данном параграфе мы демонстрируем (рис. 5) особенности классического фильтра Гильберта [29] и радиального фильтра Гильберта [30, 31].
Отметим, что радиальный фильтр Гильберта (рис. 5 и, к ) фактически соответствует вихревому фазовому фильтру [28] первого порядка m = 1 (9). Эти фильтры мы применили к объекту, представленному
в)
a)
a)
г)
Рис. 3. Демонстрация действия вихревых фильтров (5): m = 0,5 (a), m = 1 (б), m = 1,5 (в), m = 2 (г) (рисунки инвертированы)
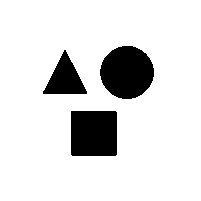
в)
д) Рис.
б)

г)


е)
4. Демонстрация действия дифференцирующего (5)
и радиального (6) фильтров для трёх объектов разной геометрии (a) в плоскости его пространственного спектра (б): горизонтальный (5) (в), вертикальный (5) (г), радиальный (6) при q = 1 (д), при q = 2 (е) (рисунки инвертированы)
Как видно из приведенных на рис. 5 результатов моделирования, одномерный фильтр Гильберта (7) или (8) (рис. 5 а - е ) позволяет выделять направления контуров, соответствующие линии фазового скачка фильтра. Хотя края получаются смазанными по сравнению с дифференциальным фильтром (5), что связа-
но с отсутствием амплитудной составляющей, которая усиливает вклад высоких частот.
и)
a)
в)
д)
ж)

к)
Рис. 5. Демонстрация действия фильтра Гильберта: горизонтальный (а, б), вертикальный (в, г), диагональный (д, е), двумерный (ж, з) и радиальный (9) (и, к) (рисунки инвертированы)
Таким образом, вращая одномерный фильтр Гильберта, можно выделять разные направления контура. Интересно, что диагональный вариант (рис. 5 д , е ) позволил для рассматриваемых объектов осуществить практически равномерное выделение контуров. В то время как двумерный вариант фильтра Гильберта (рис. 5 ж , з ) резко подчеркнул углы квадрата и горизонтальные углы треугольника. Таким образом, имеет место существенная корреляция геометрии фильтра и анализируемых фигур. Если нужно избежать такой ситуации и обеспечить равномерное
(анизотропное) выделение контура, то используют так называемое радиальное преобразование Гильберта [30, 31], которое фактически соответствует использованию вихревого фильтра (9) первого порядка [28].
Таким образом, на основе проведенных исследований, компромиссным и в то же время универсальным вариантом можно считать вихревой фазовый фильтр.
-
2.5. Многопорядковые ДОЭ, согласованные с вихревыми фильтрами
Как было продемонстрировано выше, вариации параметров рассматриваемых пространственных фильтров приводят к существенному изменению картины преобразованного объекта: возможно как равномерное выделение контура, так и подчёркивание отдельных частей и направлений.
Чтобы обеспечить одновременное преобразование анализируемого объекта набором из нескольких пространственных фильтров, которые могут различаться как по типам, так и параметрически, предлагается использовать многопорядковые дифракционные оптические элементы, согласованные с заданным набором фильтров. Принцип расчёта многопорядковых ДОЭ основан на кодировании набора заданных фильтров разными пространственными несущими частотами [41]:
P
Т ( X , У ) = ^ H p ( X , y )exp [ i ( a p X + в р У ) ] , (10) p = 1
где H p ( x, y ) – вихревой фильтр вида (4) с различными значениями вихревого порядка, ( a р , в p ) - пространственные частоты.
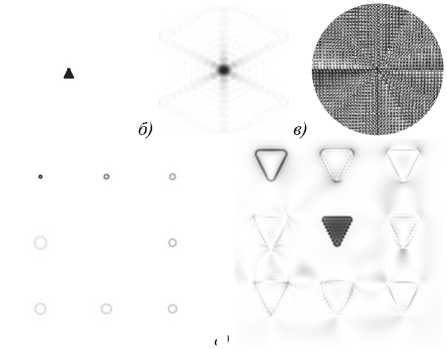
На рис. 6 показаны результаты применения 8-порядкового ДОЭ (10), согласованного с вихревыми фильтрами (9) при m = 1, ..., 8, к пространственному спектру изображения «треугольник» (рис. 6 а , б ). Видно, что наиболее чётко контур выделяется при m = 1. При m =2,3 более ярко выделяются углы объекта. При более высоких порядках m подчёркиваются различные части контура.
В работах [35–39] были рассмотрены различные модификации вихревых фильтров (9) с целью избирательного выделения краёв. Некоторые из них были использованы при численном и экспериментальном исследовании, описанном в следующем параграфе.
3. Экспериментальные результаты
Для экспериментального исследования предложенных многоканальных фильтров была использована оптическая установка на основе отражательного пространственного модулятора света (ПМС) HOLOEYE PLUTO VIS (1920×1080 пикселей, размер пикселя – 8 мкм) для реализации рассчитанных фазовых масок фильтров (см. рис. 7).
Линейно поляризованный Гауссов пучок твердотельного лазера (длина волны λ = 532 нм) расширялся и коллимировался с помощью комбинации двух линз L1 и L2 с фокусными расстояниями 25 и
150 мм. Зеркала М1 и М2 направляли сформированный пучок на дисплей модулятора света. Для реализации тестовых амплитудных изображений различных двумерных объектов использовались амплитудные маски пользованной при вставке к рис. 7).
АМ (пример такой маски, ис-моделировании, показан на
а)
г)
д)
Рис. 6. Моделирование применения к объекту «треугольник» (а) в плоскости пространственного спектра (б) 8-порядкового ДОЭ, согласованного с вихревыми фильтрами (9) при m = 1,…,8: фаза ДОЭ (в), вид ФРТ (г), действие для изображения «треугольник» (д) (рисунки инвертированы)
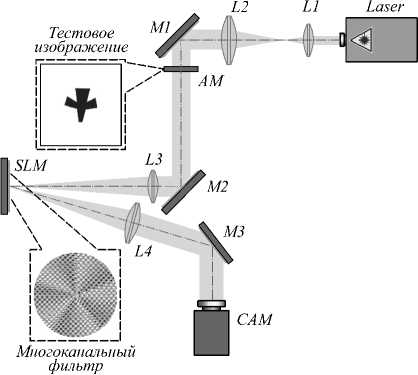
Рис. 7. Оптическая схема для экспериментального исследования рассчитанных многоканальных фильтров: Laser – твердотельный лазер (λ = 532 нм); L1, L2, L3, L4, L5 и L6 – линзы (фокусные расстояния f 1 = 25 мм, f 2 = 150 мм, f 3 = 500 мм и f 4 = 500 мм); M1, M2 и M3 – зеркала; АМ – амплитудная маска; SLM – отражающий ПМС; CAM – видеокамера. На вставках показан пример амплитудного объекта и пример фазовой маски рассчитанного многоканального фильтра
Затем для реализации 4-f оптической системы пространственной фильтрации использовалась комбинация линз L3 и L4 с фокусным расстоянием 500 мм, а также модулятор света. Пример фазовой маски, использованной в эксперименте и реализованной с помощью модулятора света, показан на вставке к рис. 7.
Для регистрации распределений интенсивности, сформированных в выходной плоскости используемой 4-f оптической системы, была использована видеокамера САМ (TOUPCAM UHCCD00800KPA, 1600×1200 пикселей, с размером пикселя 3,34 мкм). Зеркало M3 при этом направляло пучок, отражённый от модулятора, на дисплей видеокамеры.
В экспериментах мы исследовали три различных типа многоканальных фильтров:
I тип: 5-канальный фильтр, согласованный с оптическим вихревым (ОВ) пучком первого порядка, распространяющимся на оси оптической системы, и набором из четырёх несимметричных ОВ-пучков первого порядка с различными поперечными смещениями их центров относительно осей их распространения;
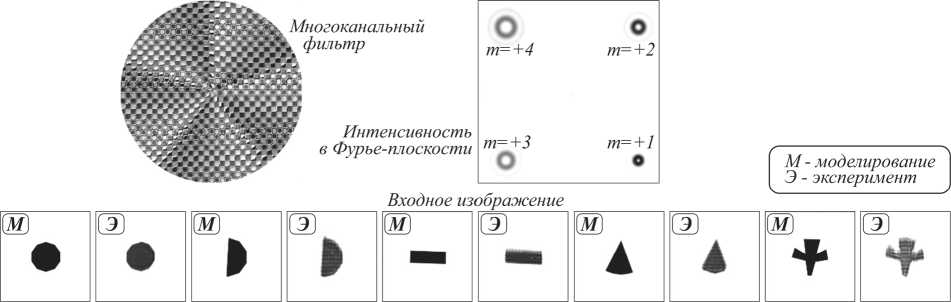
II тип: 4-канальный фильтр, согласованный с набором ОВ-пучков с топологическими зарядами +1, +2, +3 и +4;
III тип: 5-канальный фильтр, согласованный с ОВ-пучком первого порядка, распространяющимся на оси оптической системы, и набором суперпозиций двух ОВ-пучков с топологическими зарядами ±1 и различными фазовыми сдвигами Δφ между пучками в суперпозиции.
В случае первого из исследованных фильтров (5-ти канальный фильтр I типа) смещения центров пучков определялись параметром Δ. Для ОВ-пучков, сформированных в различных порядках дифракции, центр пучка смещался на ∆ по одной из прямоугольных координат в положительном или отрицательном направлении (рис. 8). Как известно, такое смещение центра пучка приводит к формированию асимметричного распределения интенсивности со спиральным волновым фронтом [42, 43]. Положение максимума интенсивности генерируемого асимметричного распределения интенсивности зависит от направления смещения центра пучка. Таким образом, такие многоканальные фильтры можно использовать для одновременного выделения контуров всего изображения в целом и его различных частей (например, верхней/нижней, правой/левой). Результаты, полученные в этом случае численно и экспериментально для нескольких различных изображений, показаны на рис. 8.
В случае 4-канального фильтра II типа при его освещении плоской волной разные ОВ-пучки формируются в разных порядках дифракции (рис. 9). Результаты, полученные численно и экспериментально для нескольких различных изображений с помощью этого фильтра, показаны на рис. 9.
Последний из исследованных – 5-канальный фильтр III типа – был предназначен для формирования как осевого ОВ-пучка первого порядка, так и набора суперпозиций ОВ-пучков ±1 порядка с различными фазовыми сдвигами Δφ между пучками в суперпозиции. Как известно, суперпозиция двух ОВ-пучков с противополож- ными топологическими зарядами m позволяет сформировать распределения интенсивности в виде расположенных по окружности 2m световых пятен (рис. 10)
-
[44]. Дополнительный фазовый сдвиг между этими ОВ-пучками позволяет контролировать ориентацию формируемых световых пятен.
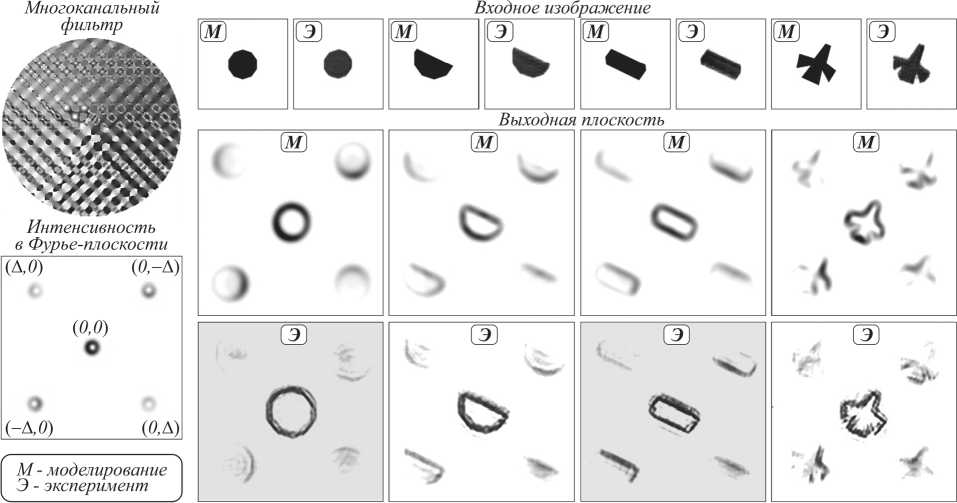
Рис. 8. 5-канальный фильтр I типа. В верхнем ряду показаны фазовая маска рассчитанного многоканального фильтра и сформированное им распределение интенсивности в Фурье-плоскости. Смоделированные и экспериментально полученные распределения интенсивности показаны для четырёх различных амплитудных масок. Рисунки инвертированы
Выходная плоскость

Рис. 9. 4-канальный фильтр II типа (остальное описание, как на рис. 7). Рисунки инвертированы
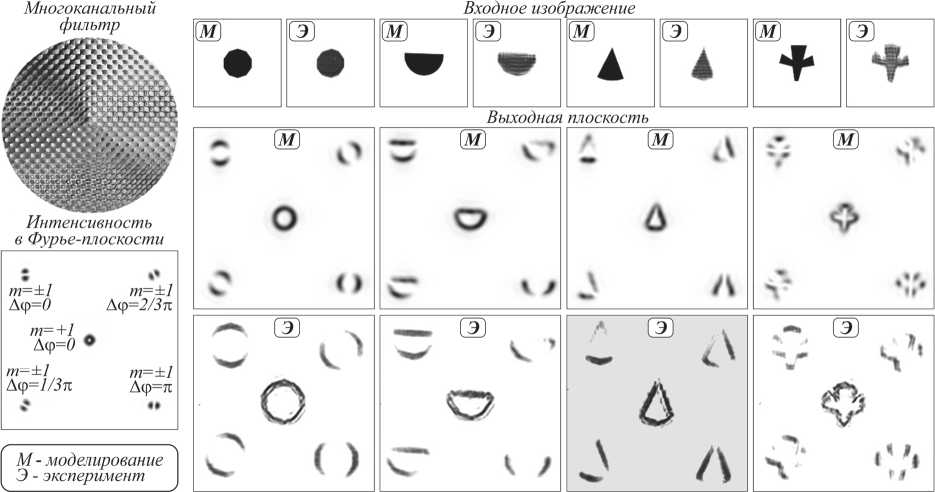
Рис. 10. 5-канальный фильтр III типа (остальное описание, как на рис. 7). Рисунки инвертированы
Как видно из рис. 10, многоканальный фильтр III типа формирует разнесённую по окружности серию двойных световых пятен, повёрнутых относительно друг друга на различные углы. Этот фильтр можно использовать для одновременного выделения контуров всего изображения и различных противолежащих частей изображения, вдоль определённых направлений. Дальнейшие модификации такого фильтра могут быть использованы для формирования ещё большего числа суперпозиций, повёрнутых друг к другу на меньшие углы, и таким образом позволят проанализировать ещё больше локальных участков контуров анализируемого изображения.
Заключение
Пространственная фильтрация оптическими методами является мощным инструментом для обработки оптических сигналов и улучшения качества изображений, в том числе выделения краёв объектов. Существуют различные оптические фильтры, которые могут быть использованы для выделения краёв объектов на изображении. Выбор конкретного метода зависит от характеристик изображения, требований к точности и эффективности, а также от конкретной задачи обработки, которую необходимо решить.
В данной работе мы численно продемонстрировали действие различных пространственных фильтров, включая полосовые, дифференциальные, радиальные и вихревые различных типов, а также фильтры Гильберта. На фигурах, имеющих различную геометрию контуров, показаны преимущества и недостатки различных фильтров по четкости и инвариантности направления выделения краев.
Для обеспечения возможности сравнительной оценки преобразования объекта, в том числе по визу- альному восприятию, нами предложено совмещение сразу нескольких фильтров в одном многопорядковом оптическом элементе.
Одной из особенностей многопорядковых оптических фильтров является параллельная обработка, что позволяет быстро анализировать объекты, преобразованные различными способами.
Показана эффективность многопорядковых фильтров, согласованных с вихревой фазой различных порядков, в том числе, со смещением, для одновременного выделения контуров объекта, его углов и различных частей контура. Проведена численная и экспериментальная апробация 4- и 5-канальных пространственных вихревых фильтров различных типов на тестовых объектах. Показана возможность использования предложенных фильтров для одновременного выделения краёв всего изображения и различных частей изображения, в том числе вдоль определённых направлений, с целью извлечения большего числа особенностей из анализируемого изображения.
Исследование выполнено в рамках научной программы Национального центра физики и математики, направление № 1 «Национальный центр исследования архитектур суперкомпьютеров. Этап 2023-2025» в части численного моделирования, а также в рамках Государственного задания НИЦ «Курчатовский институт» в теоретической части.
Список литературы Многопорядковые оптические пространственные вихревые фильтры для одновременного выделения контуров различных частей объекта
- Gillespie WA. Optical information processing. Phys Educ 1999; 127(3): 29. DOI: 10.1088/0031-9120/29/3/003.
- Goswami D. Optical computing. Resonance 2003; 8(6): 56-71. DOI: 10.1007/BF02837869.
- Ambs P. Optical computing: A 60-year adventure. Adv Opt Technol 2010; 2010: 372652. DOI: 10.1155/2010/372652.
- Minzioni P, Lacava C, Tanabe T, et al. Roadmap on all-optical processing. J Opt 2019; 21(6): 063001. DOI: 10.1088/2040-8986/ab0e66.
- Kazanskiy NL, Butt MA, Khonina SN. Optical computing: Status and perspectives. Nanomaterials 2022; 12(13): 2171. DOI: 10.3390/nano12132171.
- Weaver CS, Goodman JW. A technique for optically convolving two functions. Appl Opt 1966; 5(7): 1248-1249. DOI: 10.1364/AO.5.001248.
- Kasana RS, Bhatnagar GP, Dubey MC. A review of mathematical operations by optical data processing. IEE-IERE Proc India 1975; 13(4): 134-144. DOI: 10.1049/iipi.1975.0034.
- Javidi B, Hornder JL. Real-time optical information processing. Boston: Academic Press; 1994.
- Goodman JW. 4 decades of optical information processing. Opt Photonics News 1991; 2(2): 11-15. DOI: 10.1364/OPN.2.2.000011.
- Heanue JF, Bashaw MC, Hesselink L. Volume holographic storage and retrieval of digital data. Science 1994; 265(5173): 749-752. DOI: 10.1126/science.265.5173.74.
- Goodman J. Introduction to Fourier optics. San Francisco, CA: McGraw Hill; 1968.
- Bracewell RB. The Fourier transform and its application. McGraw Hill; 1965.
- Stark H. Applications of optical Fourier transforms. Elsevier; 2012.
- Zernike F. How I discovered phase contrast. Science 1955; 121(3141): 345-349. DOI: 10.1126/science.121.3141.345.
- Casasent D, Furman A. Determining optimal matched filter parameters. Appl Opt 1976; 15(7): 1690-1691. DOI: 10.1364/AO.15.001690.
- Yu FTS, Gregory DA. Optical pattern recognition: architectures and techniques. Proc IEEE 1996; 84(5): 733-752. DOI: 10.1109/5.488743.
- Glückstad J, Mogensen PC, Eriksen RL. The generalized phase contrast method and its applications. DOPSNYT 2001; 1: 49-54.
- Juday R. Correlation filter basics. In Book: Vijaya Kumar BVK, Mahalanobis A, Juday RD. Correlation pattern recognition. Ch 5. Cambridge University Press; 2005: 130-195. DOI: 10.1017/cbo9780511541087.006.
- Yelleswarapu CS, Kothapalli S-R, Rao DVGLN. Optical Fourier techniques for medical image processing and phase contrast imaging. Opt Commun 2008; 281(7): 1876-1888. DOI: 10.1016/j.optcom.2007.05.072.
- Schausberger SE, Heise B, Maurer C, Bernet S, Ritsch-Marte M, Stifter D. Flexible contrast for low-coherence interference microscopy by Fourier-plane filtering with a SLM. Opt Lett 2010; 35(24): 4154-4156. DOI: 10.1364/OL.35.004154.
- Maurer C, Jesacher A, Bernet S, Ritsch-Marte M. What spatial light modulator can do for optical microscopy. Laser Photon Rev 2011; 5(1): 81-101. DOI: 10.1002/lpor.200900047.
- Zheng GA, Horstmeyer R, Yang CH. Wide-field, high-resolution Fourier ptychographic microscopy. Nat Photon 2013; 7(9): 739-745. DOI: 10.1038/nphoton.2013.187.
- Xiong Y, He A, Quan C. Cryptanalysis of an optical cryptosystem based on phase-truncated Fourier transform and nonlinear operations. Opt Commun 2018; 428: 120-130. DOI: 10.1016/j.optcom.2018.07.058.
- Zhu X, Yao H, Yu J, Gbur G, Wang F, Chen Y, Cai Y-j. Inverse design of a spatial filter in edge enhanced imaging. Opt Lett 2020; 45(9): 2542-2545. DOI: 10.1364/OL.391429.
- Kato H, Goodman JW. Nonlinear filtering in coherent optical systems through halftone screen processes. Appl Opt 1975; 14(8): 1813-1824. DOI: 10.1364/AO.14.001813.
- Babkina TM, Voloshinov VB. A new method of acousto-optic image processing and edge enhancement. J Opt A: Pure Appl Opt 2001; 3(4): S54. DOI: 10.1088/1464-4258/3/4/359.
- Popescu G, Deflores LP, Vaughan JC, Badizadegan K, Iwai H, Dasari RR, Feld MS. Fourier phase microscopy for investigation of biological structures and dynamics. Opt Lett 2004; 29(11): 2503-2505. DOI: 10.1364/OL.29.002503.
- Khonina SN, Kotlyar VV, Shinkaryev MV, Soifer VA, Uspleniev GV. The phase rotor filter. J Mod Opt 1992; 39(5): 1147-1154. DOI: 10.1080/09500349214551151.
- Hahn SL. Hilbert transforms in signal processing. Boston, London: Artech House; 1996.
- Davis JA, McNamara DE, Cottrell DM, Campos J. Image processing with the radial Hilbert transform: Theory and experiments. Opt Lett 2000; 25(2): 99-101. DOI: 10.1364/OL.25.000099.
- Guo C-S, Han Y-J, Xu J-B, Ding J. Radial Hilbert transform with Laguerre-Gaussian spatial filters. Opt Lett 2006; 31(10): 1394-1396. DOI: 10.1364/OL.31.001394.
- Jesacher A, Furhapter S, Bernet S, Ritsch-Marte M. Shadow effects in spiral phase contrast microscopy. Phys Rev Lett 2005; 94(23): 233902. DOI: 10.1103/PhysRevLett.94.233902.
- Maurer C, Bernetand S, Ritsch-Marte M. Refining common path interferometry with a spiral phase Fourier filter. J Opt A: Pure Appl Opt 2009; 11(9): 094023. DOI: 10.1088/1464-4258/11/9/094023.
- Ananin MA, Khonina SN. Modelling of optical processing of images with use of the vortical spatial filter. Computer Optics 2009; 33(4): 466-472.
- Davis JA, Smith DA, McNamara DE, Cottrell DM, Campos J. Fractional derivatives – analysis and experimental implementation. Appl Opt 2001; 40(32): 5943-5948. DOI: 10.1364/AO.40.005943.
- Wang J, Zhang W, Qi Q, Zheng S, Chen L. Gradual edge enhancement in spiral phase contrast imaging with fractional vortex filters. Sci Rep 2015; 5: 15826. DOI: 10.1038/srep15826.
- Situ G, Pedrini G, Osten W. Spiral phase filtering and orientation-selective edge detection/enhancement. J Opt Soc Am A 2009; 26(8): 1788-1797. DOI: 10.1364/JOSAA.26.001788.
- Sharma MK, Joseph J, Senthilkumaran P. Selective edge enhancement using anisotropic vortex filter. Appl Opt 2011; 50(27): 5279-5286. DOI: 10.1364/AO.50.005279.
- Sharma MK, Joseph J, Senthilkumaran P. Selective edge enhancement using shifted anisotropic vortex filter. J Opt 2013; 42: 1-7. DOI: 10.1007/s12596-012-0089-6.
- Khonina SN, Porfirev AP. Generation of multi-contour plane curves using vortex beams. Optik 2021; 229: 166299. DOI: 10.1016/j.ijleo.2021.166299.
- Kazanskiy NL, Khonina SN, Karpeev SV, Porfirev AP. Diffractive optical elements for multiplexing structured laser beams. Quantum Electron 2020; 50(7): 629-635. DOI: 10.1070/QEL17276.
- Kotlyar VV, Kovalev AA, Porfirev AP. Asymmetric Gaussian optical vortex. Opt Lett 2017; 42(1): 139-142. DOI: 10.1364/OL.42.000139.
- Khonina SN, Ustinov AV. Focusing of shifted vortex beams of arbitrary order with different polarization. Opt Commun 2018; 426: 359-365. DOI: 10.1016/j.optcom.2018.05.070.
- Vickers J, Burch M, Vyas R, Singh S. Phase and interference properties of optical vortex beams. J Opt Soc Am A 2008; 25(3): 823-827. DOI: 10.1364/JOSAA.25.000823.


