Compressibility of the composite material with fiber filler and nanodimensional polyurethane matrix for road and hydro engineering construction

Original article

H ighly porous non-woven needle-punched fabrics made of synthetic fibers with a porosity coefficient of more than 0.8 [1–5] are used in road [6–9] and hydraulic engineering [10–13] construction and water drainage systems [14–17]. The general requirement for fabrics is high water permeability, which depends on the change in the porous structure when compressed by a layer of soil or road surface. The consequence of compression is a decrease in porosity and the permeability of the cloths [3–5].

When using composite materials obtained by impregnating fabrics with polymer dispersions [18], the compressibility decreases. The mechanical properties and permeability of composite materials depend on the degree of impregnation and on the distribution of polymer particles

in the cloth. The formation of a nanoscale thickness coating of polymer particles on the fibers surface increases the fibers rigidity, the connection of the fibers by “bridges” of polymer particles limits the movement of the fibers in the direction of the exposure direction [18, 19]. Moreover, with the predominant formation of the nanoscale coating on the fibers surface and connecting bridges, the macroscopic degree of fabric porosity does not change and the relatively high water permeability remains.

The condition for the coating formation on the fibers is their wetting with water and a high affinity between the dispersion polymer and the fiber-forming polymer, which is not achieved when synthetic rubbers are used to impregnate latex fabrics [19]. Water dispersions of polyurethanes have been proposed [20–22], which have a high affinity for polyethylene terephthalate [23–26]. However, the technological problems of obtaining composite mate-

TECHNOLOGY OF PRODUCTION OF BUILDING MATERIALS AND PRODUCTS rials with a given compressibility remain unsolved. Therefore, the aim of the work is to study the dependence of the composite material compressibility on the impregnation degree of fabrics made of polyethylene terephthalate fibers with a polyurethane dispersion.

METHODS AND MATERIALS

The fabrics compressibility degree was determined as the result of five measurements averaging. Under the compression strength of 20 kPa the maximum relative error was ±7%. And the relative measurement error decreased with the compression strength growing.

RESULTS AND DISCUSSION

The objects of the study are the non-woven needle-punched fabrics made of polyethylene terephthalate fibers (TU 6-13-0204077-95-91) with a linear density of 0.33 tex and 20–25 µm diameter. The fibrous cloths were obtained with the mechanical formation technique [12] and were strengthened with needle-punching density of 180 cm–2 [27]. The thickness, the surface and the bulk density of the fabric were 2.5±0.1 mm, 0.25±0.02 kg/m2 and 95±5 kg/m3 respectively. The experimental samples’ mass was determined using electronic scales with a weighing accuracy of ±0.002 g. The samples’ thickness was determined according to GOST 12023-93 using a thickness gauge with a division value of 0.01 mm according to GOST 11358-70.

We used for impregnation a water dispersion of an anionic stabilized aliphatic polyetherurethane (IMPRA-NIL DL 1380 (China)) with a dry residue of 40%. The samples were immersed in a dispersion filled container and rolled for 3–5 min at room temperature. Then the samples were removed from the container, kept on a metal mesh until the dispersion completely drained and rolled between the rollers with a half of the cloth thickness gap. The impregnation degree was varied by diluting dispersion with different dry residue using the distilled water. The heat treatment of the impregnated cloths was carried out at a temperature of 90оC. The composite materials’ impregnation degree (CM, rel. units) was calculated from the expression:

The effect of pressure on the compressibility of composite materials of various degrees of impregnation.

Dependences of ε on P are shown in Fig. 1.

The obtained dependences of ε on P (Fig. 1) are described by equation:

ε = a–bР ² +cР ³.                               (3)

A significant number of variables (a, b, kPa–2 and c, kPa–3) reduces the criterion value of Equation 3. We have found that in the coordinate system ε-Р0.5, the dependences of ε on Р are transformed into linear ones (Fig. 2) and are described as:

ε = k_P × Р ^0.5   ε < 1,

where k_P is the dimension coefficient, kPa^–1, which corresponds to the compressibility degree of the composite material at 1 kPa pressure. The smaller the kP coefficient, the higher the compressive resistance of the composite material.

The dependence of k_P on C_M was obtained using two assumptions. Firstly, the greater the impregnation degree, the lower the compressibility degree of the composite

с

M

m ₁

- m

m

where m1 and m (kg) are the samples’ mass after and before the impregnation.

The compressibility of the fabrics was determined using a dial indicator according to GOST 577-68 with a thickness measurement accuracy of ±0.001 mm. The composite material compressibility degree (ε) was calculated as:

d - d_P

Г =----— d,

where d (m) is the thickness of the composite material; dP (m) is the thickness of the composite material under pressure P (kPa).

It follows from (2) that at the ultimate strain of the cloth and composite material (or when the condition dР→0), ε→1.

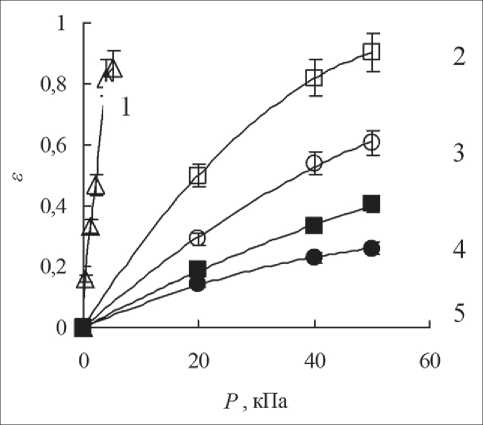
Fig. 1. Dependences of the compressibility degree on pressure for the fabric (1) and the composite material with the degree of impregnation 0,18 (2), 0,27 (3), 0,87 (4) and 1.16 (5)

TECHNOLOGY OF PRODUCTION OF BUILDING MATERIALS AND PRODUCTS

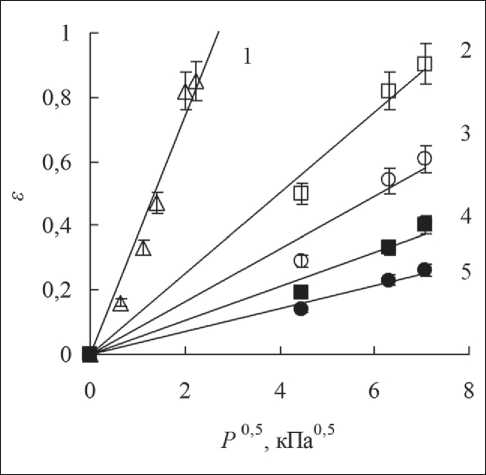
Fig. 2. Dependences of the compressibility degree on the nominal pressure for the fiber (1) and the composite material with the degree of impregnation 0,18 (2), 0,27 (3), 0,87 (4) and 1.16 (5)

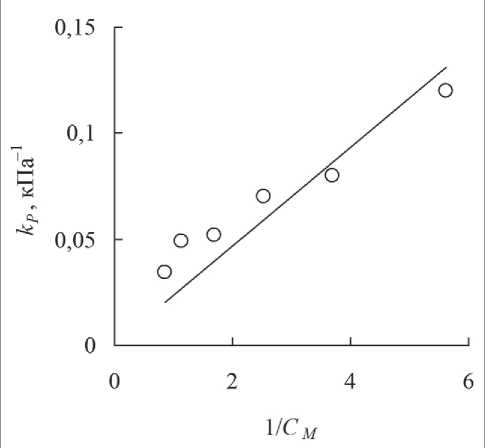
Fig. 3. Dependence of the k _P coefficient on the inverse value of the impregnation degree

material, which leads to an i inverse proportionality between k_P and C_M . Secondly, with the impregnation degree tending to the limit value when all the fiber pores are filled with polymer, the obtained material’s, compressibility degree tends to zero.

The influence of the degree of impregnation on the compressibility of composite materials.

The dependence of kP on 1/ C_M is shown in Fig. 3.

The dependence k_P on 1/ С_М has a linear form (Fig. 3) and with a correlation coefficient of 0.9 is described by the equation:

k_P = 0.023(1/ С_М ).                              (5)

Replacing the coefficient k_P in equation 4 with equation 5, we obtained an equation for predicting the compressibility degree of a composite material with a given impregnation degree and known pressure:

ε = (0.023/ С_М ) P ^0.5.                               (6)

The influence of the degree of impregnation and pressure on the compressibility of composite materials.

The graphical solution of Equation 5 for the given С_М from 0.01 to 1 and P from 1 to 100 kPa is shown in Fig. 4.

A significant decrease in the compressibility degree of the composite material is observed with an increase in the impregnation degree from 0 to 0.5. With the impregnation degree from 0.5 to 1.0, the compressibility degree practically does not depend on the polyurethane content in the composite material. So, for a composite material with the impregnation degree of 0.01, in comparison with a material with the impregnation degree of 0.5 with a pressure of 1 kPa, the compressibility degree decreases from 0.9 to 0.05, and with a pressure of 100 kPa – from 1 to 0.55, respectively (Fig. 4).

Porous structure of composite materials.

Micrographs of a composite material with an impregnation degree of less than 0,5 are shown in Fig. 5.

Visual analysis shows that with the impregnation degree less than 0.5, bridges between the fibers don’t not form. The polyurethane particles form coating on the fibers surface, the thickness and defectiveness of which depend on the impregnation degree. At the impregnation degree of 0.18 the coating has a thickness of approximately 200–500 nm with a high defectiveness (Fig. 5a). With an increase in the coating thickness up to 1000 nm for materials with an impregnation degree of 0.27 the coating defectiveness decreases (Fig. 5b), and for a material with an impregnation degree of 0.48, a monolithic coating is obtained (Fig. 5c). However, compared to the fabric, the composite materials compressibility with an impregnation degree of less than 0.5 decreases slightly.

The decrease in the composite materials compressibility with an impregnation degree of more than 0.5 is a consequence of the formation of bridges between the fibers (Fig. 6, a and b).

TECHNOLOGY OF PRODUCTION OF BUILDING MATERIALS AND PRODUCTS

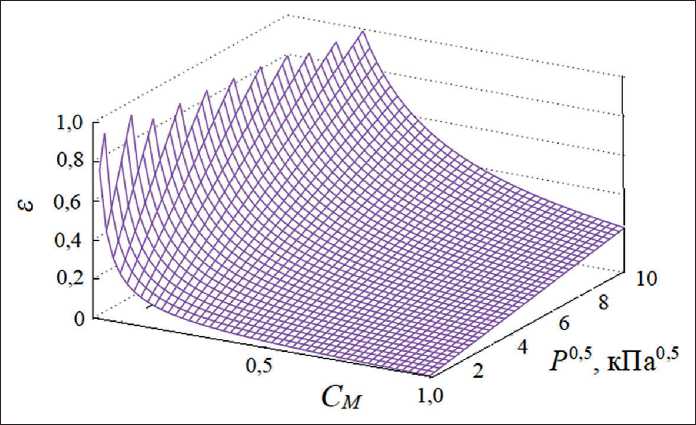
Fig. 4. Dependences of the compressibility degree of composite materials on the impregnation degree and pressure

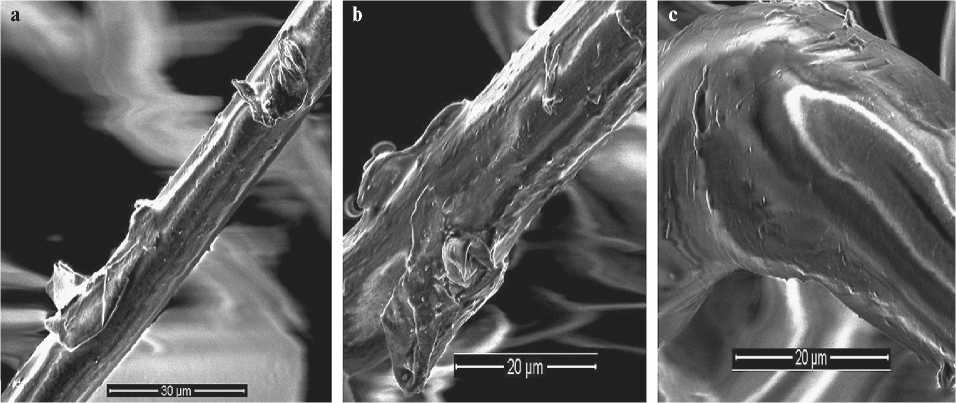
Fig. 5. Micrographs of the fibers surface of composite materials with a impregnation degree of 0,18 (a), 0,27 (b) and 0,48 (c)

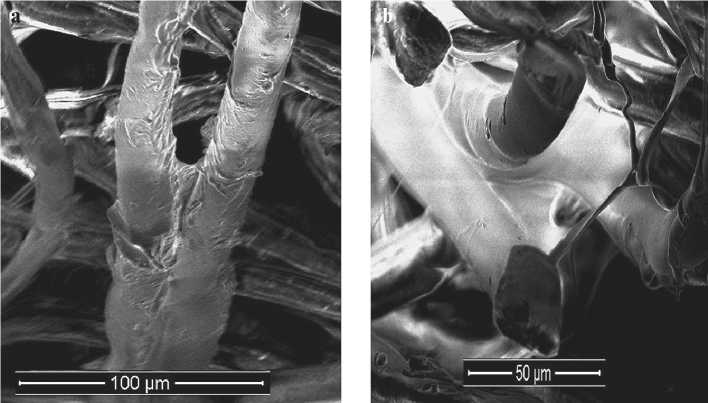
Fig. 6. Micrographs of the fiber surface of composite materials with an impregnation degree of 0,87 (a) and 1,16 (b)

TECHNOLOGY OF PRODUCTION OF BUILDING MATERIALS AND PRODUCTS

At the impregnation degree of 0.87, bridges connect adjacent fibers (Fig. 6a), and at the impregnation degree of 1.16, several fibers are connected (Fig. 6b).

CONCLUSION

– Сomposite materials with compressibility sufficient for practical use in road and hydraulic engineering construction equal to 0.5 are obtained at the impregnation degree with water dispersion of polyurethane of a non-woven needle-punched fabric made

of polyethylene terephthalate fibers with a diameter of 20–25 microns;

Сomposite materials with an impregnation degree of 0.5 are recommended for use in road and hydraulic engineering construction at a pressure of less than 36 kPa, at which the material compressibility degree is not more than 0.2;

Сomposite materials compressibility decreases when connecting fibers with bridges of polymer particles, whereas the polymer coating formation on the fibers surface practically does not affect compressibility.