Градиентная теория упругости для моделирования парапротезного перелома на поверхности кость-имплантат
Автор: Гитман И.М.
Журнал: Российский журнал биомеханики @journal-biomech
Статья в выпуске: 4 (50) т.14, 2010 года.
Бесплатный доступ
В данной работе рассматривается поверхность между кортикальной костной тканью и имплантатом, в частности, её состояние в случае парапротезного перелома, т.е. перелома кости в области вокруг протеза, замещающего сустав. Для моделирования концентрации напряжений в области перелома кортикальной кости использовалась модель теории упругости с анизотропным градиентом (теория упругости высокого порядка с дополнительными константами, учитывающими влияние микроструктуры в различных направлениях). Для учёта микроструктурных эффектов в кортикальной костной ткани были введены в рассмотрение два параметра линейного размера на макроскопическом уровне: первый параметр относится к характерному размеру системы трансверсальных Гаверсовых каналов (остеонов), другой - к продольному размеру волокон. Материал имплантата считался изотропным, а масштаб длины соответствовал характерному размеру материала. Были решены численные тестовые задачи для анализа напряжений в области вершины трещины. Показано, что градиент упругости (анизотропный для кости и изотропный для металла) не даёт сингулярных решений и учитывает неявное влияние микроструктуры. Кроме того, было изучено влияние масштабного эффекта в области кость-имплантат.
Анизотропный градиент упругости, перелом кости, концентратор напряжений в кости, поверхность на границе кость-имплантат, масштабный эффект
Короткий адрес: https://sciup.org/146216004
IDR: 146216004 | УДК: 531/534:
Текст научной статьи Градиентная теория упругости для моделирования парапротезного перелома на поверхности кость-имплантат
В настоящее время непрерывное увеличение переломов шейки бедра у пожилых людей является значительной проблемой. В некоторых случаях при данных переломах рекомендуется полностью удалить головку и шейку бедра, заменив их имплантатом. Тем не менее тотальная артропластика шейки бедра может быть также связана с серьёзными осложнениями, одним из которых являются парапротезные переломы, т.е. переломы в соседних с протезом областях. К сожалению, количество данных осложнений при полном замещении шейки бедра протезом возрастает [22]. Большинство парапротезных переломов в области шейки бедра возникает вследствие относительно небольших травм (падений или поворотных смещений имплантата), при которых появляются концентраторы напряжений, стимулирующие развитие повреждения.
С точки зрения механики, данная проблема может быть рассмотрена как анализ взаимодействия двух тел из разных материалов (костной ткани и протеза) с целью
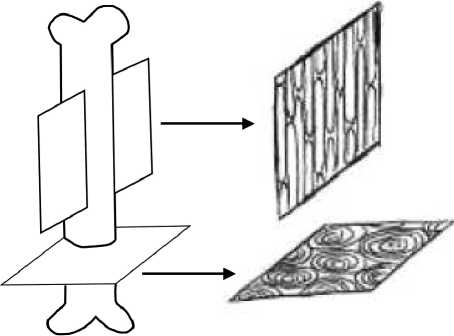
Рис. 1. Анизотропия кости нахождения упругих напряжений в области вершины трещины перпендикулярной поверхности контакта. К сожалению, классическая механика сплошной среды не позволяет реалистично описать этот феномен, что отражается в появлении сингулярных решений в области вершины трещины. Различные модели [6, 9, 10, 13] применяются для того, чтобы избежать появления нежелательных и нереалистичных решений.
Другой важной причиной, по которой применение классической теории упругости неприемлемо при решении данной задачи, является неспособность теории учитывать как сложную анизотропную структуру костного материала, так и изотропию металла, из которого изготовлен имплантат; однако эта информация крайне необходима.
Ещё одним недостатком классической теории упругости является её неспособность учёта влияния масштабного эффекта, который, как известно, имеет место.
Прокомментируем сначала влияние микроструктуры материала. Кости, как и большинство биологических тканей, являются деформируемыми телами со сложной внутренней микроструктурой (рис. 1). Минерализованная костная ткань является одним из структурных компонентов кости, благодаря которому кость обладает ячеистой (в виде пчелиных сот) трёхмерной микроструктурой. Микроструктура кости сильно влияет на её механический отклик, например в случае концентрации напряжений в области переломов. Адекватное описание поля напряжений около трещины в кости является необходимым для анализа развития перелома в дальнейшем. С другой стороны, титан (материал, который наиболее часто используемый при изготовлении имплантатов) также обладает уникальной сложной микроструктурой [23].
При решении данной проблемы необходима модель, которая не даёт сингулярных решений в области вершины трещины, учитывает микроструктуру и масштабный эффект, отличная от модели классической упругости, которая не может это учесть. Другими словами, необходим многоуровневый подход. Среди большого числа различных многоуровневых подходов можно выделить прямой метод, затратный в смысле большого числа вычислений и основанный на методе конечных элементов [15, 18]. В данной работе было предложено использовать другую методологию, основанную на добавлении дополнительного слагаемого к соотношению классической упругости (градиентную теорию упругости) [1, 3, 5, 6, 8, 21]. Модели градиентной теории упругости реалистично описывают механическое поведение благодаря добавлению дополнительного градиентного слагаемого, связанного с параметром линейного размера, который содержит информацию о структуре материала на более «мелких» уровнях.
В разделе 2 будут кратко описаны элементы градиентной теории упругости. В разделе 3 эта теория будет обобщена для случая анизотропии. Раздел 4 будет посвящен численному анализу парапротезного перелома на поверхности кость–имплантат. Масштабный эффект будет рассмотрен в разделе 5. Завершит статью обсуждение результатов в разделе «Заключение».
Градиентная теория упругости
Несколько формулировок градиентной теории упругости было предложено в литературе. Айфантис ( Aifantis ) и др. сформулировали определяющее соотношение, в котором напряжения зависят от деформаций и второго градиента деформаций [1, 3, 21]. Другая формулировка теории упругости градиентного типа была предложена ранее Эрингеном ( Eringen ) на основе нелокальной упругой формулировки интегрального типа [3, 12]. В этой модели напряжения и вторые градиенты напряжений зависят от деформаций; похожие соотношения рассматривались в работе [7]. Изучению связи между моделями Айфантиса и Эрингена посвящены работы [4, 17].
Различие между двумя вышеупомянутыми моделями заключается во введении дополнительного материального параметра внутреннего масштаба длины, который учитывает микроструктуру материала.
В данной статье будет рассмотрена модель Айфантиса. Градиентная теория упругости в случае изотропии может быть представлена в следующем виде:
ij ijkl kl kl,mm , где oij - напряжения, зависящие от деформаций £kl и второго градиента деформаций, Dijkl – тензор упругих коэффициентов четвертого ранга, l – параметр внутренней длины, связанный с размерами микроструктурных неоднородностей. Стоит отметить, чтобы перейти от (1) к классическому соотношению теории упругости, необходимо положить l , равное нулю. Уравнение (1), как и в классической теории упругости, решается совместно с уравнениями равновесия:
о j b= 0, (2)
где bi – массовые силы. Связь между деформациями и перемещениями записывается в виде классических геометрических соотношений Коши:
£ kl = 2 ( uk , l + u l , k ) ' (3)
Анизотропная градиентная теория упругости
Проблема становится гораздо сложнее, если один из рассматриваемых материалов (в данном случае костная ткань) теряет изотропию [17]. Влияние анизотропии, с одной стороны, может выражаться в эффективных упругих константах (модуль Юнга, коэффициент Пуассона), с другой стороны, необходимо учитывать больше чем один параметр линейного масштаба. Нахождение параметра линейного размера само по себе является проблемой. Некоторые попытки связать его с представительным объёмным элементом микроструктуры были предприняты в работе [16]. Рассмотрение существования представительного объёмного элемента в кости и определение его размеров являются предметом дальнейших исследований. В данной работе вместо этого определяется связь параметров линейного масштаба с характерными размерами микроструктурных компонентов кости.
Анизотропия костной ткани обусловлена её необычной архитектурой: в продольном направлении она имеет волокнистую структуру, в поперечном (трансверсальном) располагается система Гаверсовых каналов (остеонов). Необходимо учесть, что в данной работе не рассматривается трабекулярная костная ткань (хотя это очень важно); она будет рассмотрена в будущих исследованиях.
С учётом трансверсальной изотропии кости вводятся в рассмотрение два параметра линейных размеров lF и lH, связанные с особым расположением волокон и ортогональным направлением систем Гаверсовых каналов [17]. Теперь (1) может быть записано в виде g = - L (4)
ij ijkl kl mn kl,nm , где коэффициент трансверсальной изотропии находится из соотношения
L = 1 2 n n + 1 2 ( 8 - n n ), (5)
mn F m n H mn m n , где n – направление анизотропии. При специальном условии lF = lH = l можно снова перейти к изотропной градиентной теории упругости.
Следует рассмотреть подробнее производные высоких порядков от перемещений u i в уравнении равновесия в случае изотропных и анизотропных материалов. Производные четвертого порядка требуют использования частных C 1-непрерывных функций формы, которые должны быть непрерывными во всей области, как и перемещения и их первые производные. Это довольно трудно реализовать, используя метод конечных элементов, и в качестве альтернативы используются некоторые формулировки градиентной теории упругости, которые избегают этих строгих ограничений [7, 21].
Представленный Ру ( Ru ) и Айфантисом ( Aifantis ) подход преодолевает вышеупомянутые трудности. Этот метод будет использоваться в данной статье. Уравнение (4) может быть записано в следующей форме:
D . U ,j + b i = 0, (6)
O
g
-
L™n
^
g
,
m
n
=
D
i
j
k1
U
где верхний индекс с соответствует упругим «классическим» слагаемым, g – градиентным слагаемым.
Взаимодействие на поверхности кость–ИМПЛАНТАТ
Следующий уровень сложности – решение задачи о взаимодействии анизотропной костной ткани с изотропным титановым имплантатом, а именно учёт поверхности взаимодействия.
Разность между величинами различных параметров линейных масштабов материалов кости и металла на различных уровнях также должна быть учтена: остеоны (или системы Гаверсовых каналов) могут рассматриваться как цилиндрические структуры шириной 200 мкм и длиной несколько миллиметров [11]; характерный размер титана 20 мкм [23].
Геометрия задачи взаимодействия кость–имплантат в численном примере представлена на рис. 2. К образцу приложена номинальная вертикальная нормальная деформация 1%. В соответствии со стандартным подходом, а также вследствие
|
F кости |
имплантата |
|
F кости |
L
L симметрии моделируется только верхняя половина. При расчётах длина образца L = 2 мм.
Параметры титана были выбраны следующими: модуль упругости титана Е Т = 110 ГПа, коэффициент Пуассона (ν = 0,3). Материал считался изотропным, и параметр линейного масштаба был равен 0,2 мм. Экспериментальные исследования
[19, 20] позволяют использовать модули Юнга для кости в случае трансверсальной изотропии Е F = 20,7 ГПа и Е H = 11,7 ГПа, коэффициенты Пуассона ν НF = 0,23 и ν FH = 0,42. Как было упомянуто выше, учитывая связь между параметром линейного масштаба и характерным размером микроструктурных компонентов кости, параметры линейных масштабов были выбраны соответственно l F = 2 мм и l H = 0,2 мм.
Чтобы исследовать появление сингулярных решений, была решена задача методом конечных элементов и показана сходимость решений. На рис. 3 представлены результаты, полученные на сетках 8 x 8 (штрих - пунктирная линия), 16 х 16 (точечная линия), 32 x 32 (пунктирная линия), 64 x 64 (сплошная линия) четырехсторонних квадратных элементов.
Как видно из рис. 3, при сгущении сетки сходимость решений становится очень хорошей. Из рисунка также видно, что кривые зависимостей компонентов напряжений в области вершины трещины гладкие и конечные, это подтверждает способность предложенного метода избежать сингулярных решений в области вершины трещины.
Изучение влияния масштабного эффекта
В данном разделе будет показана возможность учёта масштабного эффекта с помощью анизотропной градиентной модели для сложной геометрии в проблеме взаимодействия кость–имплантат.
Масштабный эффект для учёта поведения костных тканей известен со времен Галилео Галилея, который впервые упомянул о нём в работе [14]: «Каждому известно, что лошадь, упавшая с высоты трёх или четырёх локтей (старинная линейная мера длины, равная примерно 45 см, т.е. длине локтевой части руки человека), сломает свои кости, в то время как собака или кошка, упавшие с высоты восьми или десяти локтей, не пострадают. Таким же «безвредным» может быть падение кузнечика с башни или падение муравья с высоты, равной расстоянию до Луны. Почему для детей падение с высоты обходится без последствий, в то время как для пожилого человека это может обернуться травмой ноги или, возможно, черепа? Почему чем меньше животные, тем они пропорционально крепче и сильнее, и также меньшие растения способны выдерживать больше, чем большие деревья. Я уверен, что дуб высотой двести локтей ( braccia (ит.) – локоть) не мог бы выдержать свои ветви, если бы они располагались как у дерева с обычными размерами; природа не может создать лошадь размерами в двадцать раз больше обычных лошадей или гиганта в десять раз выше обычного человека, за исключением чудес или сильно изменённых пропорций его конечностей и особенно его костей, которые должны быть значительно больше обычных. Современное мнение, что искусственные механизмы, очень большие и очень маленькие, одинаково прочны и долговечны, является ошибкой. Например, маленький обелиск, или столб, или другое твёрдое тело могут непременно быть составлены или установлены, и они будут прочными, в то время как большее тело разлетится на кусочки при малейшем воздействии, что исключительно зависит только от их собственного веса».
Хорошо изучен тот факт, что классическая теория упругости не способна корректно описать масштабный эффект, т.е. механические отклики материала, зависящие от размера. Также было показано, что в качестве альтернативы может использоваться градиентная теория упругости, которая учитывает масштабные эффекты [2, 4, 8]. В данной статье представлена анизотропная градиентная модель, которая будет протестирована в более сложной задаче исследования напряженного состояния поверхности при взаимодействии двух материалов. На рис. 4 показана зависимость логарифма отношения напряжений (σ nom /σ tip ), где σ nom – номинальное напряжение, σ tip – напряжение в области вершины трещины, от логарифма отношения
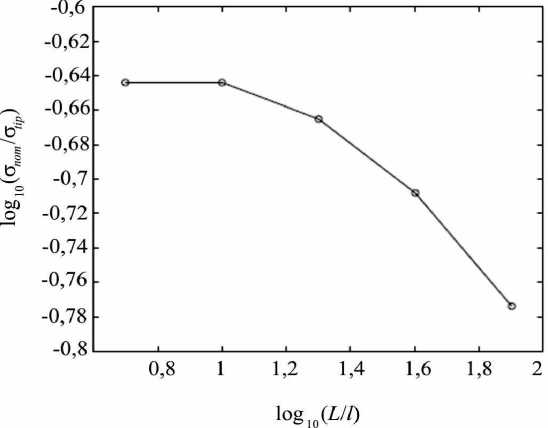
Рис. 4. Изучение масштабного эффекта характерной длины кости к масштабу длины в трансверсальном направлении, т.е. к характерному диаметру остеонов (L/l). Выбор нормализации линейного масштаба был обусловлен рекомендациями, полученными в работе [17], где было сделано заключение о предпочтительном выборе линейного масштаба, который должен совпадать с направлением существующей трещины. В данном случае перелом возникает в трансверсальном направлении, и, таким образом, в качестве доминантного масштаба был выбран диаметр остеонов.
На рис. 4 представлены полученные результаты. Для меньших размеров образца линейный масштаб по величине будет того же порядка, что и эффекты, которые строго отражаются на поведении всего образца. Можно увидеть, что в левой части кривой есть «плоский» участок с коэффициентом наклона, равным 1. В случае увеличения размера образца более гладкая зона остаётся прежней; однако она будет гораздо меньше размера всей геометрической области, и, таким образом, в образце будут возникать концентраторы напряжений в области вершины трещины. Этот эффект можно заметить в правой части кривой с коэффициентом наклона, строго отличающимся от 1 и становящимся круче с увеличением размера.
Заключение
В данной работе на примере взаимодействия на границе поверхности кость– имплантат были рассмотрены парапротезные переломы. Трещина возникает в более мягком материале (в данном случае в костной ткани) и распространяется в направлении к более жесткому материалу (в данном случае к титану). Для того чтобы избежать появления сингулярных решений, возникающих в области вершины трещины, была предложена градиентная модель вместо использования классического определяющего соотношения теории упругости. Также было проведено изучение влияния плотности разбиения области на конечные элементы с целью показать отсутствие сингулярных решений. Для класса анизотропных материалов была использована адаптированная упругая градиентная модель Айфантиса. Было показано, что данная модель способна учесть масштабный эффект при анализе взаимодействия кость–имплантат.
Список литературы Градиентная теория упругости для моделирования парапротезного перелома на поверхности кость-имплантат
- Aifantis E.C. On the role of gradients in the localization of deformation and fracture//International Journal of Engineering Science. -1992. -Vol. 30. -P. 1279-1299.
- Aifantis E.C. Strain gradient interpretation of size effects//Int. J. Fract. -1999. -Vol. 95. -P. 299-314.
- Altan B.S., Aifantis E.C. On some aspects in the special theory of gradient elasticity//Journal of Mechanical Behavior of Materials. -1997. -Vol. 8. -P. 231-282.
- Askes H., Aifantis E.C. Numerical modeling of size effects with gradient elasticity -formulation, meshless discretization and examples//Int. J. Fract. -2002. -Vol. 117. -P. 347-358.
- Askes H., Gitman I.M. Higher-order continua derived from discrete media: continualisation aspects and boundary conditions//International Journal of Solids and Structures. -2005. -Vol. 42. -P. 187-202.
- Askes H., Gitman I.M. Non-singular stresses in gradient elasticity at bi-material interface with transverse crack//International Journal of Fracture. -2009. -Vol. 156. -P. 217-222.
- Askes H., Guti´errez M.A. Implicit gradient elasticity//International Journal for Numerical Methods in Engineering. -2006. -Vol. 67. -P. 400-416.
- Askes H., Morata I., Aifantis E.C. Finite element analysis with staggered gradient elasticity//Computers and Structures. -2008. -Vol. 86. -P. 1266-1279.
- Barut A., Guven I., Madenci E. Analysis of singular stress fields at junctions of multiple dissimilar materials under mechanical and thermal loading//International Journal of Solids and Structures. -2001. -Vol. 38. -P. 9077-9109.
- Carpinteri A., Paggi M. Analytical study of the singularities arising at multi-material interfaces in 2D linear elastic problems//Engineering Fracture Mechanics. -2007. -Vol. 74. -P. 59-74.
- Currey J.D. Bones: structure and mechanics. -Princeton University Press, 2006.
- Eringen A.C. On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves//J. Appl. Phys. -1983. -Vol. 54. -P. 4703-4710.
- Fenner D.N. Stress singularities in composite materials with an arbitrarily oriented crack meeting an interface//International Journal of Fracture. -1976. -Vol. 12. -P. 705-721.
- Galilei G. Dialogues сoncerning two new sciences//Translated from the Italian and Latin into English by Henry Crew and Alfonso de Salvio. -New York: Macmillan, 1914.
- Geers M.G.D., Kouznetsova V.G., Brekelmans W.A.M. Multi-scale computational homogenization: trends and challenges//Journal of Computational and Applied Mathematics. -2010. -Vol. 234. -P. 2175-2182.
- Gitman I.M., Askes H., Aifantis E.C. The representative volume size in static and dynamic micro-macro transitions//International Journal of Fracture. -2005. -Vol. 135. -P. 3-9.
- Gitman I.M., Askes H., Kuhl E., Aifantis E.C. Stress concentrations in fractured compact bone simulated with a special class of anisotropic gradient elasticity//International Journal of Solids and Structures. -2010. -Vol. 47. -P. 1099-1107.
- Gitman I.M., Askes H., Sluys L.J. Coupled-volume multi-scale modelling of quasi-brittle material//European Journal of Mechanics A -Solids. -2008. -Vol. 27. -P. 302-327.
- Hoffmeister B.K., Smith S.R., Handley S.M., Rho J.Y. Anisotropy of Young's modulus of human tibial cortical bone//Medical and Biological Engineering and Computing. -2000. -Vol. 38. -P. 333-338.
- Rho J.Y. An ultrasonic method for measuring the elastic properties of human tibial cortical and cancellous bone//Ultrasonics. -1996. -Vol. 34. -P. 777-783.
- Ru C.Q., Aifantis E.C. A simple approach to solve boundary-value problems in gradient elasticity//Acta Mechanica. -1993. -Vol. 101. -P. 59-68.
- Sarvilinna R. Risk factors of periprosthetic hip fracture. -University of Tampere, 2005.
- Stolyarov V.V., Beigelzimer Ya.E., Orlov D.V., Valiev R.Z. Refinement of microstructure and mechanical properties of titanium processed by twist extrusion and subsequent rolling//The Physics of Metals and Metallography. -2005. -Vol. 99. -P. 204-211.


