Probabilistic solutions to the problem of rational consumer choice with random income
Автор: Timofeeva G.A., Ie O.N.
Рубрика: Математическое моделирование
Статья в выпуске: 2 т.14, 2021 года.
Бесплатный доступ
Probabilistic solutions are used when the amount of decision-makers is large. Each of them chooses the optimal solution independently of the others by solving his optimization problem. In this case, the optimal solution constructed by a randomly selected person (e.g. a consumer of goods) can be considered as a random vector. In particular, probabilistic solutions arise naturally in the rational consumer choice problem if income is assumed to be random. The problem of the utility function maximization at a time when the income of a randomly selected consumer is described as a random variable is considered as the stochastic optimization problem. The properties and distribution of the probabilistic solution of the consumer choice problem for various types of the utility function and income distribution are studied.
Stochastic optimization, probabilistic solution, the problem of consumer choice, random income, utility function
Короткий адрес: https://sciup.org/147235237
IDR: 147235237 | УДК: 519.8 | DOI: 10.14529/mmp210202
Текст научной статьи Probabilistic solutions to the problem of rational consumer choice with random income
In the study of stochastic optimization problems, for example, problems of finding the maximum of a function that depends on a random parameter, one of the following criteria is usually used: optimization of the expected value of the objective function, maximization of the probability of reaching a certain level of the objective function (probability criterion), optimization of the fixed probability quantile. A bicriterial approach can also be used to maximize the average value and minimize the variance. In all these approaches, it is assumed that the solution to the problem is taken once and is deterministic. On the other hand, there exists a significant number of problems in which the decision is made many times, by many persons, independently of each other. In these cases, it makes sense to consider stochastic optimization problems with a random solution [1, 2]. In addition, the probabilistic solution of optimization problems naturally arises in simulation systems, when for each value of a random input a (possibly auxiliary) optimization problem is solved. The study of probabilistic solutions to optimization problems with random parameters was started in [3]. The probabilistic solutions to the problem of optimizing a function depending on a random parameter were analysed in [4]. In this article the rational consumer choice problem for a randomly selected consumer is investigated as an optimization problem with a random restriction, probabilistic properties of solutions to the optimization problem with random restrictions are studied.
-
1. Definition and Properties of a Probabilistic Solution
Let us consider the general probabilistic optimization problem that contains random variables in both the objective function and restrictions
minJ( x ’^)’
*X ( £ )
G.A. Timofeeva, O.N. Ie where the function f (x, b) : Rn x Rm ^ R1 is continuous in the aggregate of variables, the mapping X (b) : Rm ^ C(Rn) is continuous compact-valued, £ = £(ш) is a random vector defined on probability space (Q, F, P) with values in Rm.
Together with probabilistic optimization problem (1), we consider the parametric optimization problem
min f (x,b). xEX (b) V 7
The set of solutions to problem (2) is denoted by
X * (b) = Arg min { f (x, b) | x G X(b) } .
Definition 1. A random compact set X * (£) = X * (£(ш)) is called the probabilistic solution to stochastic programming problem (1) .
In general case, X * (£) is a random compact set . The definition and properties of random sets were considered in [5]. If problem (2) has a unique solution for any b G B then X * (£) is a random vector . Conditions of the existence of a probabilistic solution to stochastic optimization problem (1) follow from properties of the parametrical optimization problem (2).
The following Berge Maximum Theorem (see e.g. [6]) describes the conditions for the existence and continuity of a solution to the optimization problem with parameters.
-
Theorem 1. Let f : X x B ^ R be a continuous function and ф : B ^ X be a compact valued and continuous correspondence, where X C R n and B C R m . Then the maximum value function
V(b) — max f (x, b) хЕф ( Ь )
is well-defined and continuous and the optimal policy correspondence
x*(b) — {x G ф(b)|f (x,b) = V(b)} is nonempty, compact valued and upper hemicontinuous.
The following statement follows from Berge Maximum Theorem and properties of convex functions.
Lemma 1. Let the function f (x, b) be strictly concave in x and continuous in the aggregate of variables on X x B , where X C R n is a convex compact set, B C R m is an open connected set. Then the solution x* to problem (2) exists and is unique for all b G B, and the function X * = Ф(Ь) is a continuous vector function of the parameter b.
-
Theorem 2. If the conditions of Lemma 1 hold and £(ш) is a random vector taking values from the set B then X * (ш) is a random vector.
-
2. Problem of Rational Consumer Behavior with Random Income
Indeed, let b = £ (ш) be a random vector. The existence and uniqueness of the solution to (2) implies that the set X * (£) is defined and consists of one point with probability 1. The continuity of the function X * ( • ) implies its measurability, thus X * (£) is a random vector.
If the conditions of Theorem 1 are not satisfied, then the probabilistic solution to optimization problem (1) can be a random set [7].
МАТЕМАТИЧЕСКОЕ МОДЕЛИРОВАНИЕ
Consider the properties of probabilistic solutions to the problem of consumer choice with a random income (CCRI). A theoretical study of properties of the consumer choice problem in the case of a fixed income is given in [9]. The CCRI problem is formulated as an maximization problem with a random restriction max f (x), xexШ V ’ (3)
X(b(<)) = { x G R n : P T x < b(<), x > 0 } .
Here f (x) is the utility function of goods (UF), x = { x 1 ,...,x n } is the demand vector for various goods, p = { p 1 ,...,p n } is the price vector for the corresponding goods, the parameter b(^ ) is the income of a randomly selected consumer.
Currently, there are various approaches to choosing the best approximating income distribution function. Beta and gamma distribution, Pearson distribution, various exponential functions, etc. are used as such approximations [10]. The most common hypothesis among economists is that income distribution is subject to the logarithmically normal law [11]. In particular, the log-normal law is used to describe the structure of population incomes by official statistical services of Russia, including the Federal State Statistics Service of the Russian Federation. Another, less common theory, is the application of the Pareto distribution law to approximate income [12]. The work [13] proposes a technique for using the combined Pareto-lognormal distribution function as an approximation of the distribution of incomes of the population of Russia. The proposed approach assumes the use of the lognormal distribution function for the low- and middleincome segment, and the Pareto function for the high-income segment, since the power-law dependence better approximates the ”right tail” of the distribution.
Let us study the properties of the solution to problem (3).
Denote by X * (£) the probabilistic solution to problem (3). Assume that the utility function f (x) satisfies standard assumptions and is neoclassical [9], that is
-
• f (x) is continuous and twice differentiable on the space of goods R ^ = { x G R n : x i > 0, i = 1,..., n } ;
-
• all first-order partial derivatives f X i (x) are positive inside R ^ ;
-
• the matrix of the second-order derivatives of the function f (x) (Hosse matrix) is negative definite inside R ^ .
Examples of neoclassical functions are as follows [9, 14]:
-
1) UF Cobb-Douglas
n
f(x) = A П x a i , 0 < a i < 1, i = 1,...,n, A> 0, i =1
-
2) logarithmic UF
n f (x) = 53 ai M1 + bixi), ai > 0, bi > 0, i = 1,..., n, i=1
G.A. Timofeeva, O.N. Ie
-
3) gradual additive UF
n f (x) = 52 aixei, 0 < ei < 1, xi > 0, ai > 0, i = 1,..., n, i=1
-
4) quadratic UF
n
f ( X ) = ^
i =1
1 n n
a i X i + ^EEb i j x i x j , where
i =1 j =1
n
a j + ^2 b ij x i > 0, i =1
j = 1,.. .,n.
If the function f (x) is neoclassical, then a solution to problem (3) exists and is unique for any fixed b > 0 and any set of positive prices p [9].
Stone–Geary utility function [14] has the form
n f (x) = H(xi - ai)a, ai > 0, 0 < ai < 1, i = 1,...,n, i=1
and satisfies the neoclassical conditions only for x G R a = { x G R n : x i > a i , i = 1,..., n } .
The goods distribution is calculated based on the solution X(b(£)) of the optimal choice problem (3). It is natural to expect that the distribution of goods is proportional to the average expected income. But this is true only in the case of a linear dependence of the solution X(b) to the optimal choice problem on the income b .
Let us consider examples of linear and nonlinear dependence of the distribution of goods on income.
-
Example 1. Let us take the CCRI problem with Stone–Geary utility function
n max Ц (xi — ai)ai,
i =1
X (b(£)) = { x G R n : x i > a i , i = 1,...,n, p T x < 6(£) } .
Here a i > 0 is the minimum necessary amount of the i -th good that is acquired by a consumer and is not a matter of choice; the numbers a i > 0 characterize the value of the i -th good for a consumer.
A solution to problem (4) is unique for any set of positive prices p and any fixed b > B a , where n
B a = 52 a i P i .
i =1
The optimal solution to problem (4) is written in the form:
x * (b) = a + (b — B a )c,
where a = { a 1 ,..., a n } , the coordinates of the vector c are determined by the relations
n ci = 5' A = E ai. (6)
p i A i =1
The logarithmically normal law is the most often considered as the distribution of income [11], but problem (4) has no solutions in the case of low incomes, i.e. for b G (0; B a ) . Therefore, an another law is considered as the income distribution. In [12] it is proposed
МАТЕМАТИЧЕСКОЕ МОДЕЛИРОВАНИЕ to apply the Pareto distribution law to approximate income. It is assumed that b(£) is a Pareto distributed random variable with the distribution density
( kb k m
f (x) = < x k +1 ’ x — b m , [ 0, X < b m ,
where b m — B a , k > 1.
The probabilistic solution X * (£) is a random vector and its distribution is determined by the joint distribution density. It follows from equalities (5) – (6) that the functions x * = Ф i (b) are linear in the parameter b . Therefore, the distribution densities f ф i (x) of the functions x * = ф i (b) = a i + c i (b — B a ) of a continuous random variable b(^ ) can be written.
The mean values of the probabilistic solution to CCRI problem are
E ( x * (<)) = a i + c i (b — B a ), b = E <Ж)) = 7—m, i =1,...,n, (8)
k — 1
where E is the mathematical expectation.
In the case of k > 2 , the second moments of the obtained probabilistic distributions are 2
Cov ( x * (() ,x * (()) = c i c j ^ b , a b = (T%, ij = 1,...,n. (9) k — 1 k — 2
We can also calculate the required quantity of goods of each type to meet the demand with a given probability γ , i.e. the distribution quantile:
q i (Y) : P{q : X * (() < q i } = Y-
From (5) we have that
qi (y ) = ai + (1y — Ba)ci, i = 1,...,n, where lγ is a quantile of the same level γ for income distribution. In the case of Pareto distribution, the quantile lγ is determined by the probability level and the distribution parameters bm and k:
b m
k1—~'
Thus, in this case, the distribution of the solution (the optimal choice of goods) x * (£) may be calculated according to the density of income distribution, and the distribution of consumption of various types goods is obtained on this base.
Example 2. Let us consider the CCRI problem with the power additive utility function [14] as an example with a nonlinear dependence of the solution to the consumer choice problem on income. In this case, the solution to the optimization problem is not calculated analytically. Therefore, the simulation modelling is used to calculate the distribution of consumption.
n f (x) = S aixei ^ max,
i =1
X = { x G R n : p T x < b(^), x — 0 } .
Here e i G (0; 1) , a i > 0 for all i .
G.A. Timofeeva, O.N. Ie
Let the income of a randomly selected consumer b(£) be a logarithmically normally distributed random variable with the distribution density ft (x) = —1= exp (-(Xnx—M).) .
ax \2n \ 2a2/
Although the solution to the optimal choice problem is not analytically found for an arbitrary b , it can be written as
Xi* = zi (y*^’ zi = (p/) , ci = 1 —в, where y* = g (b) is a positive root of the equation
n
G(y) = b, G(y) = ^ (УT Pizi• i=1
Since c i > 0 , p i > 0 , z i > 0 for all i = 1, • • •, n , then G(z) is a monotonically increasing function, which is continuous on [0, + ^ ) and
G(0) = 0, lim G(z) = + ^ .
Therefore, the positive root of equation (15) exists for all b > 0 and the function y = g(b) is monotonically increasing.
From formula (14), the mathematical expectation of the consumers optimal choice is found as follows:
E (x * (b(( ))) = Z i E (g c (b(( ))•
The obtained dependence is nonlinear, therefore simulation modelling should be used to find this mathematical expectation. Since the function g(b) is monotonically increasing and ci > 0, zi > 0, then it is enough to know a quantile of the population income distribution to find the consumption distribution quantile. The following relations are obtained qi (Y ) = ziQc (Y), (16)
where Q ( y ) is the root of equation (15) for b = l Y , i.e. Q = g(l Y ) , l Y is the quantile of the income distribution. In the case of the lognormal distribution, the quantile is determined by the probability level and the distribution parameters µ and σ :
l Y = exp(^ + t y a), (17)
where τ γ is the quantile of the level γ of the standard normal distribution.
Thus, the quantile of the distribution of goods can be calculated without using simulation and it is possible to study analytically the distribution of consumers choice.
Suppose that the utility function has the form f (x) = 12x0,2 + 5x0,4 + 7x3,x + 10x4,3,
X = { x G R 4 : 4x i + 7x 2 + 5x 3 + 12x 4 < b(^), x > 0 } -
МАТЕМАТИЧЕСКОЕ МОДЕЛИРОВАНИЕ
The optimal solution for different values of the distribution parameters µ and σ was found by numerical methods using simulation in Wolfram Mathematica. From the obtained distribution of the probabilistic solution to the problem on the optimal choice of X * (£) find the mathematical expectations of the components of the random vector
X = E (X * (()).
The following results are obtained. For lognormal distribution of income with ^ = 2 , a = 0,3 the mathematical expectation of the random vector is X(£) = (0, 765; 0, 207; 0,156; 0, 212). Fractions characterizing the components of the vector are equal x = (0, 571; 0,155; 0,116; 0,158) (Fig. 1).
For ^ = 7 , a = 0, 8 the mathematical expectation of the random vector is X(£) = (72, 023; 95, 727; 8, 700; 39, 351). Fractions characterizing the components of the vector are equal x = (0, 334; 0,444; 0, 040; 0,182) (Fig. 2).
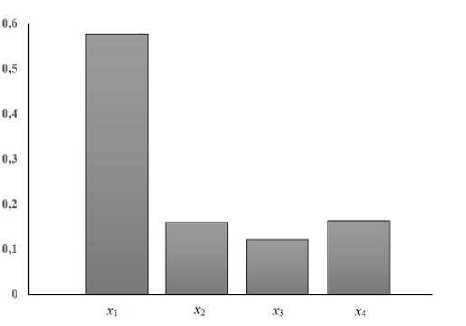
Fig. 1 . ^ = 2, a = 0, 3
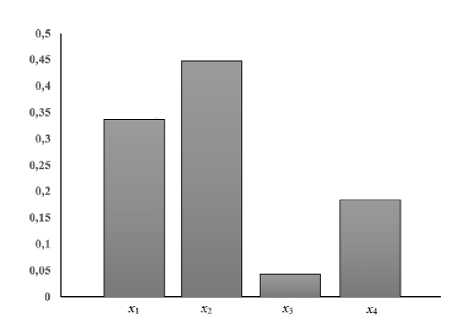
Fig. 2 . д = 7, a = 0, 8
From the considered example, it follows that the study of the demand functions and the income distribution b(£) allows to calculate the distribution of demand for goods, and, according to the distribution of X * (£) , can restore the average expected needs for benefits.
Conclusions
The distributions of the probabilistic solution to the stochastic optimization problem are quite diverse in the case of a nonlinear objective function. The distribution of the probabilistic solution to the rational choice problem with a random restriction is investigated.
Список литературы Probabilistic solutions to the problem of rational consumer choice with random income
- Timofeeva, G. Analysis of Transport Network Development via Probabilistic Modelling / G. Timofeeva, A. Martynenko // Stability and Oscillations of Nonlinear Control Systems. -V. 14. - P. 1-2.
- Timofeeva, G. Investigation of Mathematical Model of Passenger Preferences / G. Timofeeva // Application of Mathematics in Engineering and Economics. - 2019. - V. 2172. -Article ID: 080001. - 7 p.
- Popova, O.A. Optimization Problems with Random Data / O.A. Popova // Journal of Siberian Federal University. Mathematics and Physics. - 2013. - V. 6, № 4. - P. 506-515.
- Тимофеева, Г.А. Вероятностные решения задач условной оптимизации / Г.А. Тимофеева // Труды института математики и механики УрО РАН. - 2020. - Т. 26, № 1. - С. 198-211.
- Matheron, G. Random Sets and Integral Geometry / G. Matheron. - New York: Wiley, 1975.
- Aliprantis, D. Infinite Dimensional Analysis: a Hitchhikers Guide / D. Aliprantis, K. Border. - Berlin: Springer, 2007.
- Тимофеева, Г.А. Свойства вероятностных решений задач условной оптимизации со случайными параметрами / Г.А. Тимофеева, О.Н. Ие // Устойчивость и колебания нелинейных систем управления. - 2020. - V. 22. - C. 413-416.
- Поляк, Б.Т. Введение в оптимизацию / Б.Т. Поляк. - М.: Наука, 1983.
- Varian, H.R. Intermediate. Microeconomics. A Modern Approach / H.R. Varian. - New York: University of California at Berkeley, 2009.
- McDonald, J. Some Generalized Functions for the Size Distribution of Income / J. McDonald // Econometrica. - 1984. - V. 52, № 3. - P. 647-663.
- Chotikapanich, D. Global and Regional Inequality in the Distribution of Income: Estimation with Limited / D. Chotikapanich, M.R. Valenzuela, D.S. Rao // Incomplete Data. Empirical Economics. - 1997. - V. 20. - P. 533-546.
- Arnold, B. Pareto Distributions: Pareto and Related Heavy-Tailed Distributions / B. Arnold. - Riverside, University of California at Riverside, 1980.
- Бутаева, K.O. К вопросу о распределении денежных доходов населения России / K.O. Бутаева // Уровень жизни населения регионов России. - 2016. - № 2 (200). - C. 130-136.
- Singh, N. The Potluck Problem With Consumers Choice Behavior / N. Singh, S. Rao. -2009. - P. 328-333.


