The impact of geographical factors on expanding trade interactions between countries (on the example of the Asia-Pacific region)

Over the past three decades, the global economy has been growing due to the expansion of international trade and economic interactions, including on the basis of integration processes. In this regard, the influence of geographical factors on trade relations is an important indicator in studying the dynamics of intensification of interactions between national economies.

Gravitational modeling is highlighted as a theoretically substantiated method to conduct quantitative analysis of the influence of geographical factors on trade interactions (Yotov, 2022); this method is the most common tool for assessing the impact of various factors on trade, including physical distance and land borders1. Nevertheless, one of the lingering methodological problems of gravitational models has been the “distance puzzle”, which manifested itself in the high negative impact of physical distance on trading even after taking into account many factors and controlling various effects (Lin, Sim, 2012).

We should note that over the past decade and a half, significant progress has been made in the methodology of gravitational modeling, allowing for a more accurate assessment of the impact of geographical factors on trade between and within national economies. In the framework of a modern methodological approach that takes into account the deviation of trade in favor of home markets

(Yotov, 2012; Baier et al., 2018), the scientific problem of the “distance puzzle”, which existed for a long time (Bosquet, Boulhol, 2015; Buch et al., 2004), has been successfully solved, thus allowing us to obtain correct estimates of the influence of physical distances on trade. This circumstance is an important aspect in assessing the impact of geographical factors on trade, in particular the comparative costs that exporters and importers spend on overcoming the spatial distance between markets. Along with solving the above methodological problem, spatial remoteness can differentially affect the exports of various firms (Chaney, 2014); therefore, there is also the task of adequately representing physical distances to assess trade flows between countries. This ultimately leads to the need for the correct selection of this parameter to obtain appropriate quantitative estimates (Head, Mayer, 2010).

Solving the “distance puzzle” within the framework of gravitational modeling also contributed to obtaining a consistent assessment of the impact of “border area” on trade between countries (Borchert, Yotov, 2017). Despite the fact that a large share of traded goods in the global economy is transported by sea and transoceanic transport, there are a number of countries for which commodity exchange through checkpoints2 on the land border is an important component for expanding their cooperative ties with foreign markets. In addition, if integration ties between countries expand, their border territories receive an impetus in economic development through the creation and operation of joint trade and production infrastructure (Starr, Thomas, 2002). On the other hand, the presence of unresolved conflicts (Carter, Poast, 2017), noticeable differences in socio-economic development between countries (Hassner, Wittenberg, 2015) become insurmountable barriers to increasing mutual trade, leveling the natural advantages of “border area” or the factor of having a joint land border. Therefore, the presence of land borders between countries can either promote or hamper mutual trade.

Despite methodological progress in the assessment of gravity models, the impact of geographical factors on trade in general remains controversial. The available empirical ex-post assessments of the impact of geographical factors on trade within the global economy, on the one hand, indicated a reduction in the negative impact of physical distance, as well as the positive impact of land border on trade (Yotov, 2012; Borchert, Yotov, 2017); on the other hand, they indicated the cases of constant (time-invariant) influence of geographical factors on trade due to a proportional increase in trade and reduction of transport costs (Buch et al., 2004).

We should note that over the past three decades, the Asia-Pacific region (APR) has become one of the most dynamically developing economies in the world, accounting for more than half of global GDP, and its share of intraregional trade accounted for about 40% of global trade turnover3. By this indicator, the APR surpasses any other subglobal region. The estimates obtained (Izotov, 2020a; Izotov, 2023) revealed a noticeable increase in trade turnover in the APR in connection with integration processes, i.e. due to the implementation of WTO mechanisms and preferential trade agreements.

The expansion of trade interactions between the APR countries became possible due to the manifestation of economies of scale in trade between the economies in terms of leveling some tariff and nontariff barriers, as well as reducing the cost of moving products between countries, indirectly indicating the positive impact of geographical factors on trade in the subglobal region, due to the dominance of relatively cheap sea transportation4 and creating appropriate infrastructure to expand trade between border countries in the absence of active military and political confrontations in the region.

At the same time, accumulated empirical estimates of the influence of geographical factors on trade in the APR show rather contradictory results, primarily due to methodological problems associated with the presence of the “distance puzzle” (Filippini, Molini, 2003; Yu et al., 2014). The importance of assessing the impact of geographical factors on trade turnover in the APR lies in the fact that these factors are among the key elements in studying the dynamics of economic connectivity of a subglobal region, in favor of which Russia has been striving to intensify its foreign trade in recent years. The above allows us to formulate the following hypotheses: first, the APR has been facing a decrease in the negative impact of physical distance on trade in the long term; second, it is assumed that over the past three decades there has been a positive impact of the presence of a land border on trade turnover in the subglobal region.

Thus, the aim of the study is to assess the impact of geographical factors on trade in the APR. According to the research algorithm, the following tasks are addressed: 1) analyzing the dynamics of trade interactions in the APR, assessing the values of physical distances between trading economies of the subglobal region; 2) selecting an assessment methodology and forming a data set; 3) assessing the impact of physical distances, land borders and other factors on trade in the APR. The assessment is carried out for a long-term period covering 1993– 2021. As in the previous study (Izotov, 2023), the APR includes the countries and economic territories of East Asia5, Australia and Oceania6, as well as the countries of the Pacific coast of North, Central and South America7.

Trade interactions and spatial remoteness within the APR

The value of trade within the APR has increased significantly: from 2.2 trillion US dollars in 1993 to 16.7 trillion US dollars in 2021. During the period under consideration, the share of trade between the Asia-Pacific countries amounted to more than 70% of their total trade turnover, indicating a high degree of connectivity between the economies of the subglobal region. With the exception of some episodes related to global and subglobal crises89, there was a noticeable trend of long-term expansion of trade interactions in the APR (Fig. 1).

Figure 1. Trade turnover within the APR: value and physical characteristics

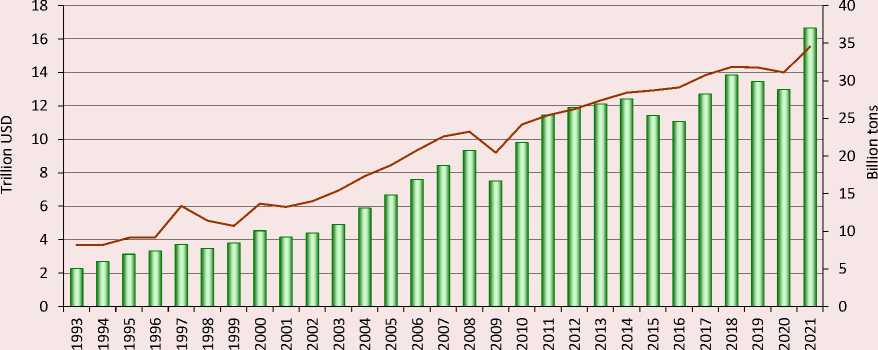
I I Value (trillion USD)            -------Physical volume (billion tons)

Note: we calculated the data on the physical volume of trade turnover for 2002–2021 on the basis of UNCTAD physical volume indices for exports and imports and statistics provided by the World Bank, RIETI, CEPII, CEIC9.

Sources: UNCTAD, World Bank, RIETI, CEPII, CEIC, own calculations.

One of the points characterizing the expansion of mutual trade is the increase in the physical volume of trade in the APR from at least 8.2 billion tons in 1993 to 34.5 billion tons in 2021. An estimate of the value of one ton of goods traded in the APR in 2021 prices indicated its reduction from 523 US dollars in 1993 to 482 US dollars in 2021. This circumstance suggests that the restraining influence of spatial remoteness on trade flows has weakened in the APR over the period under consideration. This assumption needs to be confirmed by assessing the impact of physical distances on trade in the APR.

To obtain such an estimate, it is important to choose the values of physical distances between countries, which is a separate research task due to the lack of reliable aggregated statistics based on real transport routes for this indicator. One of the widely used ways to estimate physical distances between trading countries is to calculate this indicator based on their coordinates on the Earth’s surface. In this case, the basic value s of physical distances between countries are calculated as follows:

dkl = 6381 x arccos x ([sin (Y) x sin (Yj)] + + [cos (Y) x cos (Y) x cos (Xt - Xj)]), where dkl — physical distance between agglomerations k and l of countries i and j, respectively (in kilometers), X – longitude in degrees, Y – latitude in degrees10. The values dkl are reflected in the CEPII database; they are based on the calculation of the shortest distances between two points on the Earth’s surface11, which use the geographical coordinates of the largest agglomerations of trading partner countries.

Physical distances within the countries were calculated as follows (Head, Mayer, 2010): du = 2/3 x ^ area/и, where di. — physical distance within country i; area – area of the country; π – pi value. Applying this technique to estimate physical distances within countries is based on the theoretical assumption (Head, Mayer, 2010) that in the economic space production is significant in a central location, and consumers are distributed at even distances from it, which greatly simplifies finding the values of this parameter and is a widespread way to estimate dii in gravitational modeling (Baier et al. al., 2018; Yotov, 2022).

Using basic values of physical distances to determine transport costs is controversial (Head, Mayer, 2010) due to the fact that the size of the markets of trading economies is not taken into consideration, which is important for constructing a gravity dependence. For this reason, to assess the impact of spatial remoteness on trade, effective values of physical distances can be used, obtained by adjusting the basic values of this indicator for the population of trading economies and bringing it to an arithmetic mean or harmonic mean value12: (l.ket(P0Pk/P0Pi) x Ziet(poPz/popj) x d^^, where popk — population of metropolitan area k in country i; popt — population of country i; popt — population of metropolitan area l in countryj; popj — population of country j; p — elasticity of trade flows between countries to the physical distance between them. In the case of ρ = 1, the effective distance is expressed as an arithmetic mean (Anderson, van Wincoop, 2003); if ρ = -1, – harmonic mean (Head, Mayer, 2010), respectively. The obtained values of physical distances within the countries and economic territories of the APR are also adjusted for the corresponding parameters of the elasticity of trade flows to distance.

The values of physical distances between the APR countries, calculated by the above methods (basic, arithmetic mean and harmonic mean), differ from each other. These discrepancies may affect the assessment of the impact of physical distance on trade in the APR. To visually reflect the correspondence between the values of bilateral trade and the physical distance between the trading countries of the subglobal region, we constructed a diagram (Fig. 2) reflecting the structure of trade turnover in the APR depending on the intervals of values of physical distances. The allocation of physical distance intervals is used for the purpose of distributing trade flows between countries of the subglobal region: between geographically close countries (0–375 km – high level of proximity; 375–750 km – average level of proximity; 750– 1,500 km – low level of proximity); between geographically remote countries (1,500–3,000 km – low level of remoteness; 3,000–6,000 km – average level of remoteness; more than 6,000 km – high level of remoteness). Physical distance intervals were constructed on the basis of previously conducted empirical studies (Eaton, Kortum, 2002;

Head, Mayer, 2013) with the following exception: to analyze the change in the comparative share of trans-Pacific trade, the distance interval exceeding 6 thousand km was decomposed into two intervals: from 6 to 12 thousand km; from 12 to 20 thousand km.

The analysis showed that, regardless of the considered values of physical distances, the distribution of trade turnover in the APR by 2021, compared to 1993, has changed due to an increase in trade in the range from 0.75 to 6 thousand km, i.e. between economies located within the western and eastern parts of the APR, respectively. At the same time, the share of trans-Pacific trade in intraregional trade decreased during the period under consideration, indicating a clear fragmentation of the Asia-Pacific economic space, in which the countries and economic territories of East Asia and, noticeably lagging behind them, the countries of the North American Free Trade Area (USA, Canada and Mexico) began to play a leading role

Figure 2. Structure of trade turnover in the APR depending on the intervals of different values of physical distances

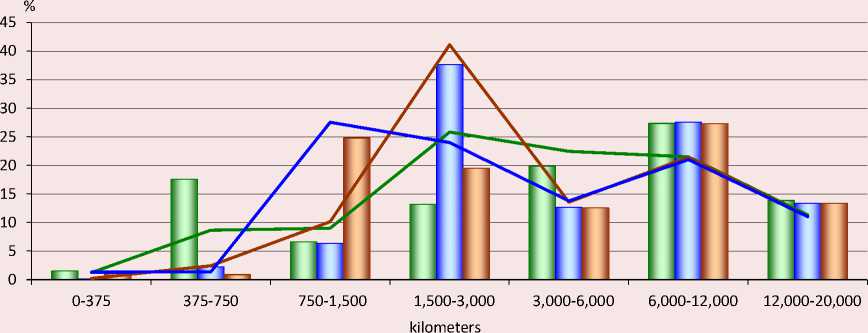
■    ■ Basic (1993)                                               Arithmetic mean (1993)

■    ■ Harmonic mean (1993)                              ^^^^^^^е Basic (2021)

^^^^^^^^n Arithmetic mean (2021)                                 ■ ^^^^^^^^^^^ Harmonic mean (2021)

Note: the trade turnover structure in the APR is presented according to the following values of physical distances: basic; arithmetic mean and harmonic mean effective distances.

Source: own calculation based on statistics from UNCTAD, World Bank, RIETI, CEPII, CEIC.

in intensifying trade. This circumstance indicates that, all other things being equal, in the long term, the growth of large and geographically neighboring economies contributed to a comparative increase in the concentration of trade in the APR in favor of close and capacious markets.

Regarding the array of different values of physical distances (basic, arithmetic mean and harmonic mean values) used in the study, we can point out that for the transport distance between the APR countries exceeding six thousand km, the values of trade turnover between them are similar. Moreover, the analysis of the structure of trade turnover in the APR, depending on the physical distances between the countries, confirmed the presence of discrepancies in the ratio between the values of physical distances and trade within 0–6 thousand km. Thus, as of 2021, according to the basic and arithmetic mean values of physical distance, the largest share of trade between the APR countries was recorded in the range of a low level of remoteness – 1.5–3 thousand km, and according to the harmonic mean value – in the range of 0.75–1.5 thousand km, i.e. within the low level of proximity. Accordingly, such discrepancies may affect the assessment of the impact of physical distance on trade in the APR. For this reason, in order to obtain more accurate estimates, we will use all three types of the above values of physical distances.

Assessment methodology and data

Assessment methodology. The assessment of the factors determining trade in the APR is based on the theoretical form of gravitational dependence between countries i and j, which is expressed as follows (Anderson, van Wincoop, 2003):

■< ^ ^{х : :} ' - (1)

where: Xij – cost of delivering goods from the point of departure (country) i to the destination (country) j; Ej – total expenses in j from all points of departure (countries); Yi – sale of goods from point (country) i to all destinations (countries); Y – world output; tij > 1 – factor of variable trade costs when moving goods from i to j; σ > 1 – constant elasticity of substitution between domestic and foreign goods; Pj – internal multilateral resistance reflecting the costs of consumers j if they purchased1goods on the world market, i.e. P)- = Ei (Ю   x 7; Пi — external multilateral resistance reflecting the trade costs faced by manufacturers in i if they supplied their products (tiA1-  Ei

"p") ^X ~Y

It follows from formula (1) that trade between countries i and j negatively depends on t_ij in comparison with multilateral external and internal resistance. This relationship is explained as follows: the more difficult the trade of countries i and j with other countries, the more incentives are created for mutual trade between i and j . Taking into account multilateral resistance in gravitational modeling significantly improved the quality of subsequent econometric estimates of foreign trade factors, and also led to a revision of previously obtained quantitative results (Baier et al., 2018). Therefore, taking into account external and internal multilateral resistance in gravitational modeling is one of the key procedures necessary to obtain correct estimates of the impact of factors on trade (Yotov, 2022).

The theoretical form of gravity dependence (1) includes domestic trade and domestic costs of country i, i.e. parameters Xii and tii, respectively (Yotov, 2012). It is necessary to include domestic trade and domestic costs in the model so as to obtain a correct assessment of the consequences of expanding trade interactions, taking into account the deviation of trade in favor of the domestic market, which affects barriers to international trade, i.e. the spatial distance of countries from each other (physical distance) and other fundamental factors (Yotov, 2012). To obtain a correct assessment, the comprehensive accounting of the costs of domestic trade is similar to the accounting of fixed effects for a particular country, including not only the costs associated with overcoming internal distances, but also any other barriers, e.g. the deviation of trade in favor of the domestic market (Borchert, Yotov, 2017). Under this approach, the trade costs of interaction with foreign countries for country i will be relative to its domestic costs.

Taking into account the accumulated recommendations for the assessment of modern gravity dependencies, the following algorithm is used. First, the gravity model is evaluated based on panel data (as a single panel). The panel data array, in addition to trade and physical distances between countries, includes domestic trade and domestic physical distances (Borchert, Yotov, 2017), and is also represented by time intervals (Egger et al., 2022), allowing to avoid underestimation due to the manifestation of effects delayed within a particular time interval. Second, external and internal multilateral resistance is controlled by fixed effects for the exporting/importing country, taking into account time factor (Baier et al., 2018). Third, the impact of all time-independent bilateral trade costs is controlled by fixed effects for the pairs of countries engaged in trade (Yotov, 2022). Fourth, in order to avoid the problem of heteroscedasticity, model specification errors caused by an incorrectly selected functional form, and in order to include “zero” trade flows, the gravity equation is estimated in multiplicative form by the Poisson quasimaximum likelihood estimator (Santos Silva, Tenreyro, 2006).

To address the tasks set in our study and complying with the above recommendations, the empirical form of model (1) is based on the method of solving the “distance puzzle” for gravity dependencies (Yotov, 2012; Borchert, Yotov, 2017), i.e. by including internal physical distances for trading countries in the panel. In addition to physical distances, in order to obtain correct estimates in the empirical model, it is recommended (Yotov, 2012) to include other factors fundamental to gravity dependence, reflected as dummy variables, namely: land border; language commonality; colonial past. The influence of other variables, unchanged and changing over time, is taken into account in fixed effects.

As a result, the estimated gravity dependence is based on Y. Yotov’s specification (Yotov, 2012). Since, in accordance with the objectives of the study, it is necessary to assess the dynamics of the influence of physical distance and land border on trade between the APR countries, the calculation of the effects of these parameters is carried out for each year (Borchert, Yotov, 2017), and the estimated gravity dependence is as follows:

Хц; =

/?<> + ^LANGij + p2CLNYij + £T°=21U pT liDIST^ +1 exp + IT-^^PtCNTGt^ x exp [тги + Xj,t + №j exp[Efj,t], (2)

where: X_ij – exports from country i to country j (this indicator also includes X_ii – trade within country i ); LANG_t j — dummy variable that takes a value equal to one if countries i and j have a common language, and zero if otherwise; CLNY_t j — dummy variable that takes a value equal to one if countries i and j were part of any colonial system (both as a dependent territory and a mother country) and zero if otherwise; lnD I ST_Ti - — natural logarithm of the physical distance between countries i and j for each year T (this indicator also includes ln DlST _Tu — natural logarithm of the physical distance in the framework of country i for each year T ); C NTG_Tt j — dummy variable that takes a value equal to one, for the presence of land border between countries i and j for each year T and zero in its absence; 0 0 — constant; T — year; t — time period; я . — fixed effects for the exporting country taking into account the year; % j — fixed effects for the importing country taking into account the year; ^ j — fixed effects for pairs of trading countries; e — error vector.

Data. Our study used panel data with a lag of four years (1993, 1997, 2001, 2005, 2009, 2013, 2017 and 2021). Within the framework of the dependent variable, statistics were used reflecting the value volumes of domestic trade of the Asia-Pacific economies and their trade with each other. In order to avoid incorrect estimates (Baldwin, Taglioni, 2006), trade statistics were presented in current prices, as well as in billions of US dollars to simplify calculations within the framework of the multiplicative model.

The availability of statistics on domestic trade ( X_ii ) was a key parameter for obtaining correct estimates. The study used one of the most common ways to calculate the value of domestic trade in national economies, which is to determine the difference between the value of goods produced in the national economy and exports (Bergstrand et al., 2015). The cost volumes of products produced in the economies of the APR were collected from special statistical databases (UNIDO, CEPII, CEIC, FAO (UN)), as well as statistical agencies of the countries of the subglobal region. However, for some countries and economic territories of the APR, it was not possible to determine the value of their domestic trade: Vanuatu, East Timor, Kiribati, North Korea, Marshall Islands, Nauru, New Caledonia, Palau, Samoa, Solomon Islands, Tuvalu, Wallis and Futuna, French Polynesia. Therefore, these economies were excluded from the estimated panel; this fact is not essential, since their total share in the intraregional trade turnover of the APR by 2021 did not exceed 0.1%. As a result, 36 Asia-Pacific economies were selected to form the data array: Australia, Brunei, Vietnam, Guatemala, Honduras, Hong Kong (SAR of the People’s Republic of China¹³), Indonesia, Cambodia, Canada, China, Colombia, Costa Rica, Laos, Macau (SAR of the People’s Republic of China), Malaysia, Mexico, Mongolia, Myanmar, Nicaragua, New Zealand, Panama, Papua New Guinea, Peru, Republic of Korea, Russia, El Salvador, Singapore, USA, Thailand, Taiwan, Tonga, Fiji, Philippines, Chile, Ecuador and Japan.

Statistical data on trade between the Asia-Pacific countries (Xij ) were taken from the databases of UNCTAD, World Bank, RIETI, CEPII, CEIC. In occasional cases, if export data for some Asia-Pacific countries were not available, we used “mirror statistics” of imports from their partner countries, which were reduced to FOB prices.

The colonial past (CLNY_tj) is taken into account for the period of the 19th – mid-20th century, covering the affiliation of certain modern countries and economic territories of the APR to the following empires: the Spanish Empire (Guatemala, Honduras, Colombia, Costa Rica, Mexico, Nicaragua, Panama, Peru, El Salvador, Chile, Ecuador); British Empire (Australia, Brunei, Hong Kong, Canada, Malaysia, Myanmar, New Zealand, Papua New Guinea, Singapore, Tonga, Fiji); Qing Empire (Hong Kong, China, Macau, Mongolia, Taiwan); Empire of Japan (Republic of Korea, Taiwan, Japan); French Empire (Indochina: Vietnam, Cambodia, Laos). Also, within the framework of the period under consideration, the “mother country – dominion” interaction between the United States and the Philippines was taken into account, which, according to the CEPII database, was included in the “integration field” of the British Empire. The belonging of a number of countries and economic territories of the APR to other colonial systems was not considered in the array, since some mother countries controlled either one country (while not belonging to the region geographically), or, for a short time, only part of the territories of modern countries of the subglobal region¹⁴, despite their notable attempts at active military and political participation in one or another part of the Pacific Ocean at the specified time.

In the course of the study, when forming an array of dummy variables to evaluate the LANGtj parameter, we found that linguistic communities in the APR were formed under the influence of past economic and political processes, which sometimes superimposed on each other (for example, large-scale migrations, as well as the existence of colonial systems that united various peoples within a single “language field”). In the APR as a whole, there are several dominant languages, either official or used along with official ones: Chinese, English and Spanish. Some countries of the APR are characterized by the presence of large Chinese diasporas; this fact allows them to be attributed to the “language field” of the Chinese language, the hieroglyphic script of which is common, despite the various dialects of the language and some simplification of writing that occurred in the 1960s in the PRC. In the study, Singapore and Malaysia are classified as the “language field” of the Chinese language in addition to China, Hong Kong, Macau and Taiwan as countries with a significant proportion of the Chinese diaspora in the total population. The “language field” of the English language includes those countries and economic territories of the APR, which were previously either part of the British Empire (Australia, Hong Kong, Canada, New Zealand, Singapore, USA), or were “under the care” of English-speaking countries, which allowed them to form a single language for communication (Papua New Guinea, Tonga, Fiji, Philippines). Spanish within the framework of the former Spanish possessions is the official language in all Latin American countries geographically belonging to the APR15; this fact was taken into account when forming the array of the corresponding dummy variable.

Physical distance is the key parameter in assessing the impact of geographical factors on trade. Statistics on physical distances included data on the distances between countries and economic territories of the APR (DlSTtj) and within them (DlSTit) so as to record transport costs in domestic markets. To determine the more accurate effect of this parameter on trade, three arrays of physical distances are used: basic distance values; arithmetic mean effective distance; harmonic mean effective distance.

The study took into account data on the presence of land border (CNTG tj ) between the following countries and economic territories of the APR: Brunei – Malaysia; Cambodia – Laos; Cambodia – Thailand; Cambodia – Vietnam; Canada – USA; Chile – Peru; PRC – Hong Kong; PRC – Macao; PRC – Laos; PRC – Mongolia; PRC – Myanmar; PRC – Russia; PRC – Vietnam; Colombia – Ecuador; Colombia – Panama; Colombia – Peru; Costa Rica – Nicaragua; Costa Rica – Panama; Ecuador – Peru; El Salvador – Guatemala; El Salvador – Honduras; Guatemala – Honduras; Guatemala – Mexico; Honduras – Nicaragua; Indonesia – Malaysia; Indonesia – Papua New Guinea; Laos – Myanmar; Laos – Thailand; Laos – Vietnam; Malaysia – Singapore; Malaysia – Thailand; Mexico – USA; Mongolia – Russia; Myanmar – Laos; Myanmar – Thailand.

As a result, the descriptive statistics of the data array have the following characteristics (Tab. 1) .

Table 1. Descriptive statistics of the data array we used

Variable	Mean	Standard deviation	Min.	Max.
Exports ( Xij ), billion USD	3.31	0.20	0	577.13
Domestic trade ( Xii ), billion USD	541.73	114.33	0.03	11245.77
Distance between countries ( DISTij ), km	–	–	–	–
Basic value of distance	9390.38	6014.67	60.77	19812.04
Arithmetic mean effective distance	9323.25	5987.45	60.77	19650.13
Harmonic mean effective distance	9273.27	6015.29	60.77	19649.83
Distance inside countries ( DISTii ), km	–	–	–	–
Basic value of distance	337.76	387.50	1.88	1554.24
Arithmetic mean effective distance	408.87	444.38	1.88	1853.80
Harmonic mean effective distance	78.43	69.73	0.22	305.74
Source: own calculation.

15 From the point of view of dummy variables for the Spanish-speaking countries of the Asia-Pacific region, their language commonality coincides with the fact that they were in the past within the framework of the Spanish Colony.

Table 2. Assessment results for model (2)

Variable	1	2	3
LANG	0.05	0.12	0.08
	(0.14)	(0.15)	(0.15)
CLNY	-0.13	-0.18	-0.16
	(0.09)	(0.11)	(0.11)
\nDIST	-0.56*	-0.66*	-0.61*
1993	(0.04)	(0.05)	(0.04)
\nDIST	-0.53*	-0.63*	-0.57*
1997	(0.04)	(0.05)	(0.04)
\nDIST	-0.53*	-0.62*	-0.57*
n        2001	(0.04)	(0.05)	(0.04)
\nDIST	-0.52*	-0.62*	-0.56*
2005	(0.04)	(0.05)	(0.04)
\nDIST	-0.54*	-0.63*	-0.58*
2009	(0.04)	(0.05)	(0.04)
\nDIST	-0.52*	-0.62*	-0.56*
n       2013	(0.04)	(0.05)	(0.04)
\nDIST	-0.51*	-0.61*	-0.55*
2017	(0.04)	(0.05)	(0.04)
\nDIST	-0.48*	-0.58*	-0.52*
n        2021	(0.04)	(0.05)	(0.04)
CNTG	0.83*	0.85*	0.65*
1993	(0.13)	(0.14)	(0.15)
CNTG	1.16*	1.14*	0.95*
1997	(0.13)	(0.13)	(0.13)
CNTG	1.21*	1.18*	1.00*
2001	(0.12)	(0.11)	(0.12)
CNTG	1.17*	1.15*	0.97*
2005	(0.12)	(0.11)	(0.12)
CNTG	1.07*	1.04*	0.85*
2009	(0.13)	(0.12)	(0.12)
CNTG	1.20*	1.16*	0.99*
2013	(0.12)	(0.11)	(0.11)
CNTG	1.20*	1.16*	0.99*
2017	(0.13)	(0.11)	(0.12)
CNTG	1.18*	1.13*	0.98*
2021	(0.13)	(0.12)	(0.12)
Constant	8.07*	7.42*	6.81*
	(0.72)	(0.79)	(0.79)
Pseudo log-likelihood	-9158	-9069	-9096
Pseudo R2	0.99	0.99	0.99
RESET-test (Prob > chi2)	0.04	0.05	0.02
Number of observations	10368	10368	10368
Δ ln DIST 1993-2021 , %	-14.72*	-12.73*	-13.93*
	(1.96)	(1.67)	(1.84)
&CNTG    %	73.69**	56.04**	80.41**
1993-2021 ,	(26.00)	(28.88)	(38.52)
Note: * p < 0.01; ** p < 0.05. 1–3 – different dependencies within the framework of model (2): 1 – dependence with the basic value of
physical distance; 2 – dependence with the arithmetic mean effective physical distance; 3 – dependence with the harmonic mean effective
physical distance. Robust values of standard errors are indicated in parentheses.
△ ln DIST 1993-2021 = ([In DIST2021 - In DIST 1993 ] / In DIST i...j x 100%. Д CNTG ,993—202, = Source: own calculation.		( [[g CV7'G 2O2i    1]   [g CV7'G i993	1]] /[е с«г С„,з _ 1]) ) х 100%.

Assessment results

The assessment of the coefficients of model (2) indicated a statistically significant dynamic impact of land border and physical distance on trade in the APR (for both basic and effective values; Tab. 2 ).

The calculations show that the negative impact of distance ( DIST ) on trade in the Asia-Pacific countries gradually decreased for all the dependencies obtained: from -0.56 in 1993 to -0.48 in 2021 for the basic value of physical distance (column 1 of Tab. 2); from -0.66 to -0.58 – for the arithmetic mean effective physical distance (column 2 of Tab. 2); from -0.61 to -0.52 – for the harmonic mean effective physical distance (column 3 of Tab. 2). This circumstance indicates the invariance of the choice of values of physical distances for the purpose of identifying a long-term tendency to reduce the negative impact of distance on trade in the APR and confirms the manifestation of gravitational attraction between the economies of the subglobal region in the long term.

The comparative analysis of the Ramsey test (RESET-test) criteria showed that the dependence with the arithmetic mean effective physical distance is generally the most acceptable of all the considered cases (column 2 of Tab. 2). We should note that the estimated values of the arithmetic mean effective physical distance are close to the basic values of the physical distance, confirming the great importance of maritime transportation carried out on linear transport routes16 in trading between the APR countries (Bertho et al., 2016). As a result, the costs of overcoming physical distance in the APR by 2021, compared with 1993 (ΔlnDIST1993–2021) decreased by 12.73%, and the estimates obtained confirmed the validity of the hypothesis stated, according to which the negative impact of physical distance on trade in the APR decreased in the long term. The attraction between the economies, which is increasing against the background of the reduction of various kinds of tariff and nontariff barriers due to integration processes, the growth of national economies and the increasing economies of scale of trade in the APR, has contributed to a noticeable reduction in the costs of moving goods between the countries within the subglobal region. As more and more economies were included in trade interactions in the subglobal region, commodity groups that had never been exported from one country or another in the early 1990s began to move within the APR in the 2010s.

The estimates obtained give grounds to assert that in the long term, with the expansion of commodity exchange of international corporations, the changing specialization of the economies in the subglobal region, the expansion of production and consumption of goods, there was a weakening of the deviation of trade in favor of the home market, reducing barriers to trade interactions between the APR economies. We should also note that the recent restrictions in trade interactions in connection with the COVID-19 pandemic did not affect the overall trend of reducing the deterrent effect of spatial remoteness on trade in the APR, indicating the close interdependence between the economies in the subglobal region. Besides, tough competition between maritime carriers in the APR is an important process, which, apparently, had a positive effect on reducing the cost and time of cargo delivery, contributing to the intensification of trade between the economies of the subglobal region (Hummels et al., 2009; Khuziyatov, 2010). If the domestic transportation market is not isolated by serious barriers, then competition between domestic and foreign transport companies can be carried out not only on international routes, but also on the domestic market (Novoseltsev, Kholosha, 2011).

The expansion of trade in the global economy in general and in the APR in particular is, among other things, a consequence of technological progress in transportation (Filina, 2009). Economies of scale, the introduction of innovations and competition between carriers contributed to the fact that the share of transport costs in the total value of traded goods in the APR, estimated rather approximately as the difference between imports in CIF prices and “mirror” exports in FOB prices, decreased from more than 6.0% in 1993 to 1.8% in 2021, confirming the fairness of the estimates obtained and reflected in Table 2.

Despite the fact that the bulk of trade turnover in the APR was carried out by sea, the land border had a positive impact on trade interactions in the subglobal region during the period under consideration: from 0.85 in 1993 to 1.13 in 2021 (column 2 of Tab. 2). In 2021, the presence of a land border contributed to an increase in trade turnover between the Asia-Pacific countries by 208.3% ((e ¹-¹³ — 1) x 100%) compared to the trade between the countries that do not have a common border, which was similar to the reduction of barriers in tariff equivalent by 28.1% [e ¹-¹3/(^1-0') — 1] x 100%, at a = 5 (Anderson, van Wincoop, 2003). In the long term, the positive impact of the presence of a land border increased by more than 56.0% (Δ CNTG _1993–2021), or by 74.8 percentage points (208.3% – 133.5%¹⁷) by 2021 compared to 1993. As a result, the assessments confirmed the validity of the second hypothesis – in the long term we observed a positive impact of the presence of a land border on trade turnover in the APR.

The result obtained is supported by the fact that in the period under consideration, despite the tightening of measures for border migration control by some APR countries, in general, the infrastructure of checkpoints in the border countries of the subglobal region expanded and the time for customs procedures decreased, in particular, within the North American market (Barajas et al., 2014; Olayele, 2019), as well as for the interaction of the

PRC with border countries (Izotov, 2020b). The positive impact of the presence of a land border on trade in the APR can also be explained by the absence of military and political confrontations in the region, with the exception of the Korean Peninsula (the DPRK was not included in the analyzed data panel) (Carter, Poast, 2017). Exogenous processes of a crisis nature restrained the positive impact of the presence of a land border on trade interactions between the APR countries: it happened during the 2009 global crisis due to a reduction in global trade and during the COVID-19 pandemic in 2021, when checkpoints were occasionally closed in order to ensure national security measures to localize the spread of coronavirus, especially in the case of the border with China. For this reason, the positive impact of the presence of a land border on trade between the APR countries in 2021 decreased compared to 2017.

The assessment indicates that the colonial past ( CLNY ) did not have a statistically significant impact on trade in the APR. This supports the findings of several empirical studies (Head et al., 2010; Jacks et al., 2011) according to which the processes of relatively free exchange of goods, integration at the global and regional levels, the disappearance of the formerly unified “institutional framework” characterized by some features of a closed trading bloc, lead to the gradual weakening and subsequent destruction of the close economic interactions formed in the past between the former mother country and its dominions or between former dominions within the framework of the former colonial system.

The parameter of language commonality (LANG) also had no statistically significant impact on trade interactions in the APR in the period under review18. We should note that in the modern world, language commonality as a whole has little or no effect on trade turnover between countries, since over the past three decades procedures for export-import operations have been significantly simplified and are effectively serviced by relatively small professional groups. As for the APR, trade interactions in this region are mainly carried out between the three major world economies (the United States, China and Japan), which use different official languages. In addition, the APR has economies such as Hong Kong and Singapore, which successfully perform intermediary functions between the countries of Southeast Asia, China and the United States, thereby leveling the negative effects of the lack of a common language in the largest economies of the subglobal region. In turn, the Spanish-speaking countries of the APR mainly trade with the United States and East Asian countries, rather than with each other.

Conclusion

Over the past three decades, trade between the countries and economic territories of the APR has significantly intensified due to the high level of connectivity of the economies. In 1993–2021, the growth of large and geographically close economies contributed to an increased deviation of trade in the APR in favor of relatively close and capacious markets, indicating the manifestation of fragmentation of the economic space in the subglobal region. At the same time, in general, there was a reduction in the transportation costs by an average of one ton of traded goods in the APR in real prices.

Physical distance is the key parameter in assessing the impact of geographical factors on trade in the APR. To determine the more accurate effect of this parameter on trade, several types of values were used: basic; arithmetic mean effective and harmonic mean effective values of physical distances.

The estimates obtained within the framework of the modern methodological approach to the construction of gravity models, on the one hand, confirmed the validity of the hypothesis about the long-term reduction of the negative impact on trade between the APR economies; on the other hand, the estimates proved the invariance in the choice of values of physical distances to determine the trend of the influence of geographical factors on trade in the subglobal region. In addition, based on formal criteria, it was determined that using the arithmetic mean effective physical distance as a key parameter of the gravity model is more acceptable for obtaining correct estimates of the influence of geographical factors on trade in the APR compared with other types of distance. As a result, the assessment showed that the cost of overcoming physical distance in the APR by 2021, compared with 1993, decreased by almost 13%, which is higher than the previously obtained values for the global economy as a whole (Borchert, Yotov, 2017). This confirms the high intensity of commodity exchange and the presence of dynamic characteristics of the impact of geographical factors on trade in the subglobal region.

Further, the estimates obtained confirmed the validity of the second hypothesis about the presence of a long-term positive impact of the land border on trade turnover between the Asia-Pacific countries, despite the fact that the bulk of trade in the subglobal region was carried out by sea. In 2021, the presence of a land border contributed to an increase in trade turnover between the Asia-Pacific countries by 208.3%. The positive impact of the land border has increased by more than 56% by 2021 compared to 1993, which may be explained by the development of the capacity of the border infrastructure, the absence of serious military and political confrontations in the APR. At the same time, exogenous processes of a crisis nature restrained the positive impact of the presence of a land border on trade in the APR.

As for other factors, such as the colonial past and language commonality, the assessment did not show their statistically significant impact on trade in the APR. First, in a relatively barrier-free environment for the movement of goods, as well as in conditions when relative costs of transporting goods decrease due to the dominance of gravitational attraction between countries, economies began to converge in terms of expanding trade interactions both between natural trading partners located nearby and with geographically remote economies. Thus, the colonial ties that existed in the recent past, formed in some cases on a non-economic basis, were leveled. Second, gravitational and integration processes in the APR helped to overcome the barrier such as the lack of a single language and, in general, did not contribute to the manifestation of special advantages from language commonality for expanding trade in the subglobal region, which is partly confirmed by empirical studies for longterm periods at the global economic level (Borchert, Yotov, 2017; Jacks et al., 2011).

Assessing the impact of geographical factors on trade turnover in the APR is an important aspect for studying the dynamics of economic connectivity in the subglobal region, in favor of which Russia has been striving to intensify its foreign trade over the past decade. In fact, the assessment of the influence of geographical factors on trade indicated the manifestation of long-term fundamental processes in the subglobal region associated with the intensification of trade interactions in conditions of reduced barriers and the dominance of gravitational attraction between the Asia-Pacific economies. Prerequisites are being created for the formation of a highly competitive commodity exchange in the subglobal region and its desire for effective Pareto equilibrium within one or another part of the APR in conditions of inevitable fragmentation of the economic space. Accordingly, the reduction in exports by any small economy to the APR, which includes Russia due to its modest share in intraregional trade, while reducing comparative transport costs, in most cases can be offset by supplies from any other country in the subglobal region. Since the cost of transportation of raw materials for the end user is more noticeable in comparison with products with high added value, the comparative reduction of transport costs for the transportation of such goods becomes an important element of competitive advantages for the Asia-Pacific countries specializing in the export of raw materials, which includes Russia.

Within the framework of the current severe restrictions on Russian products on foreign markets from developed countries, in the context of the general dynamics of reducing comparative transport costs in the APR, an increase in such costs can limit the geographical distribution of exports from Russia; thus, Russian exports will be focused more and more on the country’s neighboring markets, where significant barriers to the admission of these goods have not yet been created. Despite Russia’s being among the top eight countries – the largest owners of naval vessels in the world (Fan et al., 2018), which, all other things being equal, allows it to maintain close interactions with global markets for a long time, the Russian economy within the APR will increasingly be influenced by the gravitational pull of the Chinese economy due to the creation of barriers to medium and longdistance maritime transport in the Pacific region by narrowing the possibilities for transportation and insurance of supplies, in fact de-diversifying the geography of its trade in favor of the nearby markets of East Asia. On the other hand, the expansion of trade with China through land border checkpoints may soon become one of the main components in maintaining the functioning of a number of traditional sectors of the Russian economy, even despite the risks associated with the negative effects of monopsony.