Stationary solutions for the Cahn - Hilliard equation coupled with Neumann boundary conditions
Автор: Krasnyuk I.B., Taranets R.M., Chugunova M.
Рубрика: Математическое моделирование
Статья в выпуске: 2 т.9, 2016 года.
Бесплатный доступ
The structure of stationary states of the one-dimensional Cahn - Hilliard equation coupled with the Neumann boundary conditions has been studied. Here the free energy is given by a fourth order polynomial. The bifurcation diagram for existence and uniqueness of monotone solutions for this problem has been constructed. Namely, we find the length of the interval on which the solution monotonically increases or decreases and has one zero for some fixed values of physical parameters. Under the non-uniqueness we understand a possibility of existence of more than one monotone solutions for the same values of physical parameters.
The cahn - hilliard equation, neumann boundary conditions, steady states
Короткий адрес: https://sciup.org/147159372
IDR: 147159372 | DOI: 10.14529/mmp160206
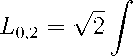
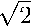
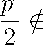
Список литературы Stationary solutions for the Cahn - Hilliard equation coupled with Neumann boundary conditions
- Cahn J.W., Hilliard J.E. Free Energy of a Nonuniform System, I. Interfacial Free Energy. The Journal of Chemical Physics, 1958, vol. 28, pp. 258-267. DOI: DOI: 10.1063/1.1744102
- Carr J., Gurtin M.E., Slemrod M. Structured Phase Transition on a Finite Interval. Archive for Rational Mechanics and Analysis, 1984, vol. 86, pp. 317-351. DOI: DOI: 10.1007/BF00280031
- Fife P.C., Penrose O. Interfaced Dynamics for Thermodinamically Consistent Phase-Field Models with Nonconcerved Order Parameter. Electronic Journal of Differential Equations, 1995, vol. 16, pp. 1-49.
- Grinfeld M., Novick-Cohen A. Counting Stationary Solutions of the Cahn -Hilliard Equation by Transversality Arguments. Proceedings of the Royal Society of Edinburgh: Section A Mathematics, 1995, vol. 125, no. 2, pp. 351-370. DOI: DOI: 10.1017/S0308210500028079
- Grinfeld M., Novick-Cohen A. The Viscous Cahn -Hilliard Equation: Morse Decomposition and Structure of the Global Attractor. Transactions of the American Mathematical Society 6, 1999, vol. 351, no. 6, pp. 2375-2406.
- Provatas N., Elder K. Phase-Field Methods in Materials Science and Engineering. Weinheim, Wiley-VCH, 2010. 312 p.
- Novick-Cohen A., Peletier L.A. Steady States of the One-Dimensional Cahn -Hilliard Equation. Proceeding of the Royal Society of Royal of Edinburg, 1993, vol. 123A, pp. 1071-1098. DOI: DOI: 10.1017/s0308210500029747
- Smoller J., Wasserman A. Global Bifurcation of Steady-State Solutions. Journal of Differential Equations, 1981, vol. 39, pp. 269-290. DOI: DOI: 10.1016/0022-0396(81)90077-2
