To numerical methods for solving multidimensional integro-differential equations

In the mathematical modeling of many processes in mechanics, physics, biology, economics, there are such systems with memory, the behavior of which depends on the entire «history» of the [1] system and is not entirely determined by the state at the moment, therefore it is necessary to describe such systems by integro-differential equations containing the corresponding integral over the time variable, i.e. when an unknown function is included in the differential expression and, at the same time, appears under the integral sign. Partial differential equations with memory are studied in

[2 -5] – when describing the thermomechanical behavior of [2 -3] polymers, viscoelastic fluids at low temperatures [4 -5] . Boundary value problems for parabolic equations with a non-local (integral) source arise when describing the mass distribution function of drops and ice particles, taking into account the microphysical processes of condensation, coagulation (combining small drops into large aggregates), crushing and freezing of drops in convective clouds [6 -9] .

From the point of view of numerical implementation, multidimensional (in terms of spatial variables) problems are considered the most complex. The difficulty lies in the significant increase in the amount of calculations that occurs when moving from one-dimensional problems to multidimensional ones. In this regard, the problem of constructing economical difference schemes that have the ability to sufficiently effectively stabilize solutions (stability) and require Q arithmetic operations proportional to the number of grid nodes, so that Q = O h p^, , where h = min h i , p is dimension of space, h i are grid steps in direction x i .

The work is devoted to the construction of a locally one-dimensional difference scheme for the numerical solution of the third initial-boundary value problem for a multidimensional differential equation in partial derivatives of parabolic type of general form with memory effect and non-local linear source, the main idea of which is to reduce the transition from layer to layer to the sequential solution of a number of one-dimensional problems in each of the coordinate directions. Moreover, although each of the intermediate problems may not approximate the original differential problem, in the aggregate and in special norms such an approximation takes place. These methods are called splitting methods, which were developed in the works of Douglas J., Peaceman D.W., Rachford H.H. [10 -11] , N.N. Yanenko [12] , A.A. Samarsky [13 -14] , G.I. Marchuk [15] , E.G. Dyakonova [16] and others.

In the works [13 -14, 17 -24] for the numerical solution of multidimensional parabolic equations, a LOS was constructed

Thus, in [13] , in an arbitrary domain G , a locally one-dimensional scheme is considered for solving linear and quasilinear parabolic equations. The stability of the difference scheme with respect to the right-hand side, boundary and initial data is proved, as well as convergence with a rate O(h 2 + т ) . In [14] , locally one-dimensional difference schemes are considered on arbitrary "nonuniform grids"for linear and quasilinear equations of parabolic type with "heat conductivity coefficient" k _a = k _a (x,t,u) depending on the "temperature" u = u ( x, t ) .These schemes converge on arbitrary nonuniform grids ω _h .

In the work [17] locally-one-dimensional difference schemes for the frac- tional diffusion equation in multidimensional domains are considered. Stability and convergence of locally one-dimensional schemes for this equation are proved. In [18] for a fractional diffusion equation with Robin boundary conditions, locally one-dimensional difference schemes are considered and their stability and convergence are proved. In the [19] locally one-dimensional difference scheme for a general parabolic equation in a p—dimensional parallelepiped is considered. To describe microphysical processes in convective clouds, non-local (nonlinear) integral sources of a special type are included in the equation under consideration. An a priori estimate for the solution of a locally one-dimensional scheme is obtained and its convergence is proved.

In the article [20 , 21] discusses the construction and study of parallel algorithms for solving the multidimensional diffusion–convection problem. Schemes of a special type are constructed - explicit-implicit difference schemes with weights, which assume the representation of the original problem as a chain of two-dimensional and one-dimensional problems.

In the work [22] the analysis of the initial-boundary value problem with a multidimensional space variable belonging to the Euclidean space R ⁿ , (n >  2) for the transport equation of a continuous medium with distributed parameters on a network-like domain is considered. An algorithm for the numerical solution of the problem under consideration is proposed.

This work is a continuation of the author’s series of works [23 -25] devoted to the study of local and nonlocal boundary value problems for multidimensional parabolic equations.

1 Problem statement

In a cylinder Qt = G x [0 <  t <  T ] , the base of which is a p - dimensional rectangular parallelepiped G = { x = (x i , X 2 ,..., X p ) : 0 <  x _a <  l _a ,a = 1, 2, ...,p } with boundary Г , G = G U Г , consider the problem

t

∂u

(x,t) G Qt ,

——+ K (x,t,T)u(x,T)dr = Lu + f (x,t), ∂t ka(x,t)~—= в-аи — ^-a(x,t), xa = 0, 0 < t < T, ∂xα

, — k a (x,t) d ^d X a = в + a U — ^ ₊ a (x,t),  x a = l a , 0 <  t <  T,

u(x, 0) = uo(x), x G G, p where Lu =   Lau, a=1

∂

L a U = я--

∂x _α

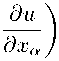
∂u

+ r a (x,t)---

∂x α

lα j pa(x, t)u(x, t)dxa, 0

0 < c o <  k a (x,t) <  c i , | r a (x,t) | , | k x a (x,t) | , К (x,t) | , | K ^{( x,} t ^,T ) | , | p^{(x,t) |} , ^{| e} ±a ^{(x,t) |}<  ^c 2 ,                       ⁽⁴⁾

u(x,t) E C4,2 (QT), ka(x,t) E C3,1 ^QT), ra(x,t), K(x,t,T), Pa(x,t), f(x,t) E C2,1 (Qt}, 0 < T < t, co,ci,c2 are positive constants, a = 1, 2,...,p, p±a(x,t) are continuous functions.

Further, we will use positive constants M i ,i = 1, 2,..., depending only on the input data of the problem under consideration

2 Locally one-dimensional scheme

We divide the interval [0, T ] into equal parts ш _т = { t j = jr, j = 0,1,..., j o } with a step t = T/j o - The interval [t j , t j +i ] is divided into p equal parts by the following points t j + a = t j + t ^ , a = 1, 2, ...,p, and we denote by A _a = (t j + a - i ,t j + a ] .

For each direction Ox _a , we construct a uniform grid with a step h _a = N a , ^a = 1,2,...,p :

p

^h J^ J^ ^ha , ^ha    {xa      ia~a, ia 0, 1, ..., Na}, a = 1, 2, ...,p,

. _ ha, ia = 1, 2,..., N a - 1, ~^a   V" :   I! \

I 2 , a    ^u, ^{1 v}a ^.

Equation (1) can be rewritten as ^2^ =1 £ _a u = 0, £ _a u =

19u p ∂t Lαu   fα ,

where fa(x, t), (a = 1, 2, ...,p) are functions that have the same smoothness p as f (x, t) and satisfy the normalization condition ^ fa = f• a=1

On each half-interval A a , a = 1, 2,...,p we will successively solve the problems

£a^(a) = 1 d^^1 - La^(a) - fa = 0, X E G, t E Aa,  a = 1, 2,...,p, p ∂t

∂ϑ ka      = в—a^(a) -

∂x α

^ —a , x a — ⁰,

k ^a 9x 2 = ^e + ^a ^ ( a )

-

Ц + a , x a    l a ,

wherein

^ (1) ^(x, ⁰⁾ = u o ^(x), ^ (1) ^(x,t j )= ^ ( p ) ^{( x,t} j ), ^j = 1, ^2,...^,j 0 ^- 1,

Using the technique of Samarsky A.A. constructing a monotone circuit [26 , p. 401], we thus obtain for each equation of number α a monotone scheme of the second order of accuracy in h _α , then we rewrite the equation with a perturbed operator L _α for a fixed α :

1 d^(a)    - „ .    .

= L a ^ ( a ^{) +} f a ,   t € A a , ^a = 1, ^{2 ,} ...,P,           (7)

p ∂t where La^(a) = XadXa (ka(x,t) ^дхО)) + ra(x,t) "a.^ -

t                                            l _α

- p J K(x,t,T N(a ) (x,T)dT - J P a (x,tyd ( a ) dx a , 00

X _a =    1g , R _a = ⁰ . ⁵ h ^{a |r a} | is the difference Reynolds number, x

^{1 +} R a             k a

( x 1 ,

x a- 1 , x a

^0.5h a , x a +1 , ..., x p ),

( - 0 . 5 a )

X = (X1,X2, ...,Xp),   Г+ = 0.5(ra + |'a|) > 0, ra = 0.5(r— - |'a|) < 0, h+ — ——  - — ——  т    ?'+   r n       (т- 0.5a) A u a — i , ua — i , ra — ra + 'a, aa — ^a lx , t j , kkα

' a = r a (x,t), P a = P a (x,t), ^ a = f a (x, t), t = t j + 1 .

Approximating on

the half-interval A_a = ^t j + a - i ,t j + a

each equation

(7) of number α implicitly we obtain p one-dimensional equations [26 , p.

401]:

y j + ppp

-

j + a — ¹ y ^p

~

~

Л — У =

-

τ

j

= Л —у

j + ^a p

^а«У х _а

x α

-^j+ p + ₽^{7 p}

,

a = 1, 2, ...,p,

I ⁺o⁽⁺¹“⁾? j ^{+ p} α a α    y x α

_    j + ^a

^{+ Ь}_а^аа У _{$ а} ^Р

N α

-

1                            As j+ a p J2 K(x,tj ,tj 0 )y(x,tj 0 + p )t - ^Payia p ~a,

j 0 =0

i a =0

j + ^a p

y x α

j + a y i a +1

-

j + ^a p

y _{i α}

j + ^a p

j + ^a p

h α

,

^y x _a

y _{i α}

-

j + ^a p

y _{i α}

h α

.

The difference analogue (6) takes the form

^a

(1 _a ) ^{j +} Р _ Q ^{j + p y} x a 0 = ^e -a ^y 0

'a

-

µ α ^,

x α

= 0,

-

( N a ) ^{j +} Pp a—  y ^ XaNNa

j + ^a = e + a y N „^P

-

У + a ,

x α

.

Let us increase the order of accuracy of the boundary conditions (9) to

O(h0), then, using the equation (1), we obtain j+ a         j+ a a       = в-^ p - Д-a + O(ha).

From the latter, by the Taylor formula, we obtain j+ a ya "   ya              г/1")-j+ Pv/

0.5ha                = x-aaa  yxa,a — e—aya j0α

- 0.5ha- X K(x,t j ,t j о ) у 0 ⁺p p j 0 =a

τ

-

N α

0.5ha ^ Р а У^~ а + Д -a , X a = 0, i a =a

j + p     j + ^{a - 1}

nrk yN a - yN a    _       ( N _a ) j ⁺ P Q   j ⁺ P

0.5ha------T------ = -X+aaa ;yxa,Na - e+ayNa - j

1           „             _x j 0 + ^a

- 0.5ha- 2 K (x,t j ,t j 0 )y _{N a} ^p T p j 0 =a

N α

- 0.5ha У2 Payi^^a + Д+a, Xa = la, ia=a where

^ _{— a} — ^Д—a ^{+ 0.5h} a ^f a, a ,   ^ + _a — Ц + a ^{+ 0 . 5 h} a f a,N _a ,  ^ ±a — ^±a⁽tj ),

^{x -a} , , a . 5 h a |r (⁰⁾ |

^{1 +}    L>6)

k _α

, r a ^a) <  0,

_      1

^{x + a} = -I , a . 5 h « |r ^{( Na )} |

^{1 +} tCNa - O.S) k _α

r a ^{N a )} >  0.

The integral over the space variable is approximated by the trapezoid formula to achieve second order accuracy.

Thus, we obtain the following difference scheme j+ a     j+ a-1

y p - y p A   j+ a , j+ p1 9

---------------- = ЛaУJ p + ^a p , a = 1, 2, ...,p, Xa G Wh„, j+a n y p - y p        j+a , _„

0.5h a-------------- = Л a y ^p + Д -a , X a = 0,

τ j+a  j+ a—

_ 0.5h ay----T----- = Л + y ^{+ p} + Д + a , X a = l a ,

y(x, 0) = U a (X),

where

^Л a У =

„ . ^{3 +} p a a y _{S a} ^P

x α

+ b + a a ⁺¹“^j

_   j + ^a

⁺ b a a a y x _a ^P

-

1 j                                N a

--EK ^{( x,t} j ,tj 0 'W.tj 0 ₊ ⁵ )^T - 52 P a У^Ъ, x a £ ^ h ,

^P j 0 =0                        ^P      i a =0

-V- a^) u'p     v^{j+ 5} _2^h a VKUtt-W 0⁺pr-

^a У = x-aaa yxa,0   e—ay0               / v K (x, tj, tj 0)y0    T p j 0=0

N α

- 0.5h a 52 P a У ^(а^ ~ а , X a = 0, i a =0

+            ( N _a ) ^{j + 5           j + 5}

^ a y = ^—X + a ^aa ’y^Na ^— P + a y^

j

-       E K (X,tj ,tj 0 )yNa+ p T - p j 0=0

N α

- 0.5h_a 52 Pay ⁽^ ~ a , X a = la, i a =0

y p

^{( a )}

—

t

j + ⁵     j + ⁵

y ^p - y ^p

τ

3 LOS approximation error

Replacing in (10) — (12) y ^{j + p} = z ^{j + p} + u ^{j + p} we get the problem for z ^{j +} 5 :

z ^{j +} p

-

τ

α - 1

Z p Л j+ 5    j + 5

------ = k a Z ^p + i a p ,

where z ^{j +} p

= У

, j⁺ 5

-

u ^{j +} p , u ^{j +} p

л j + ⁵ ,    ^{j + 5}

k aU^{3 p} + ^ a

-

_u j +^α p

-

α - 1 u j + _p

.

τ

j ⁺ 5

is the solution of problem (1) - (3) , ψ α

Denoting by -°_a = ^L_au + f _a

-

j + a

0, if P f a = f, we represent i a ^p

\ j+1 / 2                           P „

- ddU I and noticing that ^2 ia p '                            a=1

= ⁱ a ^{+ i X :}

, j + 5 i    j + 5     j + 5

i a    = k aU^{3 p} +^ a

u ^{j +} P

-

-

j + ^{5 - 1} u ^p

τ

j + ⁵

+i a — i a = y k_aU^{J p}

-

LaU j ^{+ 2}) +

j + a

⁺ I ^ a

-

f ^{j +}2 f _α

u j ⁺ 5

- u

j + ^{5 - 1 p}

-

-

τ

p

du \ ^{j +1 / 2} ∂t

)

°

°

+ i a = i a + C.

Obviously

p iX = O(ha + T), ia = О(1), E ^

j + ⁵ α ^p

p

p

E i a + E i X = och l ² + T ).

We write the boundary condition for x _a = 0 as follows:

j + ⁵     j + ^{5 - 1}

pp

0     y0____ - y0_____ _     _fl(1p)_?y^{( a )} _R (O)^-

^{0 . 5 h} a                = ^x —aaa ^y x 5 , 0    ^e —a ^y 0

1                   j 0+ p

-0.5ha- У^ K (x,tj,tj 0 )y0 PT - 0.5ha У2 Pay\a ~a + 0.5hafa,0 + Ц-a p j 0=0

Substituting yj+ p = zj+ p + uj+ p , we have j+ a j+ p pp

0 5/, z0____— zfl(ia)z(a)>)_

^{O . 5}h a                 = ^x -a a a z x p , 0

• 1                         j 0+a(a)..

• — 0.5h_a-      K(x,t j ,t j о )z 0     t — 0.5h _a У^ P _a Z i _p ~ _a +

p j 0=0

j j + ^a            1                       j 0 + ^a

+X -a aa “ ) u X _a , ₀ — e -a U ₀ ^p — 0.5ha- ^ K (x,t j ,t j о )u 0 ^P T — p j 0 =0

N “                 -j a _— u j + ^{2 - 1}

• — 0.5h _a ^X p _a u ⁽ a ) ~ _a — 0.5h _a —-------⁰-- + 0.5h _a f _a, 0 + Ц _{- а} .

0.5h _a ^ _{- a}

i a =0

To the right-hand side of the resulting expression, we add and subtract

-

= 0.5h a

∂

∂x α

l _α

∂u          ∂u

^:a я   + r a (X,t) д

∂x _α           ∂x _α

- j K(x,t,T)udT — У p _a (x,t)udx _a + f _a

-

1 du # p ∂t

j +1 / 2

.

Then, due to the boundary conditions (2) , we obtain

ψ -

α

°

= 0.5h _a ^

-

α

+ <

∗

-

α ,

ψ

∗

α

= O(h a + T ) + O(h a T ).

So, the problem for the error z ^{j +} p takes the form:

0 . 5 h a

0 . 5 h a

z ^j+ p

z ^j+ p

z j+p

-

-

j + ^{2 - 1} z ^p

τ

j + ^{2 - 1} z ^p

τ

-

j + ^{2 - 1} z ^p

τ

= л a z ^{( a )} + ^;

= A _a z ^{( a )} + ^.

, j⁺ a

α

-

,

_α , x _α

= Л + z ^{( a )} + ^ + a , X a

= 0 ,

— l a ,

z(x, 0) = 0, where

Ф а = Ф а + С, Ф а = 0(1), Ф *а = О^ а + Т ), ф- а = 0•5h а ф -а + Ф - а ,

p

Ф + а = 0.5h а ^ + а +Ф + а ,  Ф ± а = О^ а +Т ), Ф ±а = 0(1), X ф ±а = 0.

а =1

4 Stability of a locally one-dimensional scheme

Let us multiply equation (10) scalarly by у ^{( а )} = y ^{j +} p :

[ ,У ^(а^ - [ Л а У ^{( а )} ,у ^{( а )} ] = [ ф ^{( а )} ,у ^{( а )} ] ,               (14)

where [ u,v ] = P N L₀ u i a v i a ~ а , II У ^{( а ) k} L ₂ ( а ) = P a У 2 ~ а , ^а                                         i . =0

p

[ u,v = X UVH, H = Y ~а, Ну^Щ^) = X By(а)ЛL2(а)H/~а • xG^h               а=1                        ie=ia

Based on the Cauchy inequality with ε , the Cauchy-Bunyakovsky-Schwartz inequality, Lemma 1 [27] and transformations

I

N α

У ^{( а )} , X Р а У ^^^а i a =0

]  < [2, ( У ( а ) ) ²]  +[

αα

2,

/ Na           \ 2 I

I X Р а У ^{( а )} ~ а\     <

\i a =0          / J а

NN α

< 2 | y ^{( а )} | L 2 ( а ) + c 2 2 X ~ а X У 2 а ~ а <  i a =0    i a =0

• ²    N a

• < ₂ I У ^{( а )} l L ₃ ( а ) + c 2 2 X У ? . ~ а <  M 1 » y ⁽ " ⁾ « L > ( « ) , i a =0

j

• - [ у ^{( а )} , X K(x,t j ,t j 0 )y(x,t j ⁺ p ) t ] <

p j0=0                             а j

< |k X ^{K (}x,t j ,t j 0 )y^(x,t ^{j + p ) t} | |_L, Лу ^(а) 1к ( < p                                            II L 2 ( а ) II      1^2( а )

j 0 =0

j

< 2pl X Тlly(x,tj + p 1 L2- + 2llyj+p^L2(i), p j 0=0

after summing over i p = i _a , в = 1, 2, ...,P , from (14) we get

2p ( k y j ^{+ a} l^,, ) ) ,- + M-IMU . ) <  M 4 = k y X a ^p ] l L ₃ < =>h ) +

_α                   j _α

+M5(E)kyj + p k^) + M6 X kyj '+ P kWh)T+ j 0=0

⁺ 2 I k ^^ k L^h ) ⁺ X ( ^ ² a ⁺ ^ + a ) H/~ a j ,        ⁽¹⁵⁾

\                  ie = ia                        ]

where e > 0, c(e) = i ¹ + | .

Choosing e <  2 ^3- , from (15) we find

2P ( k ^{y j + a} H L ₂ (^) ) _t+

j

+ Mr k y Xa ) ] l L 2 ( ^ h ) <  M 5 ^X k y(x,t ^j ' ^{+ a} )llW + M 6 k y ^{( a )} k L 2 (_{O A} ) + j 0 =0

+ 1 ( ll^l^ ,.) + X (ЛЛ) + ^.(t ) ) h/M •    (16)

\                  ie = i a                                  j

Let us sum (16) first over α from 1 to p and then, multiplying both sides by 2т and summing over j 0 from 0 to j, we get:

j p 0α                    j p kyj+1kL2(.h) + E тX      piiL2(Oh) < M7 X тX kyj0+akL2(.h)+ j 0=0 a=1                        j 0=0 a=1

jp

X т X k^' 0+ p kL2(=h) + X (^2-a + м+a) H/~a + j 0=0 a=1 \                     ie=ia/

+ lly0kL2(^h)^ , where M7 = TM5 + Мб.

From (17), we have ky’ + 1kL2(Oh) < M7 X т X ky' '+ a kL3(i=h) + M8F' , j '=0 a=1

j p

F j = P T P   k ^ j ⁺ p 1^) + P (p -a + . 2   H/~ a + MIU.) '

j '=0 a=1 у                   ie=ia/

Let us show that the following inequality holds a                j-1

max ||yj+p Ц^) < vi V T max V'+ p H^ + Fj, - -p                    j0=0- -p where vi, v- are known positive constants.

In view of this, we rewrite the inequality (16) as

α                    α-1                     j kyj + p       < kyj + V      ) + M5T X Tkyj '+p Ill2(^) + j '=0

⁺2^TM 6 k ^{y j + p} k L ₂ ( w _h ) ⁺

+ T HIj a ll^h) + X (^2a + P+a)H/~a j •

\                     ie = ia

Summing (19) over α ⁰ from 1 to α , then we get

α                                       α j

W + p llL2(Oh) < kyj Hl2(O.) + M5 X T X Tkyj + “ к^2(^.) + a0=1 j 0=0

+T X | k^j+ ’ kl2(Oh) + X P-   + P+a0)H/~a | + a0=1 \                    ie=ia/

^α    0              ^pj

+2TM6 E kyj+Й2(С1) < kyjki2(S.) + M5 X T X Tkyj'+ a Й2(И1) + a0=1                                       a=1 j '=0

p

+2TM6 X kyj+? kL2(.h) + a=1

+ T ^X ( » _V ^{j + a} k l 2 (_O h ) + X ( p -a + m +« ) h/M .      (20)

^{a 1} у                     i e = i a                           у

Without loss of generality, we can assume that

αα

1та|р ky TkL2(^.) =ky pkL2(^.), otherwise (19) will be summed up to such a value of a that ||yj+ a |^(_^

reaches its maximum value for a fixed j . Then (20) can be rewritten as

^x p ^{k y j + p} H L^) <^{k y j} k L 2 ( _ h ) ⁺

_α                        j _α

⁺²P^TM 6 max k y ^{j + p} k L ( _ ) + pM 5 T J2 max W ⁴ p Hl₂ (__h) ^T+

Ka

p

+ T Ell ’ ^a HU. ) + X (V-a + ^ + a) H/~ a L (21) a =1 у                   i e = ia                       /

We rewrite (21) once again in the following form

_α                         j _α

(1 — 2pM6T) max ||yj+p k^h) < pM5T V max kyj'+p kL2(_h)T+ j0=0

p

+ k y ’ H L* + T El i ’ ^a k L , (_.) + E (^a + ^ + a) H/~ a | . (22) ^a =1 \                   i e = ia                       /

Choosing t < To = 4pM6, from (22), we find max kyj+ p

1

jα kL2(_h) < M9 E 1^ W + p kL2(_h)T + M10F’ , j 0=0

pα where F’ = V kL2(_h)+T P  k^ p kL2(_h) + P f^2a + ^+a) H/~a a = 1 \                    ie = ia 4             7

Based on Lemma 4 [28 , p. 171] from (23) , we get the estimate

α max ky p kL2(_h) < M9kyj kL2(_h) +

p

^{+ T}M 10 E l ^{k j} p ^k L 2 ( _ _h ) ⁺ E (^ ² a ⁺ ^ +a) ^H/ ~ a ^a =1 \                   i e = i a

.

Since it follows from (18) that kyj kL2(_h)

^j — ¹                 /a

< M 7 E ^T ^x ^{k y} ’ ⁺ p ^k L 2 ( _ h ) ^{+ M s F} ’ ^{- 1} , j 0 =0

then, from (24), we have j-i

max k ^{y j + a} |^) <  ^v 1 X ^т max k y j 0 + ^a ||L₃( _C>h ) + ^V 2 F j . j 0 =0   "

max k y ^{j +} p ||2 x

1 2 ^{( ш к )}

, the last inequality

Introducing the notation g j +i can be rewritten as follows:

j gj+i < vi E тдк + V2Fj,                   (25)

k=i where vi, V2 are known positive constants.

Applying Lemma 4 [28, p. 171] to (25), from (18), we obtain the estimate jp ky’^l^,.) < M

k y ⁰ k L <^ + X т X b^ 0 ^{+ a} ц^ *

j 0=0 a=i jp

+ E ТЕ E ^²a (0,X 0 ,t j 0 )+ ^ + a (l a ,X 0 ,t j 0 )) H/~ a ,      (26)

j 0=0 a=1 ie=ia where M = const > 0 does not depend on ha and т, X0 = (xi, X2, ..., Xa-1, Xa+1, — , , Xp)-

Theorem 1. Let conditions (4) be satisfied, then the LOS (10) - (12) is stable with respect to the right-hand side and initial data, so estimate (26) is valid for the solution of the difference problem (10) - (12) with т <  r g .

• 5    Convergence of a locally one-dimensional scheme

The solution z ( _a ) of the problem (13) can be represented as z ( _a ) = U ( _a ) + П ( _а ) , Z ( _a ) = z j ^{+ p} , where n ( _a ) is defined by the conditions [26]

ⁿ ( a ) ^{- n} ( a- i)     ?                              , _o

------------- = ^ a , X G W h a + Y h,a , a = 1, 2,...,p,         (27)

n(x, 0) = 0,    ^ a

^

ψ _α , x _α ∈ ω h _α ,

^ -a , X a = 0,

J

Ф + a , ^x a — l a ^.

We represent the solution n in (27) as n ^{j +i} = П (_Р) = n ^j + тутК + ^J2 + ... + ^J p) = n ^j = n ^{j- i} = ... = n ⁰ = 0. For n ( _a ) we have n ( _a ) = т^1 + ^J 2 + ... + ^J a ) = О(т).

The function U (_a) is determined by the conditions

u(a)   u(a—1)    7           7    7

-----T----- = Лаи (a) + ^a, ^a = ЛаП(а) + ^a, Xa € Wh^ ,

0.5h a ^^ - _T ^{U (a-1)} = Л - U^ ) + Ka, Ka = Л -^a ) + , Xa = 0, (29)

0.5h a ^{U (a)}     '   = л + u ( a ) + Ka,     ^ = Л^ ) + , Xa = U, (30)

u(x, 0) = 0.

If there exist continuous in the closed domain QT derivatives d2 u d 4u    d3u dt2 , dx2 дхв , dx^ dt

1 7 а, в 7 p, a = в,

then Л a n ( a ) = - TЛ a (^ a +1 + ... + K) = O ( t ), Л ± n ( a ) = O ( t ) .

We estimate the solution of problem (28) - (31) with the help of (26)

^{k u} j ^{+ 1} k L 2 ( ^ h ) ⁷

jp      p

0 α

+

T X W^a p k L ₃ ( _B h ) + XX 4-a + V’+a) H/~

j 0 =0 _a =1                      a =1 i e = i a

Since n ^{j +1} = ⁰, n ( a ) = ^{O ( t} ), ^{kz j +1 k} L 2 ( ^ _h ) ⁷ k ^U j ^{+ 1} k L 2 (Cu h ) , ^then it follows from estimate (32)

Theorem 2. Let problem (1) - (3) have a unique solution u(x, t) continuous in QT and there exist derivatives also continuous in QT d2 u   d 4u    d3u dt2 , дх2дхв , d^dt'

1 7 а, в 7 p , а = в,

and conditions (4) are satisfied, then the locally one-dimensional scheme (10) - (12) converges to the solution of the differential problem (1) - (3) with the rate O( | h | ² + t ) , so that for sufficiently small t the following estimate is valid

||y ^{j +1} - u ^{j +1} k L 2 ( ^ h ) 7 M (Jh | ² +r),   0 7 T o ,    | h | ² = h^ + h 2 + ... + h p .

Таблица 1: The error in the norm || • ||L₂ (_{W hT} ) when decreasing grid size for problem (1)–(3)

h	Maximum error	CO 1	CO 2
1/10	1.937583553e-1
1/20	7.249175016e-2	1.418369799	0.876007024
1/40	1.848616435e-2	1.971370905	1.081827896
1/80	4.662492757e-3	1.987272525	1.225050757
1/160	1.172124523e-3	1.991975638	1.329794326
1/320	2.948753635e-4	1.990948648	1.409241578

Таблица 2: The error in the norm || • ||c (_{W hT} ) when decreasing grid size for problem (1)–(3)

h	Maximum error	CO 1	CO 2
1/10	6.222675505e-1
1/20	1.716194235e-1	1.858322172	0.588328911
1/40	4.432645471e-2	1.952972959	0.844748018
1/80	1.120724198e-2	1.983736692	1.024912780
1/160	2.821637800e-3	1.989826572	1.156696892
1/320	7.136216269e-4	1.983301564	1.256025397

• 6    Numerical experiment

Let us define the coefficients and boundary conditions of problem (1)– (3) so that the exact solution of the problem in the two-dimensional case is the function

u(x, t) = t ³ (x 4 + X ^ ).

Below in Tables 1-2, we present the maximum value of the error ( z = y —

• u ) and the computational order of convergence (CO) in the norms IHlL^'w^) ^{and 1} • llc(w _hT ) , ^where Me (WhT) = , ^max_ Ы, ^{when h} = h l = h 2 = V ⁷ , ^{( x} i ,tj )E ^w h _T

while the mesh size is decreasing. The error is being reduced in accordance with the order of approximation O( | h | ² + т ) .

The order of convergence is determined by the following formulas:

,           llzill       ,        llzill ln Hz2||

CO ² = “ins"'

CO1 = log ^ T--T = log2    77, h2 |Ы|         |Ы| where zi и Z2 are the errors corresponding to steps 0, 5h, h.

Conclusion

We study the third boundary value problem for a multidimensional integro-differential convection-diffusion equation with a memory effect and a nonlocal (integral) source. Problems of this kind arise in the study of natural processes, for which it is necessary to take into account the prehistory (memory, hereditary properties) of the process. From physical considerations, a nonlocal source in the integral form arises in mathematical modeling in cases where there are sources (or sinks, depending on the sign of p(x, t) ) and it is impossible to obtain information about the ongoing process using direct measurements, or when it is possible to measure only some of the averaged (integral) characteristics of the desired value. For the problem under study, a locally one-dimensional difference scheme is constructed. The main research method is the method of energy inequalities. An a priori estimate of the LOS solution is obtained, from which follow uniqueness, stability, and convergence of the solution of the difference problem to the solution of the original differential problem at a rate equal to the approximation error. Numerical experiments were carried out.